Two metal rods 1 and 2 of the same length have same temperature difference between their ends. Their thermal conductivities are K1andK2 and cross-section areas A1andA2 respectively. What is the required condition for the same rate of heat conduction in them?
To determine the required condition for the same rate of heat conduction in two metal rods with different thermal conductivities and cross-sectional areas, we can follow these steps:
1. Understand the Formula for Heat Conduction:
The rate of heat conduction (Q/t) through a material is given by Fourier's law of heat conduction:
Qt=K⋅A⋅(ΔT)L
where:
- Q is the amount of heat transferred,
- t is the time,
- K is the thermal conductivity of the material,
- A is the cross-sectional area,
- ΔT is the temperature difference across the length,
- L is the length of the rod.
2. Identify the Given Conditions:
- Both rods have the same length (L).
- Both rods have the same temperature difference (ΔT).
- The thermal conductivities are K1 and K2.
- The cross-sectional areas are A1 and A2.
3. Set Up the Equations for Both Rods:
For rod 1:
Qt=K1⋅A1⋅ΔTL
For rod 2:
Qt=K2⋅A2⋅ΔTL
4. Equate the Heat Transfer Rates:
Since we want the same rate of heat conduction in both rods, we can set the two equations equal to each other:
K1⋅A1⋅ΔTL=K2⋅A2⋅ΔTL
5. Simplify the Equation:
Since the length L and the temperature difference ΔT are the same for both rods, they can be canceled out from both sides:
K1⋅A1=K2⋅A2
6. Final Condition:
Thus, the required condition for the same rate of heat conduction in both rods is:
K1⋅A1=K2⋅A2
The rate of heat conduction (Q/t) through a material is given by Fourier's law of heat conduction:
Qt=K⋅A⋅(ΔT)L
where:
- Q is the amount of heat transferred,
- t is the time,
- K is the thermal conductivity of the material,
- A is the cross-sectional area,
- ΔT is the temperature difference across the length,
- L is the length of the rod.
- Both rods have the same length (L).
- Both rods have the same temperature difference (ΔT).
- The thermal conductivities are K1 and K2.
- The cross-sectional areas are A1 and A2.
For rod 1:
Qt=K1⋅A1⋅ΔTL
For rod 2:
Qt=K2⋅A2⋅ΔTL
Since we want the same rate of heat conduction in both rods, we can set the two equations equal to each other:
K1⋅A1⋅ΔTL=K2⋅A2⋅ΔTL
Since the length L and the temperature difference ΔT are the same for both rods, they can be canceled out from both sides:
K1⋅A1=K2⋅A2
Thus, the required condition for the same rate of heat conduction in both rods is:
K1⋅A1=K2⋅A2
Topper's Solved these Questions
- THERMAL CONDUCTIONBOOK - ICSECHAPTER - THERMAL CONDUCTIONEXERCISE - LONG ANSWER QUESTIONS
5 Videos
- THERMAL CONDUCTIONBOOK - ICSECHAPTER - THERMAL CONDUCTIONEXERCISE - SHORT ANSWER QUESTIONS
19 Videos
- THERMAL CONDUCTIONBOOK - ICSECHAPTER - THERMAL CONDUCTIONEXERCISE - SELECTED PROBLEMS (Taken from the Previous Years ISC, AISSCE, HSSCE various States. Boards Roorke Qns & NCERT text) FROM EXPERIMENT TO DETERMINE K
2 Videos
- SELF ASSESSMENT PAPER 5BOOK - ICSECHAPTER - SELF ASSESSMENT PAPER 5EXERCISE - Section-D
6 Videos
- THERMAL RADIATION BOOK - ICSECHAPTER - THERMAL RADIATION EXERCISE - SELECTED PROBLEMS (from WIEN.S DISPLACEMENT LAW)
14 Videos
Similar Questions
Two metal rods 1 and 2 of same lengths have same temperature difference between their ends. Their thermal conductivities are K1 and K2 and cross sectional areas A1 and A2 respectively. If the rate of heat conduction in 1 is four times that in 2, then
View SolutionTwo rods P and Q of equal lengths have thermal conductivities K1andK2 and cross-sectional areas A1andA2 respectively. If the temperature difference across the ends of each rod is the same, then the condition for which the rate of flow of heat through each of them will be equal, is
View SolutionTwo rods of same length and areas of cross section A1 and A2 have their ends at same temperature. If K1 and K2 are their thermal conductivities, C1 and C2 their specific heats and ρ1 and ρ2 are their densities, then the condition that rate of flow of heat is same in both the rods is
View SolutionConsider two rods of same length and different specific heats (S1 and S2), conductivities K1 and K2 and area of cross section (A1 and A2) and both having temperature T1 and T2 at their ends. If the rate of heat loss due to conduction is equal then
View SolutionSteady state temperature are shown in the diagram. Find ratio of thermal conductivity k1k2 if length of all rods are same
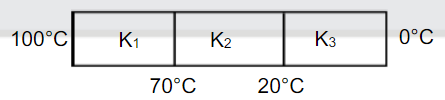
View SolutionTwo rods A and B of different materials are welded together as shown in figure. Their thermal conductivities are K1 and K2. The thermal conductivity of the composite rod will be

View SolutionCondider two rods of same length and different specific heats (s1,s2), thermal conductivities (K1,K2) and areas of cross-section (A1,A2) and both having temperatures (T1,T2) at their ends. If their rate of loss of heat due to conduction are equal, then
View SolutionTwo identical rods are made of different materials whose thermal conductivities are K1andK2. They are placed end to end between two heat reservoirs at temperatures θ1andθ2. The temperature of the junction of the rod is

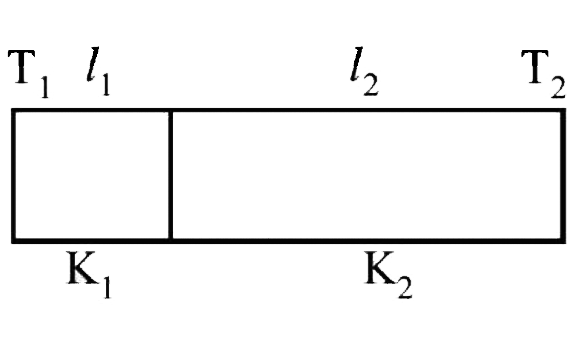
View SolutionOne end of a thermally insulated rod is kept at a temperature T1 and the other at T2. The rod is composed of two sections of length l1andl2 and thermal conductivities K1andK2 respectively. The temperature at the interface of the two section is
View SolutionThree rods A, B and C of the same length and same cross-sectional area are joined as shown. Their thermal conductivities are in the ratio 1:2:3/2. If the open ends of A and C are at 200∘C and 18∘C, respectively, then the temperature at the junction of A at steady state is
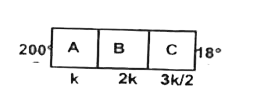
View Solution
