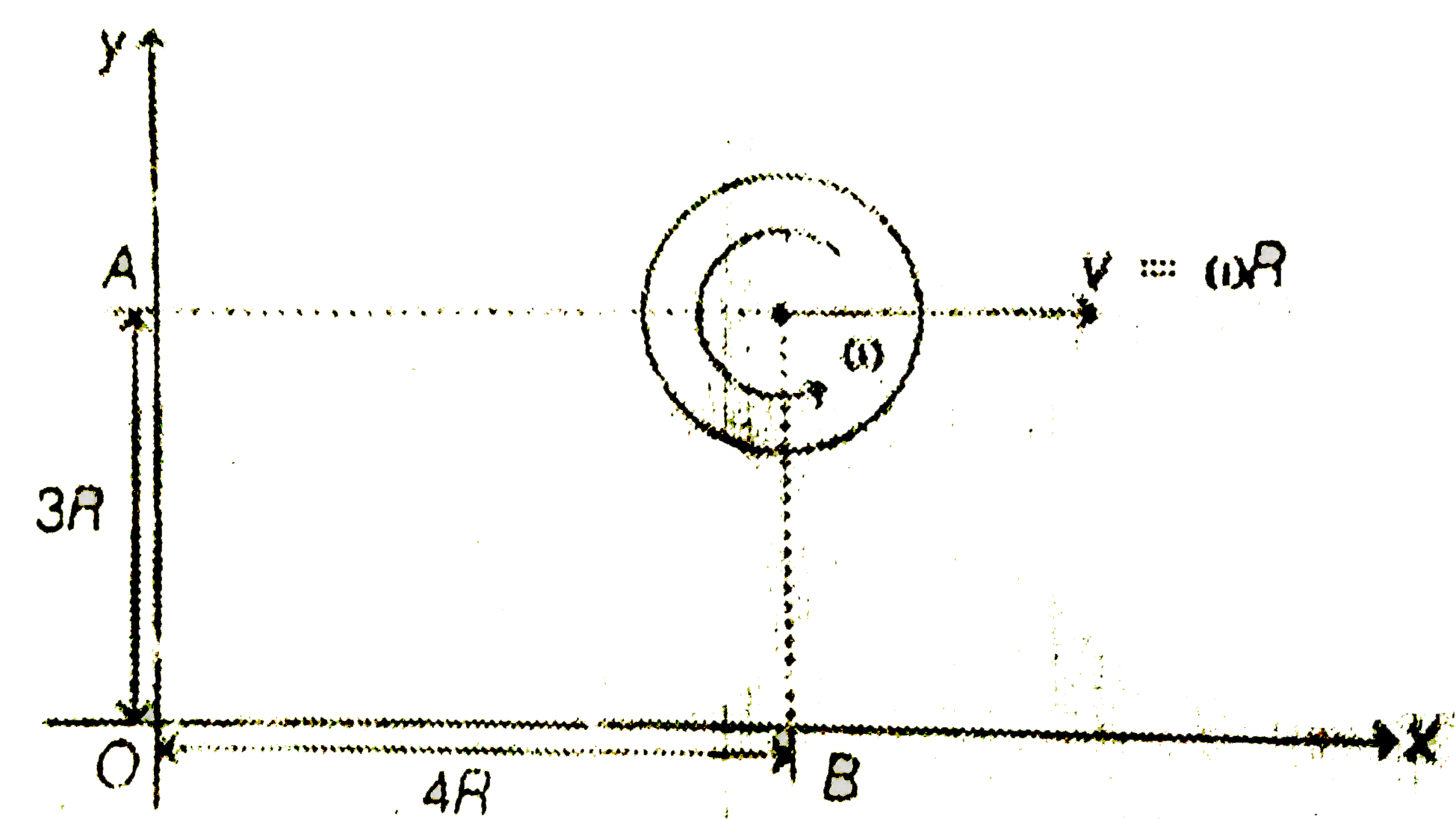
A lamina is made by removing a small disc of diameter 2 R from a bigger disc of uniform mass density and radius 2R, as shown in figure. A second similar disc is made but instead of hole a disc of triple the density as of first it filled in the hole. Centre of mass is calculated in both the cases and was found at a distance r1&r2 from centre O respectively find the ratio ∣∣∣2r2r1∣∣∣
The correct Answer is:2
r1=[−σ×πR2]×R[−σ×πR2]+[σ×π(2R)2]
&r2=[2σ×πR2]×R[2σ×πR2]+[σ×π(2R)2]⇒∣∣∣2r2r1∣∣∣=2
Similar Questions
Knowledge Check
- Question 1 - Select One
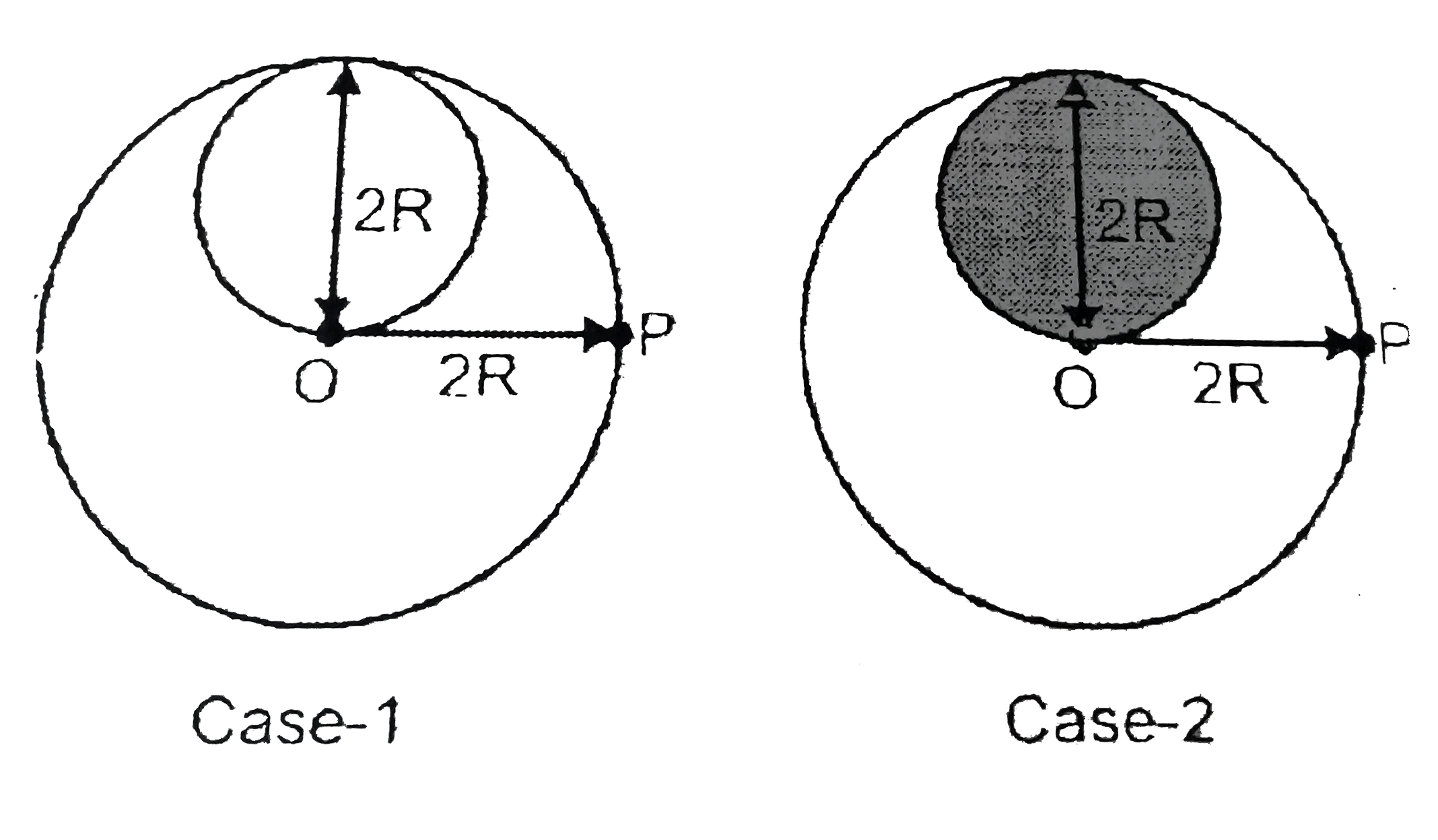
A lamina is made by removing a small disc of diameter 2R from a bigger disc of uniform mass density and radius 2R, as shown in the figure. The moment of inertia of this lamina about axes passing though O and P is IOandIP respectively. Both these axes are perpendicular to the plane of the lamina. The ratio IPIO is
 A13/37B37/13C73/31D8/13
A13/37B37/13C73/31D8/13
The centre of mass shifts by a distance S, when a square of side R2 is cut from a uniform disc of radius R as shown in figure. The value of S is
 View Solution
View SolutionA circular metallic disc of radius R has a small circular cavity of radius r as shown in figure. On heating the system
 View Solution
View SolutionFrom a uniform disc of radius R, a circular section of radius R/2 is cut out. The centre of the hole is at R/2 from the centre of the original disc. Locate the centre of mass of the resulting flat body.
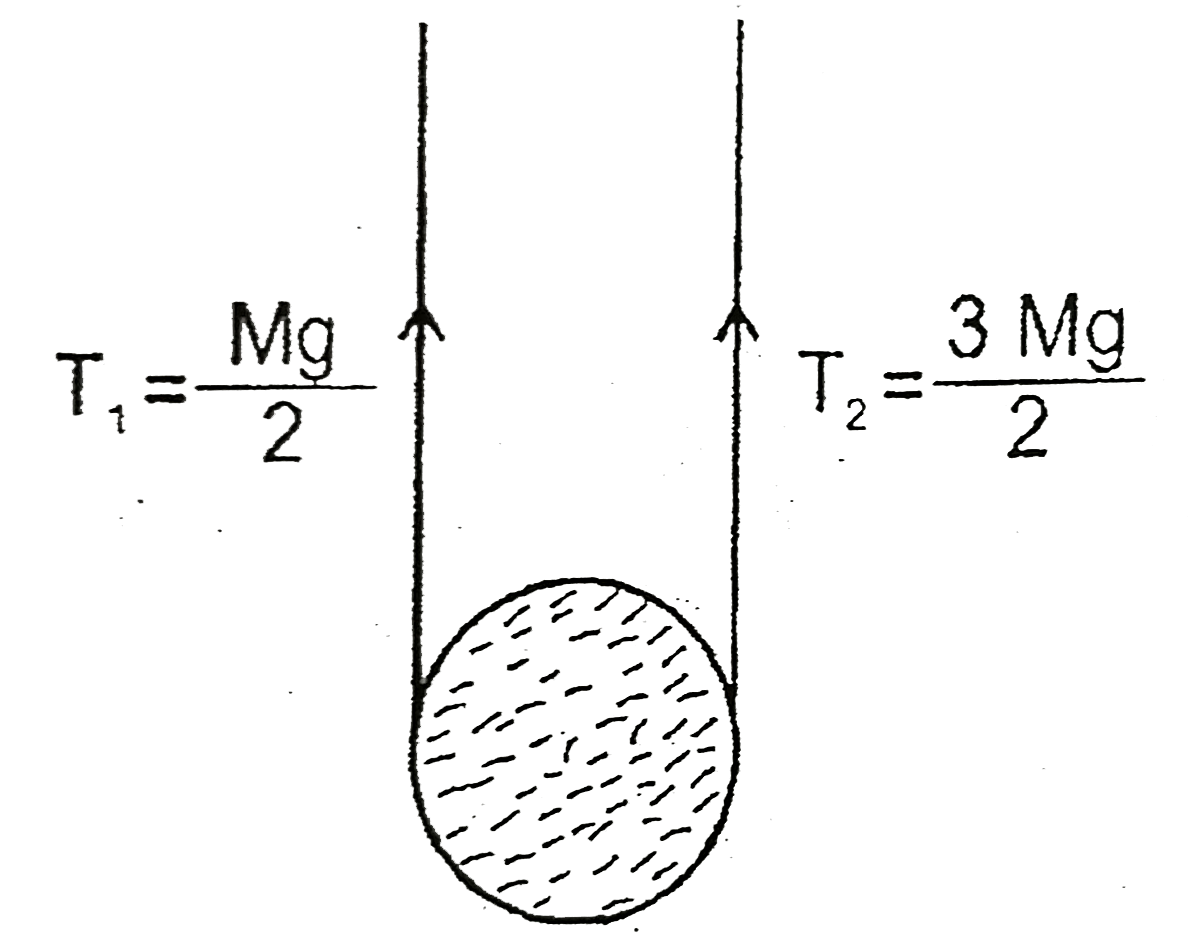
View SolutionA uniform disc of mass M and radius R is liffted using a string as shown in the figure. Then choose incorrect option(s),
 View Solution
View SolutionA uniform metal disck of radius R is taken and out of it a disc of diameter R is cut-off from the end. The center of mass of the remaining part will be
View SolutionThree forces of magnitude F, F and √2F are acting on the periphery of a disc of mass m and radius R as shown in the figure. The net torque about the centre of the disc is
View SolutionThe distance of center of mass from the center of a uniform disc of radius R. if a circular plate is removed from the disc as shown in figure will be (C is centre of complete disc)
View Solution