A cylinderical vessel is filled with water up to height H. A hole is bored in the wall at a depth h from the free surface of water. For maximum range h is equal to
H
3H/4
H/2
H/4
The correct Answer is:C
x=2√h(H−1)
for xmaxdxdh=0 or h=H2
Topper's Solved these Questions
- FLUID MECHANICSBook:RESONANCE ENGLISHChapter:FLUID MECHANICSExercise:Exercise- 2 PART - I
Explore 18 Videos
- FLUID MECHANICSBook:RESONANCE ENGLISHChapter:FLUID MECHANICSExercise:Exercise- 2 PART - II
Explore 6 Videos
- FLUID MECHANICSBook:RESONANCE ENGLISHChapter:FLUID MECHANICSExercise:Exercise- 1 PART - II Section (B)
Explore 7 Videos
- ELECTROSTATICSBook:RESONANCE ENGLISHChapter:ELECTROSTATICSExercise:Exercise
Explore 52 Videos
- FULL TEST 1Book:RESONANCE ENGLISHChapter:FULL TEST 1Exercise:Exercise
Explore 30 Videos
Similar Questions
A cylindrical vessel is filled with a liquid up to a height H. A small hole is made in the vessel at a distance y below the liquid surface as shown in figure. The liquid emerging from the hole strike the ground at distance x
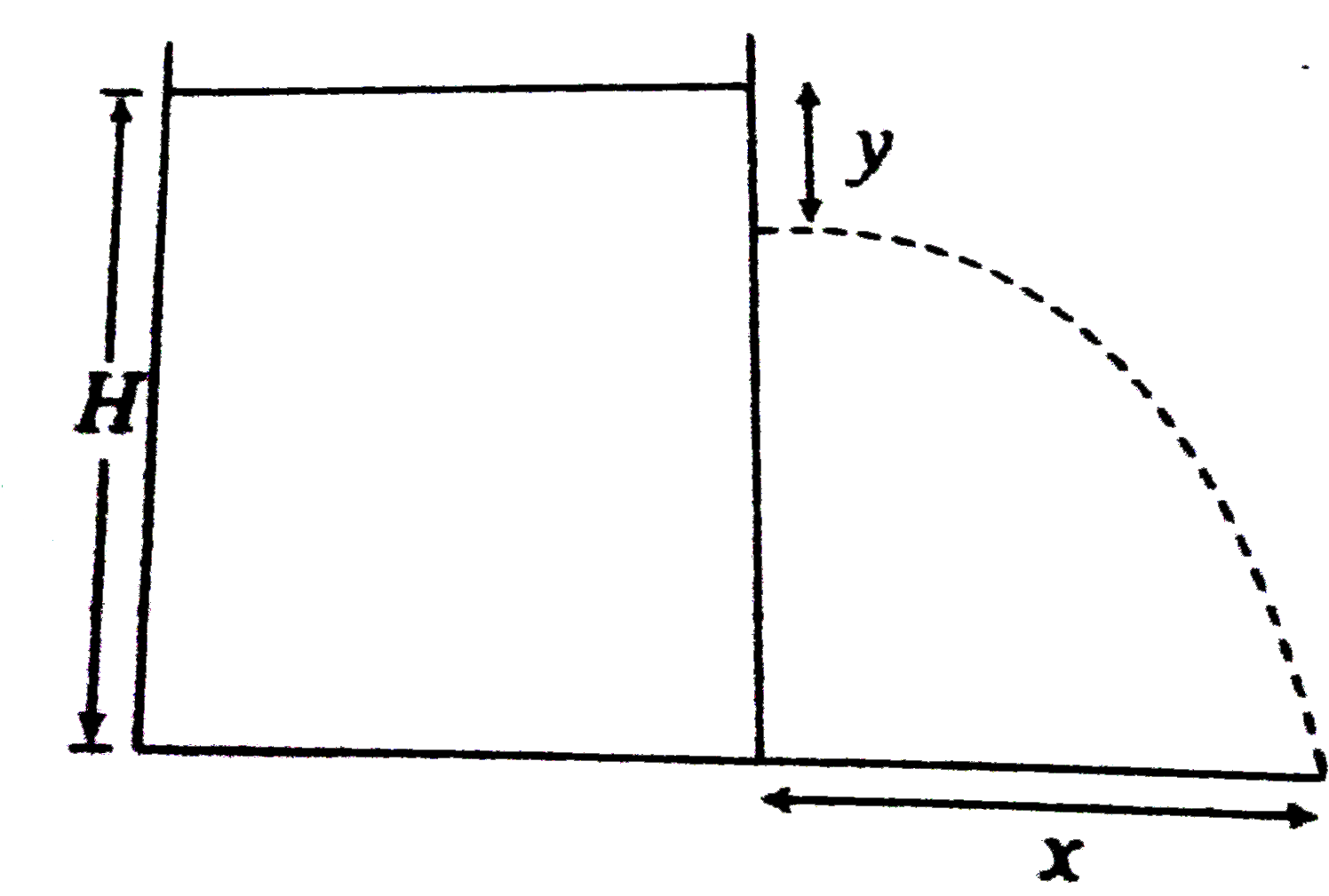
View SolutionA tank is filled with water upto a height H. A hole is punched in one of the walls at a depth h1 below the water surface. (a) Find the distance from the foot of the wall at which the stream strikes the floor. (6) Is it possible to make second hole at another depth so that this second stream also has the same range? If so find its depth ?
View Solution
Knowledge Check
A cylindrical vessel is filled with a liquid up to a height H. A small hole is made in the vessel at a distance y below the liquid surface as shown in figure. The liquid emerging from the hole strike the ground at distance x
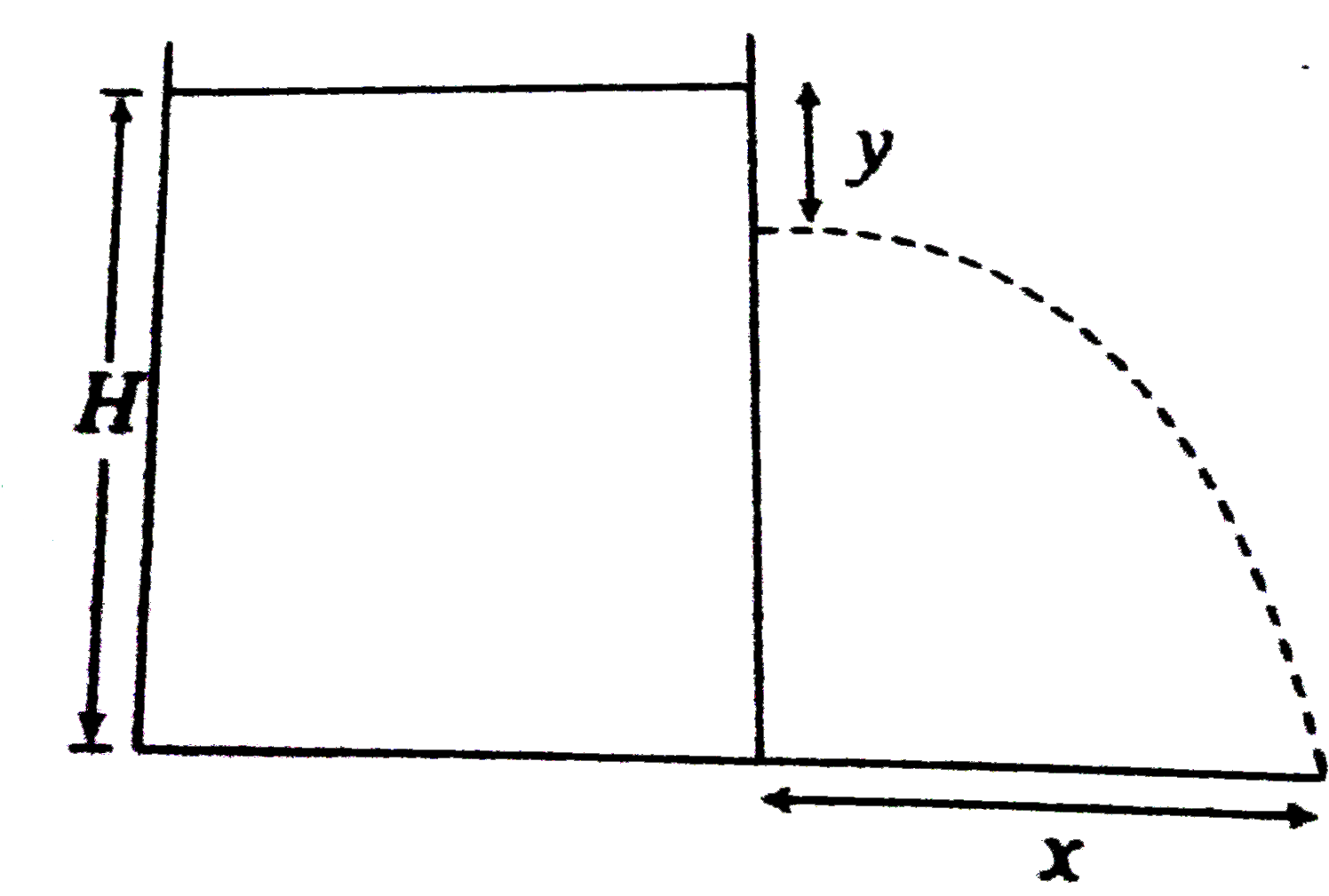 Ax is equal if hole is at depth y or H-yBx is maximum for y=H/2CBoth (a ) and (b) are correctDBoth (d) and (d) are wrong
Ax is equal if hole is at depth y or H-yBx is maximum for y=H/2CBoth (a ) and (b) are correctDBoth (d) and (d) are wrongA tank with vertical walls is mounted so that its base is at a height H above the horizontal ground. The tak is filled with water to a depth h. A hole is puched in the side wall of the tank at a depth x below the water surface. To have maximum range of the emerging stream, the value of x is
AH+h4BH+h2CH+h3D3(H+h)4A tank is filled with water up to a height H. Water is allowed to come out of a hole P in one of the walls at a depth D below the surface of water. Express the horizontal distance x in terms of H and D
 .Ax=√D(H−H)Bx=√D(H−D)2Cx=2√D(H−D)Dx=4√D(H−D)
.Ax=√D(H−H)Bx=√D(H−D)2Cx=2√D(H−D)Dx=4√D(H−D)
A tank is filled with water to a height H. A hole is punched in the wall at a depth h below the water surface. Find the distance x from the wall at which the stream strikes the floor. Could a hole be punched at another depth so that this second stream would have the same range? If so at what depth? For what value of h is range maximum?
View SolutionA tank is filled to a height H. The range of water coming out of a hole which is a depth H/4 from the surface of water level is
View SolutionA tank if filled with water upto height H. When a hole is made at a distance h below the level of water. What will be the horizontal range of water jet ?
View SolutionA tank is filled to a height H. The range of water coming out of a hole which is a depth H/4 from the surface of water level is
View SolutionA tank if filled with water upto height H. When a hole is made at a distance h below the level of water. What will be the horizontal range of water jet ?
View Solution
