Choose the most appropriate graph of iminv/sA, for a light ray which is incident on one face of a prism and is coming out from the opposite face (without TIR). (The symbols have their standard meanings).
A. 
B. 
C. 
D. 
The correct Answer is:B
To solve the problem of determining the appropriate graph of iminv/sA for a light ray incident on a prism, we need to analyze the relationship between the prism angle (A) and the minimum angle of incidence (i_min) under the condition that the light ray exits from the opposite face without undergoing total internal reflection (TIR).
1. Understanding the Prism and Angles:
- A prism has an apex angle denoted as A.
- When light enters the prism, it refracts at the first face and exits at the second face.
- The angles of refraction at the two faces are denoted as r1 and r2.
2. Conditions for No Total Internal Reflection:
- Total internal reflection occurs when the angle of incidence exceeds the critical angle (c) for the material of the prism.
- For light to exit the prism without TIR, the angle of refraction (r2) must be less than the critical angle (c).
3. Relation Between Angles:
- The relationship between the prism angle (A) and the angles of refraction can be expressed as:
\(
A = r1 + r2
\)
- For light to emerge from the prism, we must ensure that both r1 and r2 are less than the critical angle (c).
4. Analyzing Different Ranges of A:
- Case 1: When A < c
- In this case, both r1 and r2 can be adjusted such that they remain below the critical angle. Therefore, i_min can be zero, meaning that light can enter the prism at any angle.
- Case 2: When c < A < 2c
- Here, r1 can still be less than c, but r2 may approach or exceed c, leading to the possibility of TIR. However, there is still a range of i_min that allows for emergence.
- Case 3: When A > 2c
- In this scenario, r2 will definitely exceed the critical angle, leading to TIR. Thus, i_min will again be zero since no light can emerge.
5. Conclusion:
- From the analysis, we find that the minimum angle of incidence (i_min) is zero when A is less than c and also when A is greater than 2c.
- Between these ranges, there exists a non-zero minimum angle of incidence. Therefore, the graph that represents this relationship will show that i_min is zero for A < c and A > 2c, and it will rise as A approaches the critical angle.
Final Answer:
The correct graph representing the relationship between imin and A is Option B, which shows that i_min is zero for A < c and A > 2c, and increases in between these ranges.
- A prism has an apex angle denoted as A.
- When light enters the prism, it refracts at the first face and exits at the second face.
- The angles of refraction at the two faces are denoted as r1 and r2.
- Total internal reflection occurs when the angle of incidence exceeds the critical angle (c) for the material of the prism.
- For light to exit the prism without TIR, the angle of refraction (r2) must be less than the critical angle (c).
- The relationship between the prism angle (A) and the angles of refraction can be expressed as:
\(
A = r1 + r2
\)
- For light to emerge from the prism, we must ensure that both r1 and r2 are less than the critical angle (c).
- Case 1: When A < c
- In this case, both r1 and r2 can be adjusted such that they remain below the critical angle. Therefore, i_min can be zero, meaning that light can enter the prism at any angle.
- Case 2: When c < A < 2c
- Here, r1 can still be less than c, but r2 may approach or exceed c, leading to the possibility of TIR. However, there is still a range of i_min that allows for emergence.
- Case 3: When A > 2c
- In this scenario, r2 will definitely exceed the critical angle, leading to TIR. Thus, i_min will again be zero since no light can emerge.
- From the analysis, we find that the minimum angle of incidence (i_min) is zero when A is less than c and also when A is greater than 2c.
- Between these ranges, there exists a non-zero minimum angle of incidence. Therefore, the graph that represents this relationship will show that i_min is zero for A < c and A > 2c, and it will rise as A approaches the critical angle.
The correct graph representing the relationship between imin and A is Option B, which shows that i_min is zero for A < c and A > 2c, and increases in between these ranges.
Topper's Solved these Questions
- TEST PAPERSBOOK - RESONANCE ENGLISHCHAPTER - TEST PAPERSEXERCISE - PART - II PHYSICS
106 Videos
- SIMPLE HARMONIC MOTION BOOK - RESONANCE ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Advanced Level Problems
13 Videos
- TEST SERIESBOOK - RESONANCE ENGLISHCHAPTER - TEST SERIESEXERCISE - PHYSICS
130 Videos
Similar Questions
What is the required condition, if the light incident on one face of a prism, does not emerge from the other face?
View SolutionA light ray is incident by grazing one of the face of a prism and after refraction ray does not emerge out, what should be the angle of prism while critical angle is C ?
View Solution
Knowledge Check
A ray of light is incident at 60∘ on one face of a prism of angle 30∘ and the emergent ray makes 30∘ with the incident ray. The refractive index of the prism is
A1.732B1.414C1.5D1.33
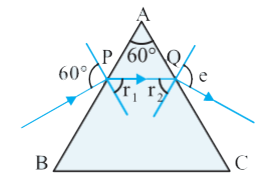
A ray of light is incident on one face of a prism (m=√3) at an angle of 60∘. The refracting angle of the prism is also 60∘. Find the angle of emergence .
View SolutionA light ray is incident normally on one of the refracting faces of a prism and just emerges out grazing the second surface. Then, the relation between angle of the prism and its critical angle is
View SolutionA light ray is incident normally on one of the refracting faces of a prism and just emerges out grazing the second surface. Then, the relation between angle of the prism and its critical angle is
View SolutionA ray of light is incident at 60∘ on one face of a prism of angle 30∘ and the emergent ray makes 30∘ with the incident ray. The refractive index of the prism is
View SolutionDraw a ray diagram to illustrate how a ray of light incident obliquely on one face of a rectangular glass slab of uniform thickness emerges.
View SolutionIf a light ray incidents normally on one of the faces of the prism of refractive index 2 and the emergent ray just grazes the second face of the prism, then the angle of deviation is
View SolutionIf a light ray incidents normally on one of the faces of the prism of refractive index 2 and the emergent ray just grazes the second face of the prism, then the angle of deviation is
View Solution
