A simple pendulum of length 'l' and having bob of mass 'm' is doing angular SHM inside water A constant buoyant force equal to half the weight of he bob is acting on the ball. Find the time period of oscillation?
To find the time period of oscillation of a simple pendulum bob submerged in water, where a constant buoyant force equal to half the weight of the bob is acting on it, we can follow these steps:
1. Identify the Forces Acting on the Bob:
- The weight of the bob (W) is given by:
W=mg
- The buoyant force (B) acting on the bob is given as half of its weight:
B=12mg
2. Determine the Effective Weight:
- The effective weight (W_eff) of the bob when submerged in water can be calculated by subtracting the buoyant force from the weight:
Weff=W−B=mg−12mg=12mg
3. Calculate the Effective Acceleration Due to Gravity (g_eff):
- The effective force acting on the bob can be expressed as:
Weff=m⋅geff
- Setting the two expressions for effective weight equal gives:
12mg=mgeff
- Dividing both sides by m (assuming m≠0):
geff=g2
4. Use the Formula for the Time Period of a Simple Pendulum:
- The time period T of a simple pendulum is given by:
T=2π√lgeff
- Substituting geff=g2 into the formula:
T=2π
⎷lg2
- This simplifies to:
T=2π√2lg
5. Final Result:
- The time period of oscillation of the pendulum bob in water is:
T=2π√2lg
- The weight of the bob (W) is given by:
W=mg
- The buoyant force (B) acting on the bob is given as half of its weight:
B=12mg
- The effective weight (W_eff) of the bob when submerged in water can be calculated by subtracting the buoyant force from the weight:
Weff=W−B=mg−12mg=12mg
- The effective force acting on the bob can be expressed as:
Weff=m⋅geff
- Setting the two expressions for effective weight equal gives:
12mg=mgeff
- Dividing both sides by m (assuming m≠0):
geff=g2
- The time period T of a simple pendulum is given by:
T=2π√lgeff
- Substituting geff=g2 into the formula:
T=2π
⎷lg2
- This simplifies to:
T=2π√2lg
- The time period of oscillation of the pendulum bob in water is:
T=2π√2lg
Topper's Solved these Questions
- SIMPLE HARMONIC MOTION BOOK - RESONANCE ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Solved Miscellaneous Problems
9 Videos
- SIMPLE HARMONIC MOTION BOOK - RESONANCE ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Board Level Exercise
24 Videos
- SEMICONDUCTORSBOOK - RESONANCE ENGLISHCHAPTER - SEMICONDUCTORSEXERCISE - Exercise 3
88 Videos
- TEST PAPERSBOOK - RESONANCE ENGLISHCHAPTER - TEST PAPERSEXERCISE - PHYSICS
784 Videos
Similar Questions
A simple pendulum of length 1 m with a bob of mass m swings with an angular amplitude 30∘. Then (g=9.8m/s2
View SolutionA simple pendulum of length l is pulled aside to make an angle θ with the vertical. Find the magnitude of the torque of the weight w of the bob about the point of suspension. When is the torque zero?
View Solution
Knowledge Check
A simple pendulum of length l and having a bob of mass M is suspended ina car. The car is moving on a circular track of radius R with a uniform speed v. If the pendulum makes small oscillations in a radial direction about its equilibrium, what will be its time period ?
AT=2π√LgBT=2π ⎷L√g2+v4R2CT=2π ⎷L√g2+v2RDT=2π ⎷Lg2−v4R2
A spring of force constant 'k' is cut into four equal parts one part is attached with a mass m. The time period of oscillation will be
View SolutionA simple pendulum of length l and having a bob of mass M is suspended ina car. The car is moving on a circular track of radius R with a uniform speed v. If the pendulum makes small oscillations in a radial direction about its equilibrium, what will be its time period ?
View SolutionA simple pendulum of length l and mass m is suspended in a car that is moving with constant speed v around a circle of radius r. Find the period of oscillation and equilibrium position of the pendulum.
View SolutionA simple pendulum of length l and bob of mass m is displaced from its equilibrium position O to a position P so that hight of P above O is h . If is then released. What is the tension in the string when the bob passes through the through the equilibrium position O ? Neglect friction. v is the velocity of the bob at O
View SolutionA simple pendulum of length l and bob mass m is hanging in front of a large nonconducting sheet having surface charge density σ. If suddenly a charge +q is given to the bob & it is released from the position shown in figure. Find the maximum angle through which the string is deflected from vertical .
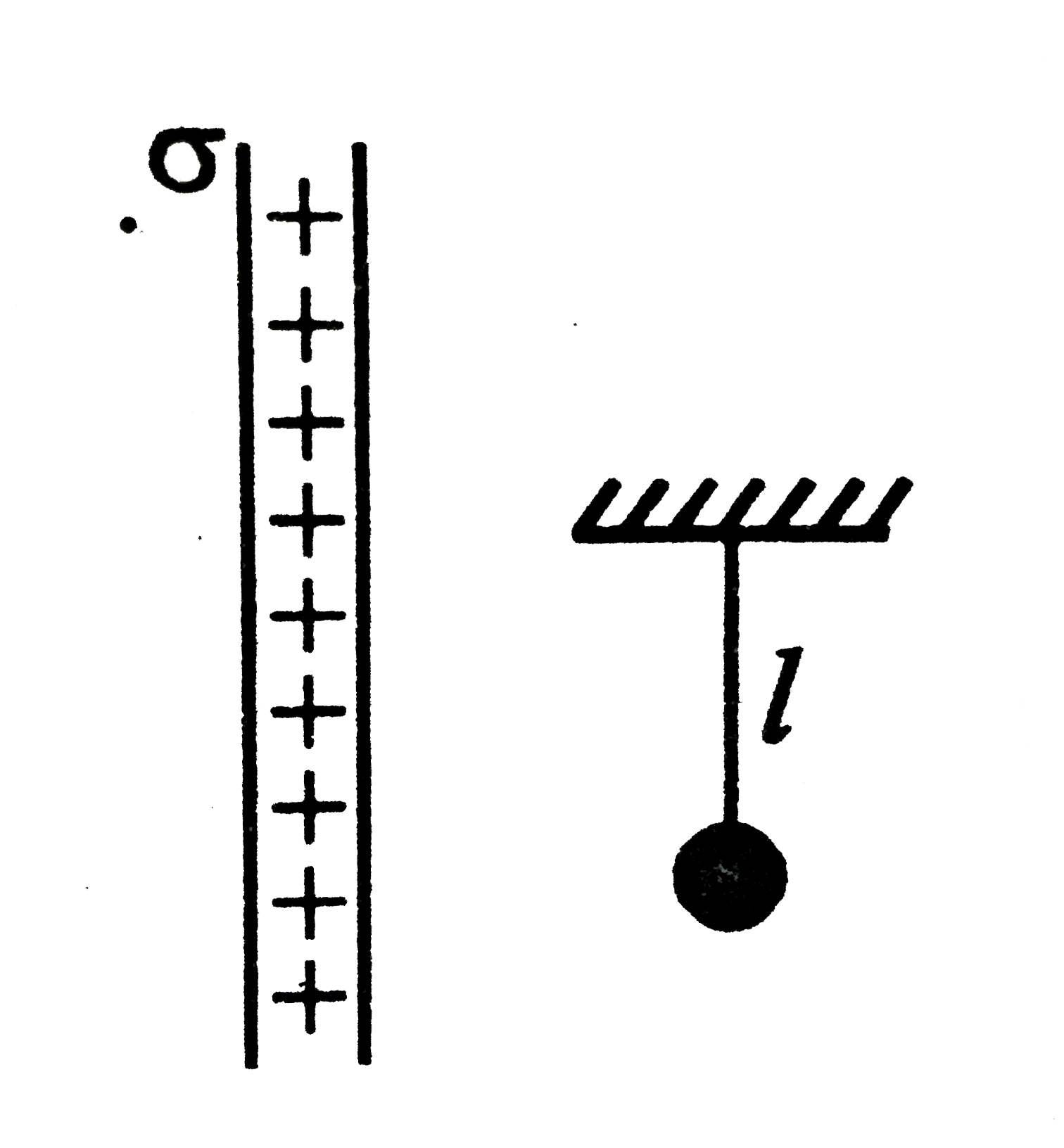 .
.
View SolutionA simple pendulum is hanging from the roof of a trolley which is moving horizontally with acceleration g. If length of the string is L and mass of the bob is m, then time period of oscillation is
View SolutionA simple pendulum of length 1 oscillates aboμt the mean position as shown in the figure. If the total energy of the pendulum is E, the velocity of the pendulum bob of mass m at point P is:
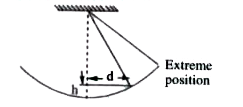
View Solution
