A prism (n=2) of apex angle 90∘ is palced in air (n=1). What should be the angle of incidence so that light ray strikes the second surface at an angle of incidence 60∘.
The correct Answer is:90∘
To solve the problem, we need to find the angle of incidence I such that a light ray strikes the second surface of a prism with an apex angle of 90∘ at an angle of incidence of 60∘. The refractive index of the prism is n=2 and that of air is n=1.
1. Understanding the Geometry of the Prism:
- The prism has an apex angle A=90∘.
- The light ray enters the prism from air and strikes the first surface (AB) of the prism.
2. Identifying Angles:
- Let r be the angle of refraction at the first surface (AB).
- The angle of incidence at the second surface (BC) is given as 60∘.
3. Using the Prism Formula:
- For a prism, the relationship between the angle of incidence I, angle of refraction r, and the angle of the prism A is given by:
r+r′=A
- Here, r′ is the angle of refraction at the second surface (BC).
- Since A=90∘, we have:
r+r′=90∘
- Given that r′=60∘, we can find r:
r+60∘=90∘⟹r=30∘
4. Applying Snell's Law at the First Surface (AB):
- Snell's Law states:
n1sinI=n2sinr
- Here, n1=1 (air), n2=2 (prism), and r=30∘.
- Substituting the values:
1⋅sinI=2⋅sin30∘
- We know sin30∘=12, so:
sinI=2⋅12=1
5. Finding the Angle of Incidence:
- Since sinI=1, we find:
I=90∘
Final Answer:
The angle of incidence I should be 90∘.
- The prism has an apex angle A=90∘.
- The light ray enters the prism from air and strikes the first surface (AB) of the prism.
- Let r be the angle of refraction at the first surface (AB).
- The angle of incidence at the second surface (BC) is given as 60∘.
- For a prism, the relationship between the angle of incidence I, angle of refraction r, and the angle of the prism A is given by:
r+r′=A
- Here, r′ is the angle of refraction at the second surface (BC).
- Since A=90∘, we have:
r+r′=90∘
- Given that r′=60∘, we can find r:
r+60∘=90∘⟹r=30∘
- Snell's Law states:
n1sinI=n2sinr
- Here, n1=1 (air), n2=2 (prism), and r=30∘.
- Substituting the values:
1⋅sinI=2⋅sin30∘
- We know sin30∘=12, so:
sinI=2⋅12=1
- Since sinI=1, we find:
I=90∘
The angle of incidence I should be 90∘.
Topper's Solved these Questions
- GEOMATRICAL OPTICS BOOK - RESONANCE ENGLISHCHAPTER - GEOMATRICAL OPTICS EXERCISE - Exercise-2
62 Videos
- GEOMATRICAL OPTICS BOOK - RESONANCE ENGLISHCHAPTER - GEOMATRICAL OPTICS EXERCISE - Exercise-3
80 Videos
- GEOMATRICAL OPTICS BOOK - RESONANCE ENGLISHCHAPTER - GEOMATRICAL OPTICS EXERCISE - Problem
33 Videos
- EXPERIMENTAL PHYSICSBOOK - RESONANCE ENGLISHCHAPTER - EXPERIMENTAL PHYSICSEXERCISE - PART -II
10 Videos
- GRAVITATIONBOOK - RESONANCE ENGLISHCHAPTER - GRAVITATIONEXERCISE - HIGH LEVEL PROBLEMS
16 Videos
Similar Questions
When a ray of light strikes a plane mirror along the normal, the angle of incidence is :
View SolutionUnpolarized light is incident on a plane glass surface. What should be the angle of incidence so that the reflected and refracted rays are perpendicular to eachother ?
View SolutionA light ray is incident normally on a plane mirror.
What is the angle of incidence?
View SolutionFigure shows graph of deviation δ versus angle of incidence for a light ray striking a prism. Angle of prism is
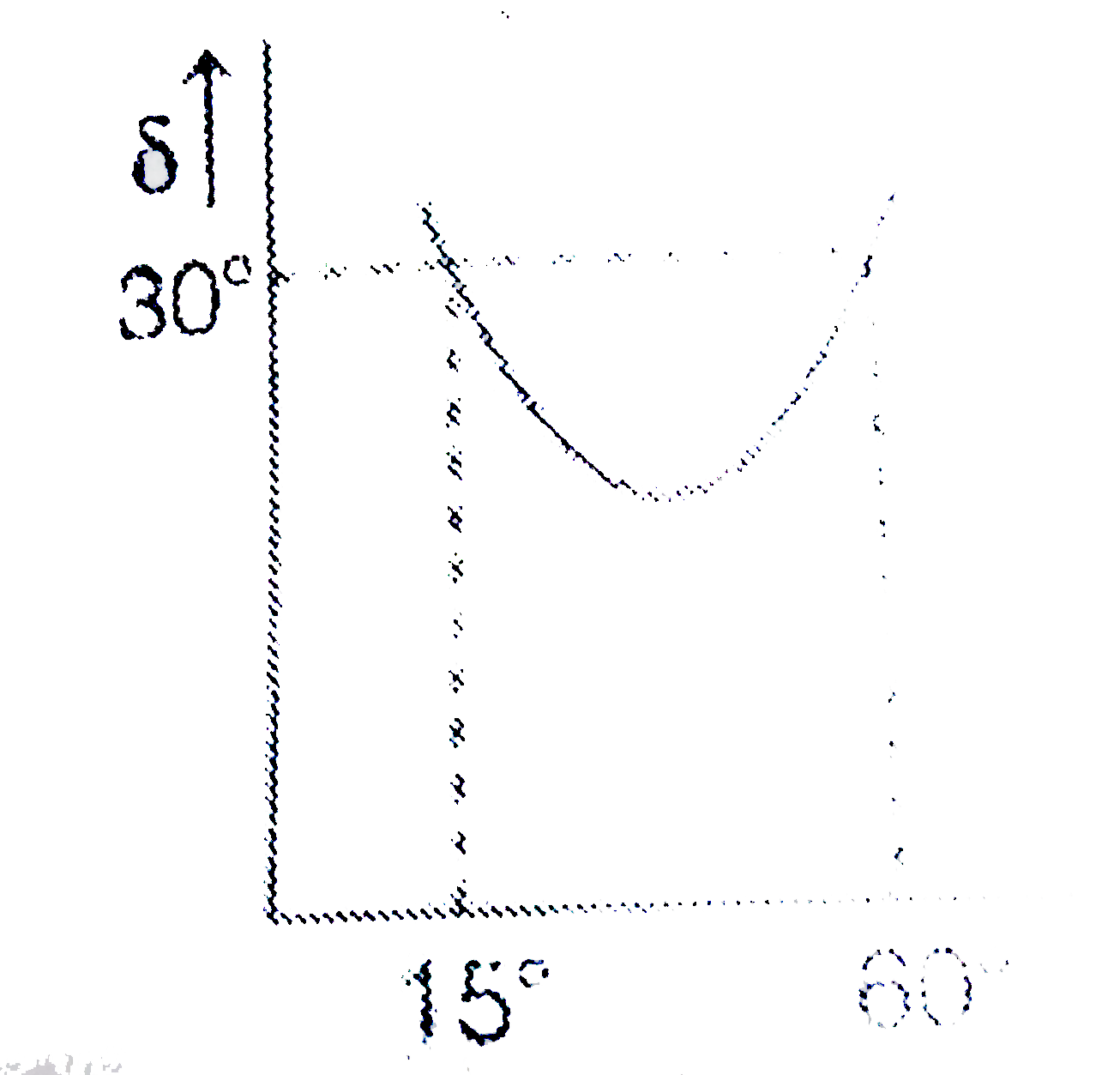
View SolutionWhat should be the angle of incidence for a ray of light which suffers minimum deviation of 36∘ through an equilateral prism ?
View SolutionIf the angle of incidence is 32∘, then what will the angle between incident ray and reflected ray?
View SolutionLight ray is incident on a prism of angle A=60∘ are refractive index μ=√2. The angle of incidence which the emergent rays grazes the surface is given
View SolutionA ray of light passes from vaccum into a medium of refractive index n. If the angle of incidence is twice the angle of refraction, then the angle of incidence is
View SolutionFor a prism, A=60∘,n=√7/3 . Find the minimum possible angle of incidence, so that the light ray is refracted from the second surface. Also, find δmax.
View SolutionA prism of apex angle A=60∘ has the refractive index μ=√2. The angle of incidence for minimum deviation is
View Solution
