Use Biot-Savart law to derive the expression for the magnetic field on the axis of a current carrying circular loop of radius R.
Draw the magnetic field lines due to circular wire carrying current I.
Consider a circular loop of radius r carrying current I ampere, held perpendicular to the plane of the paper.We want to find the magnetic field at point P which is at a distance x from the centre of the coil.Let us take a small element ab of length dl.From Biot-Savart's Law, the field at point P due to small elements is given by:The direction of the field is in a plane perpendicular to the plane containing dl and is at right angle to line AP Resolve this into two components dBsinα along the x-axis and dBcosα at right angle to the line OP.It is clear from the figure that the resultant field along xaxis (i.e.,component dBcosα cancel each other)is zero.Field the total field at P due to all such elements is given by
B=∫dBsinα=∫μ0l4π(r2+x2)dlsinα
=μ0Isinα4π(r2+x2)∫dl=μ0Isinα4π(r2+x2)[∴∫dl=2πr]
sinα=r√r2+x2substuting the values, we get
B=μ0lr22(r2+x2)3/2
If there are n turns in the coil then B=μ0nIr22(r2+x2)3/2
The magnetic field lines due to a circular current carrying loop are shown below:


Topper's Solved these Questions
- BOOK - RESONANCE ENGLISHCHAPTER - ELECTRODYNAMICSEXERCISE - Advanced level problems31videos
ELECTRODYNAMICS
- BOOK - RESONANCE ENGLISHCHAPTER - ELECTRODYNAMICSEXERCISE - Exercise-3 PART-227videos
ELECTRODYNAMICS
- BOOK - RESONANCE ENGLISHCHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - Exercise 327videos
ELECTRO MAGNETIC WAVES
- BOOK - RESONANCE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - A.l.P19videos
ELECTROMAGNETIC INDUCTION
Similar Questions
What will be magnetic field at centre of current carrying circular loop of radius R?
View SolutionDraw the pattern of magnetic field lines for a circular coil carrying current.
View SolutionThe magneitc field produced at the center of a current carrying circular coil of radius r, is
View SolutionUsing Biot-Savart’s law, derive an expression for magnetic field at any point on axial line of a current carrying circular loop. Hence, find magnitude of magnetic field intensity at the centre of circular coil.
View SolutionThe magnetic field in a straight current carrying conductor wire is:
View SolutionFind the magnetic field induction at a point on the axis of a circular coil carrying current and hence find the magnetic field at the centre of circular coil carrying current.
View SolutionDraw magnetic field lines in and around a current carrying straight solenoid.
View Solution(a) Using Biot-Savart's law, derive an expression for the magnetic field at the centre of a circular coil of radius R, number of turns N, carrying current.
(b) Two small identical circular coils marked 1,2 carry equal currents and are placed with their geometric axes perpendicular to each other as shown in the figure. Derive an expression for the resultant magnetic field at O.
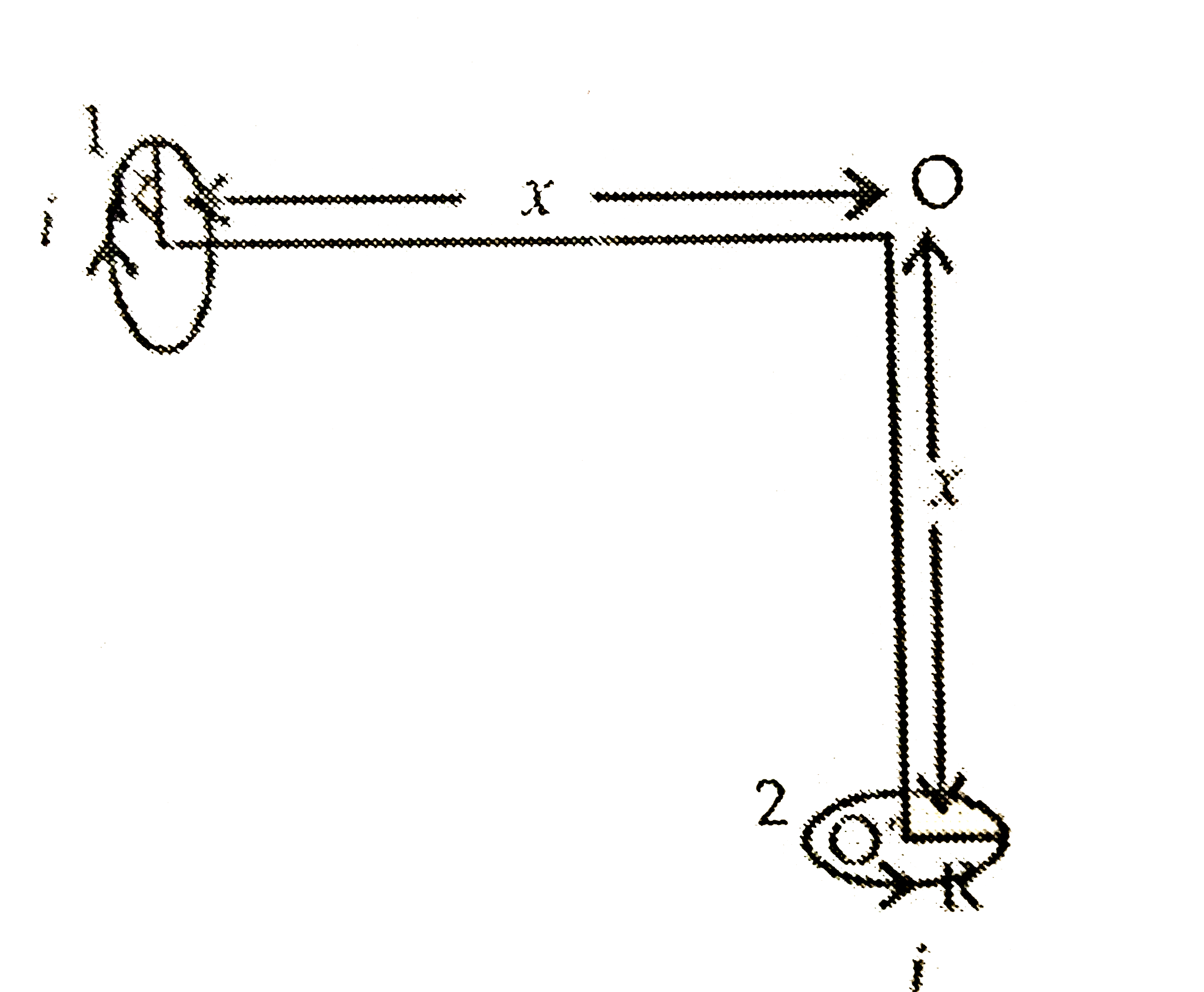 View Solution
View SolutionState Biot-Savart law, giving the mathematical expression for it.
Use this law to derive the expression for the magnetic field due to a circular coil carrying current at a point along its axis.
How does a circular loop carrying curent behave as a magnet?View Solution(a) Using Biot-Savart's law, derive the expression for the magnetic field in the vector form at a point on the axis of a circular current loop.
(b) What does a toroid consist of? Find out the expression for the magnetic field inside a toroid for N turns of the coil having the average radius r and carrying a current I. Show the magnetic field in the open space inside and exterior to the toroid is Zero.View Solution

