Fig. shows a surface XY separating two transparent media, medium 1 and medium 2. Lines ab and cd represent wavefronts of a light wave travelling in medium 1 and incident on XY. Line ef and gh represent wavefront of the light wave in medium 2 after rafraciton. Speed of light is
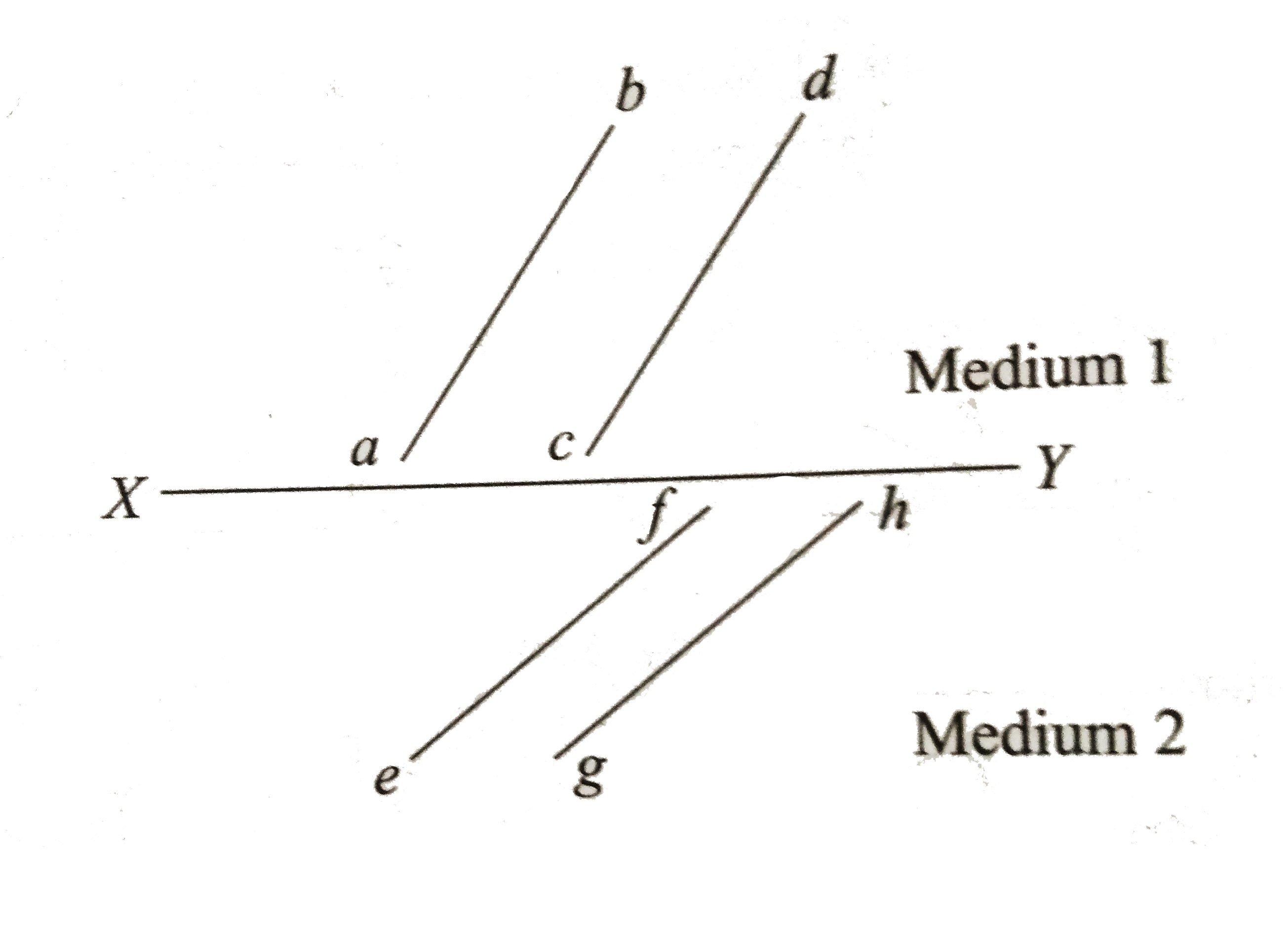
ϕc cannot be equal to ϕd
ϕd cannot be equal to ϕe
(ϕd−ϕf) is equal to (ϕc−ϕe)
(ϕd−ϕc) is equal to (ϕf−ϕe)
The correct Answer is:C
c and d are at same wavefronts
So, ϕc=ϕd
and ϕe=ϕf
∴ϕd−ϕf=ϕc−ϕf
D is incorrect
ϕd−ϕc=0
ϕf−ϕe=0
So both should be equal.
Topper's Solved these Questions
- BOOK - RESONANCE ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Exercise-3 (Part-2)15videos
WAVE OPTICS
- BOOK - RESONANCE ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Exercise-3 (Part-3)22videos
WAVE OPTICS
- BOOK - RESONANCE ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Exercise-2 (Part-4)8videos
WAVE OPTICS
- BOOK - RESONANCE ENGLISHCHAPTER - WAVE ON STRING EXERCISE - Exercise- 3 PART II7videos
WAVE ON STRING
Similar Questions
Fig. shows a surface XY separating two transparent media, medium 1 and medium 2. Lines ab and cd represent wavefronts of a light wave travelling in medium 1 and incident on XY. Line ef and gh represent wavefront of the light wave in medium 2 after rafraciton.
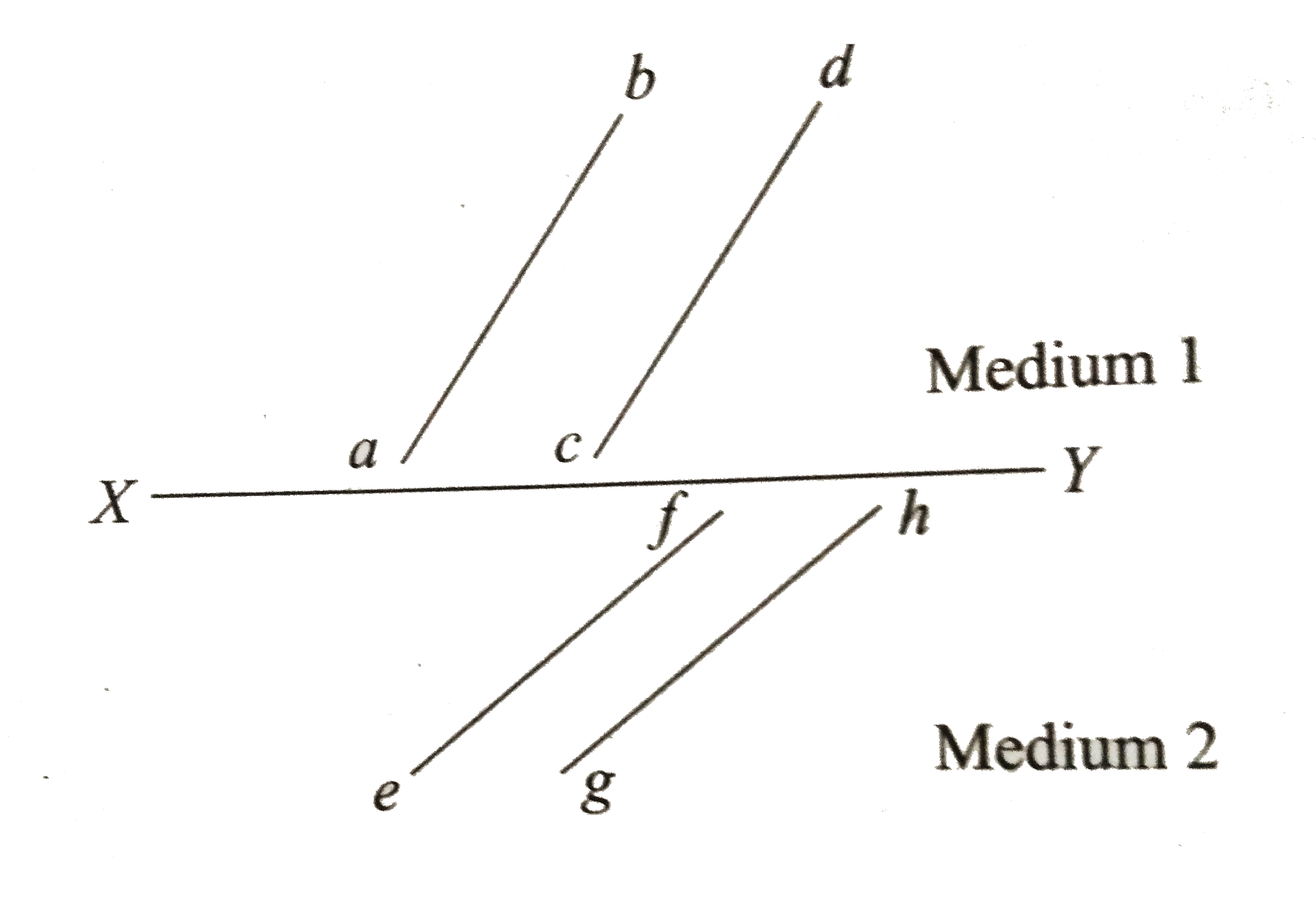
Light travel as aView SolutionFig. shows a surface XY separating two transparent media, medium 1 and medium 2. Lines ab and cd represent wavefronts of a light wave travelling in medium 1 and incident on XY. Line ef and gh represent wavefront of the light wave in medium 2 after rafraciton.

The phase of the ligth wave at c, d, e, and f are ϕc, phi_(d), ϕe and ϕf, respectively. It is given that ϕc≠ϕf. ThenView Solution
Knowledge Check
- Question 1 - Select One
Consider two adjacent transparent media. The speed of light in medium 1 is v1, and the speed of light in medium 2 is v2. If v1<v2, then total internal reflection will occur at the interface between these media if a beam of light is
Aincident in medium 1 and strikes the interface at an angle of incidence greater than sin−1(v1/v2).Bincident in medium 1 and strikes the interface at an angle of incidence greater than sin−1(v2/v1).Cincident in medium 2 and strikes the interface at an angle of incidence greater than sin−1(v1/v2).Dincident in medium 2 and strikes the interface at an angle of incidence greater than sin−1(v2/v1).
Yellow light with wavefront 0.5μm is air surface refraction in a medium in which velocity of light is 2×10−8m/s. Then the wavelength of the light in the medium would be .
View SolutionA light wave of frequency 5×1014Hz enters a medium of refractive index 1.5. In the velocity of the light wave is ….. And its wavelength is ……
View SolutionThe critical angle for a given transparent medium and air is ic. A ray of light travelling in air is incident on this transparent medium at an angle of incidence equal to the polarising angle ip, What is the relation between the two angles ic and ip ?
View SolutionA spherical surface of radius 30 cm separates two transparent media A and B with refractive indices 1'33 and 1.48 respectively. The medium A is on the convex side of the surface. Where should a point object be placed in medium A so that the paraxial rays become parallel after refraction at the surface ?
View Solution(i) Find the speed of light of wavelength λ=780 nm (in air) in a medium of of refractive index µ=1.55.
(ii) What is the wave length of this light in the given medium?View SolutionA ray of light is incident at an angle α on the boundary separating two transparant media. It transmited in other medium. If the angle incidence is increased very slightly, the ray gets reflected in the same medium. The different between angles of deviation in the two cases will close to
View SolutionIf light travels a distance x in t1 sec in air and 10x distance in t2 sec in a medium, the critical angle of the medium will be
View Solution
