Consider a Body Centred Cube (BCC) arrangement, let $d_{e}, d_{f d}, d_{b d}$ be the distances between successive atoms located along the edge, the face-diagonal, the body diagonal respectively in a unit cell. Their order is given as:

A

$d_{e} < d_{f d} < d_{b d}$

B

$d_{f d} > d_{b d} > d_{e}$

C

$d_{f d} > d_{e} > d_{b d}$

D

$d_{b d} > d_{e} > d_{f d}$

Video Solution

Text Solution

Generated By DoubtnutGPT

The correct Answer is:C

To solve the problem regarding the distances between successive atoms in a Body-Centered Cubic (BCC) arrangement, we will follow these steps:
Step 1: Understand the BCC Structure
In a Body-Centered Cubic (BCC) arrangement, there are 8 atoms located at the corners of the cube and 1 atom located at the center of the cube.
Step 2: Identify the Distances
We need to find the distances between successive atoms along three different paths:
1. Edge Distance (D_E): This is the distance between two atoms located at the corners of the cube along one edge.
2. Face Diagonal Distance (D_FD): This is the distance between two atoms located at the corners of the cube along the face diagonal.
3. Body Diagonal Distance (D_BD): This is the distance between two atoms located at the corners of the cube along the body diagonal.
Step 3: Calculate the Distances
1. Distance along the Edge (D_E):
- The distance between two atoms along the edge of the cube is equal to the edge length of the cube, denoted as $A$ .
- Therefore, $D_{E} = A$ .
2. Distance along the Face Diagonal (D_FD):
- The face diagonal of a cube can be calculated using the Pythagorean theorem. The length of the face diagonal is given by $\sqrt{2} A$ .
- The distance between two atoms along the face diagonal is half of this length, so $D_{F} D = \frac{\sqrt{2} A}{2} = \frac{A \sqrt{2}}{2}$ .
3. Distance along the Body Diagonal (D_BD):
- The body diagonal of a cube can also be calculated using the Pythagorean theorem. The length of the body diagonal is given by $\sqrt{3} A$ .
- The distance between two atoms along the body diagonal is half of this length, so $D_{B} D = \frac{\sqrt{3} A}{2}$ .
Step 4: Compare the Distances
Now we have:
- $D_{E} = A$
- $D_{F} D = \frac{A \sqrt{2}}{2}$
- $D_{B} D = \frac{\sqrt{3} A}{2}$
To compare these distances, we can express them in terms of $A$ :
- $D_{E} = A$
- $D_{F} D = 0.707 A$ (approximately)
- $D_{B} D = 0.866 A$ (approximately)
Step 5: Determine the Order of Distances
From the values calculated:
- $D_{E} > D_{B} D > D_{F} D$
Thus, the order of distances is:
1. $D_{E}$ (Edge Distance)
2. $D_{B} D$ (Body Diagonal Distance)
3. $D_{F} D$ (Face Diagonal Distance)
Final Answer
The correct order of distances is:
$D_{E} > D_{B} D > D_{F} D$

Updated on:7/8/2024

|

Class 11 CHEMISTRY SOLID STATE

Topper's Solved these Questions

Similar Questions

Knowledge Check

The relationship between atomic radius (r) and the edge length 'a' of a body centred cubic unit cell is:
A $r = \frac{a}{2}$
B $r = \sqrt{\frac{a}{2}}$
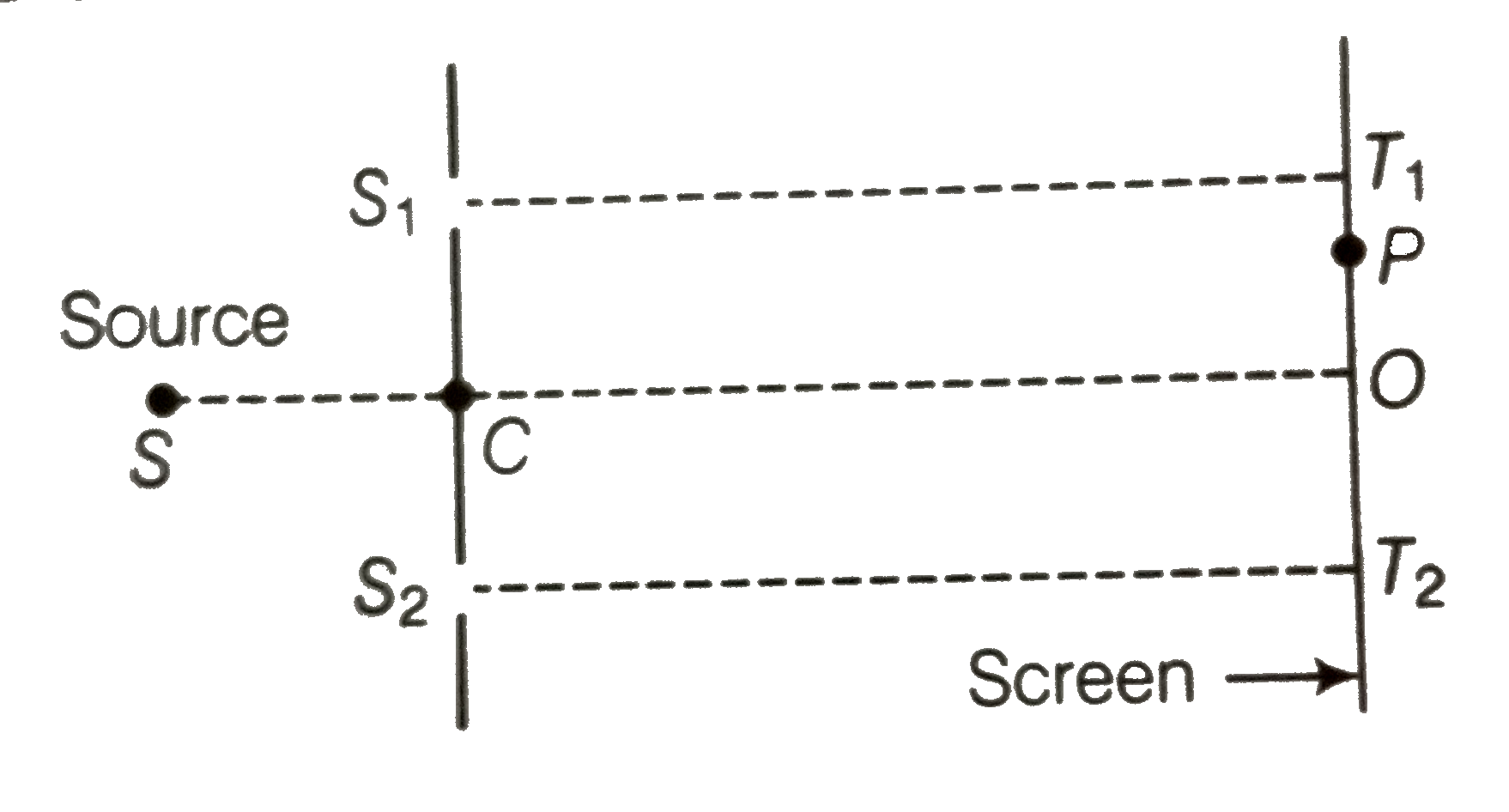
C $r = \frac{\sqrt{3}}{4} a$
D $r = \frac{3 a}{2}$
Consider a two slit interference arrangements (figure) such that the distance of the screen from the slits is half the distance between the slits. Obtain the value of D in terms of $λ$ such that the first minima on the screen falls at a distance D from the centre O.

A $\frac{λ}{2.472}$
B $\frac{λ}{2.236}$
C $\frac{λ}{1.227}$
D $\frac{λ}{3.412}$

RESONANCE ENGLISH-SOLID STATE-Part-II : Only one option correct type

Home
Ask Doubt
Profile