Concavity and convexity :
if f''(x)>0∀x∈(a,b) then the curve y=f(x) is concave up ( or convex down) in (a,b) and iff''(x)<0∀x∈(a,b) then the curve y=f(x) is concave down (or convex up ) in (a,b)
![Concavity and convexity : if f''(x) gt 0 AA x in (a,b) then the curve y=f(x) is concave up ( or convex down) in (a,b) and if f''(x) lt 0 AA x in (a,b) then the curve y=f(x) is concave down (or convex up ) in (a,b) Inflection point : The point where concavity of the curve changes is known as point of inflection (at inflection point f''(x) is equal to 0 or undefined) Exhaustive set of values of 'a' for which the function f(x) =x^(4) +ax^(3)+(3x^(2))/(2)+1 will be concave upward along the entire real line is :
(A) [-1,1]
(B) [-2,2]
(C) [0,2]
(D) [0,4]](https://d10lpgp6xz60nq.cloudfront.net/physics_images/RES_MATH_AOD_E02_202_Q01.png)
Inflection point :
The point where concavity of the curve changes is known as point of inflection (at inflection point f''(x) is equal to 0 or undefined)

Exhaustive set of values of 'a' for which the function f(x)=x4+ax3+3x22+1 will be concave upward along the entire real line is :
(A) [−1,1]
(B) [−2,2]
(C) [0,2]
(D) [0,4]
[−1,1]
[−2,2]
[0,2]
[0,4]
The correct Answer is:B
Step by step video & image solution for Concavity and convexity : if f''(x) gt 0 AA x in (a,b) then the curve y=f(x) is concave up ( or convex down) in (a,b) and if f''(x) lt 0 AA x in (a,b) then the curve y=f(x) is concave down (or convex up ) in (a,b) Inflection point : The point where concavity of the curve changes is known as point of inflection (at inflection point f''(x) is equal to 0 or undefined) Exhaustive set of values of 'a' for which the function f(x) =x^(4) +ax^(3)+(3x^(2))/(2)+1 will be concave upward along the entire real line is : (A) [-1,1] (B) [-2,2] (C) [0,2] (D) [0,4] by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- APPLICATION OF DERIVATIVESBOOK - RESONANCE ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Exersise-3 Part I
21 Videos
- APPLICATION OF DERIVATIVESBOOK - RESONANCE ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Exersise-3 Part II
22 Videos
- APPLICATION OF DERIVATIVESBOOK - RESONANCE ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Exersise-2 Part III
19 Videos
- COMBINATORICSBOOK - RESONANCE ENGLISHCHAPTER - COMBINATORICSEXERCISE - Exercise-2 (Part-II: Previously Asked Question of RMO)
5 Videos
Similar Questions
Concavity and convexity :
if f''(x)>0∀x∈(a,b) then the curve y=f(x) is concave up ( or convex down) in (a,b) and iff''(x)<0∀x∈(a,b) then the curve y=f(x) is concave down (or convex up ) in (a,b)

Inflection point :
The point where concavity of the curve changes is known as point of inflection (at inflection point f''(x) is equal to 0 or undefined)
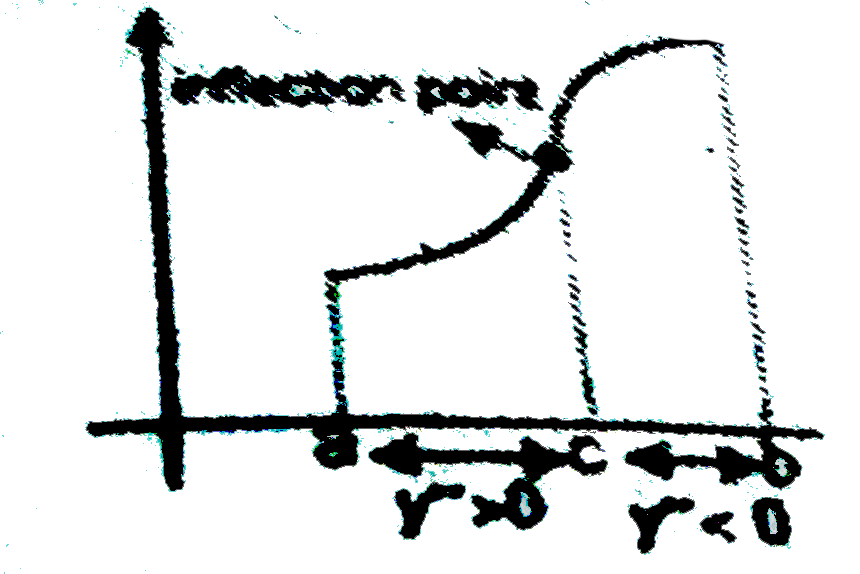
Number of point of inflection for f(x)=(x−1)3(x−2)2 is :
View SolutionLet g'(x)>0andf'(x)<0∀x∈R, then
View Solution
Knowledge Check
The least value of the function f(x)=ax+bx(x>0,a>0,b>0)
A√abB2√abCabD2ab
If f(x)>x;∀x∈R. Then the equation f(f(x))−x=0, has
View SolutionLet f(x) have a point of inflection at x=1 and let f(x)=x. If f′(1)=0, then f(x) is equal to
View SolutionFind the points of inflection for (a)f(x)=sinx (b)f(x)=3x4−4x3
View SolutionIf y=f(x) is differentiable ∀x∈R, then
View SolutionFind the coordinates of the point of inflection of the curve f(x) =e^(-x^(2))
View SolutionLet f(x) be a continuous function, ∀x∈R,f(0)=1andf(x)≠x for any x∈R, then show f(f(x))>x,∀x∈R+
View SolutionIf A and B are the points of intersection of y=f(x) and y=f−1(x), then
View Solution
