A particle of mass m, charge q>0 and initial kinetic energy K is projected from infinity towards a heavy nucleus of charge Q assumed to have a fixed position.
If the aim is perfect, how close to the center of the nucleus is the particle when it comes instantaneously to rest ?
The correct Answer is:(a) Qq4πε0K (b) √Km
To solve the problem, we need to apply the principle of conservation of energy. The initial kinetic energy of the particle will be converted into electrostatic potential energy as it approaches the nucleus.
1. Understand the Initial Conditions:
- A particle of mass m and charge q>0 is projected from infinity towards a nucleus of charge Q.
- The initial kinetic energy of the particle is K.
2. Identify Energy Conservation:
- At infinity, the potential energy is zero, and the total energy is purely kinetic, given by K.
- As the particle approaches the nucleus, it slows down due to the electrostatic force and will eventually come to rest at some distance R from the nucleus.
3. Set Up the Energy Equation:
- When the particle comes to rest at distance R, all its initial kinetic energy K is converted into electrostatic potential energy U.
- The electrostatic potential energy U between two point charges is given by:
U=14πϵ0QqR
4. Apply Conservation of Energy:
- According to the conservation of energy:
K=U
- Substituting the expression for potential energy:
K=14πϵ0QqR
5. Rearranging to Find R:
- Rearranging the equation to solve for R:
R=14πϵ0QqK
6. Final Expression:
- Thus, the distance R at which the particle comes to rest is given by:
R=Qq4πϵ0K
Final Answer:
The closest distance to the center of the nucleus when the particle comes instantaneously to rest is:
R=Qq4πϵ0K
- A particle of mass m and charge q>0 is projected from infinity towards a nucleus of charge Q.
- The initial kinetic energy of the particle is K.
- At infinity, the potential energy is zero, and the total energy is purely kinetic, given by K.
- As the particle approaches the nucleus, it slows down due to the electrostatic force and will eventually come to rest at some distance R from the nucleus.
- When the particle comes to rest at distance R, all its initial kinetic energy K is converted into electrostatic potential energy U.
- The electrostatic potential energy U between two point charges is given by:
U=14πϵ0QqR
- According to the conservation of energy:
K=U
- Substituting the expression for potential energy:
K=14πϵ0QqR
- Rearranging the equation to solve for R:
R=14πϵ0QqK
- Thus, the distance R at which the particle comes to rest is given by:
R=Qq4πϵ0K
The closest distance to the center of the nucleus when the particle comes instantaneously to rest is:
R=Qq4πϵ0K
Topper's Solved these Questions
- ELECTROSTATICSBOOK - RESONANCE ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Exercise-1 Section (E)
2 Videos
- ELECTROSTATICSBOOK - RESONANCE ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Exercise-1 Section (F)
4 Videos
- ELECTROSTATICSBOOK - RESONANCE ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Exercise-1 Section (C)
14 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - A.l.P
19 Videos
- EXPERIMENTAL PHYSICSBOOK - RESONANCE ENGLISHCHAPTER - EXPERIMENTAL PHYSICSEXERCISE - PART -II
10 Videos
Similar Questions
A particle of mass m, charge q and kinetic energy T enters in a transverse uniform magnetic field of induction B. After the 3 s, the kinetic energy of the particle will be
View SolutionA particle of mass m carrying charge q is projected with velocity v from point P toward aninfinite line of charge from a distance a . Its speed reduces to zero momentarily at point Q, which is at a distance a/2 from the line of charge. If another particle with mass m and charge −q is projected with the same velosity v from P toward the line of charge, what will be its speed at Q ?
View SolutionA particle of charges Q and mass m travels through a potential difference V from rest. The final momentum of the particle is
View SolutionA particle of mass m and positive charge q is projected with a speed of v0 in y–direction in the presence of electric and magnetic field are in x–direction. Find the instant of time at which the speed of particle becomes double the initial speed.
View SolutionA particle of mass m and charge q is projected into a region having a perpendicular magnetic field B. Find the angle of deviation fo the particle as it comes out of the magnetic field if the width d of the regions is very smaller then

View SolutionA particle of mass m and charge q is projected into a region having a perpendicular magnetic field B. Find the angle of deviation fo the particle as it comes out of the magnetic field if the width d of the regions is very smaller then

View SolutionRutherford model: The approximate size of the nucleus can be calculated by using energy conservation theorem in Rutherford's α-scattering experiment. If an α-particle is projected from infinity with speed v towards the nucleus having Z protons, then the α-particle which is reflected back or which is deflected by 180∘ must have approached closest to the nucleus .It can be approximated that α particle collides with the nucleus and gets back. Now if we apply the energy conservation equation at initial point and collision point then:
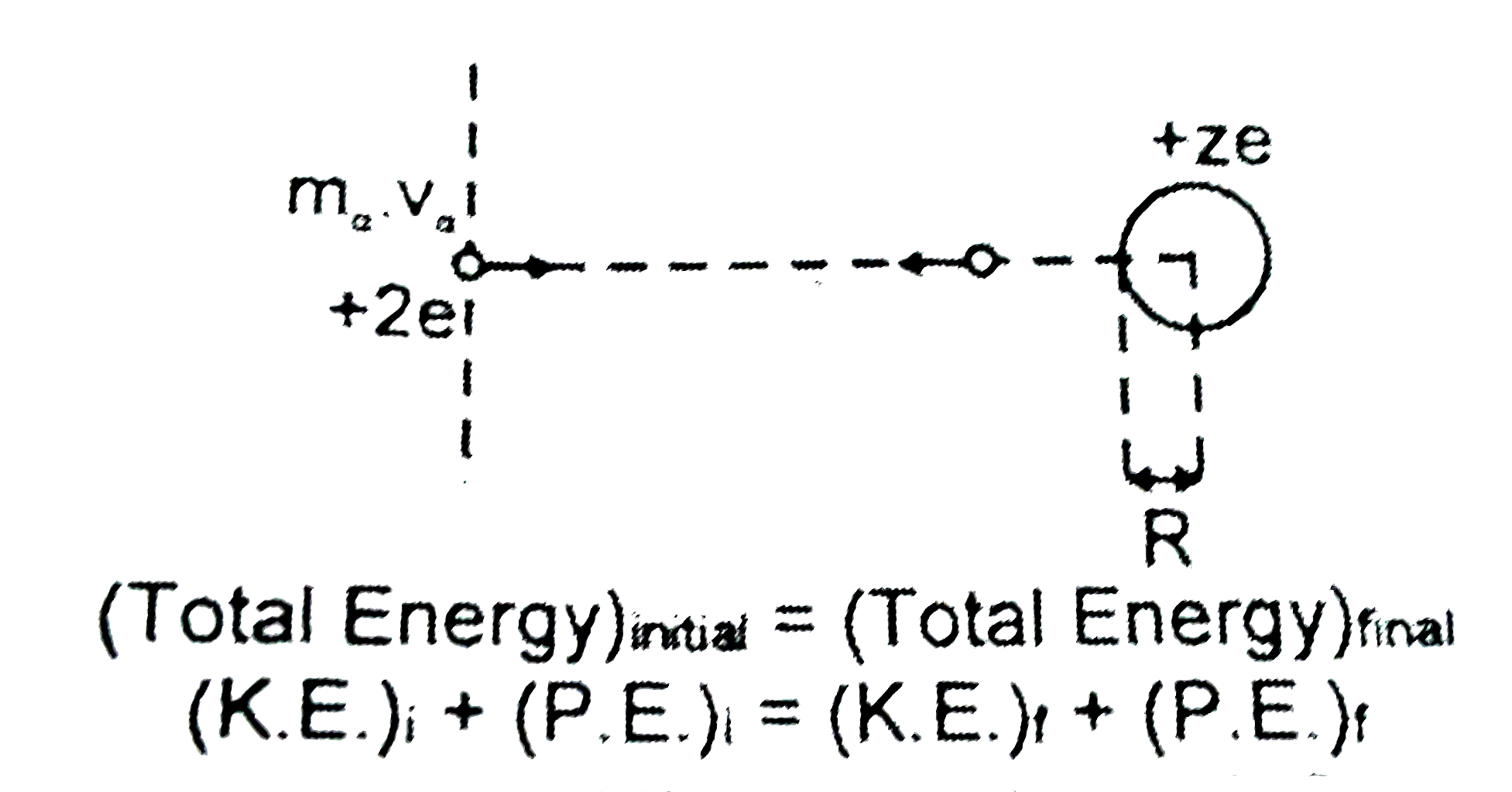
(P.E.)i=0, since P.E. of two charge system separated by infinite distance is zero. Finally the particle stops and then starts coming back.
12mαv2α+0=0+Kq1q2R⇒12mαv2α=K2e×ZeR⇒R=4KZe2mαv2α
Thus the radius of nucleus can be calculated using above equation. The nucleus is so small a particle that we can't define a sharp boundary for it
An α-particle with initial speed v0 is projected from infinity and it approaches up to r0 distance from a nuclie. Then, the initial speed of α-particle, which approaches upto 2r0 distance from the nucleus is :
View SolutionA particle P of charge q and m , is placed at a point in gravity free to move. Another particle Q, of same charge and mass , is projected from a distance r from P with an initial speed v0 towards P , initial the distance between P and Q decreases and then increases.
The potential energy of the system of particles P and Q, at closest separation is
View SolutionWhen an α particle of mass m moving with velocity v bombards on a heavy nucleus of charge Ze, its distance of closest approach form the nucleus depends on m as
View SolutionRutherford model: The approximate size of the nucleus can be calculated by using energy conservation theorem in Rutherford's α-scattering experiment. If an α-particle is projected from infinity with speed v towards the nucleus having Z protons, then the α-particle which is reflected back or which is deflected by 180∘ must have approached closest to the nucleus .It can be approximated that α particle collides with the nucleus and gets back. Now if we apply the energy conservation equation at initial point and collision point then:
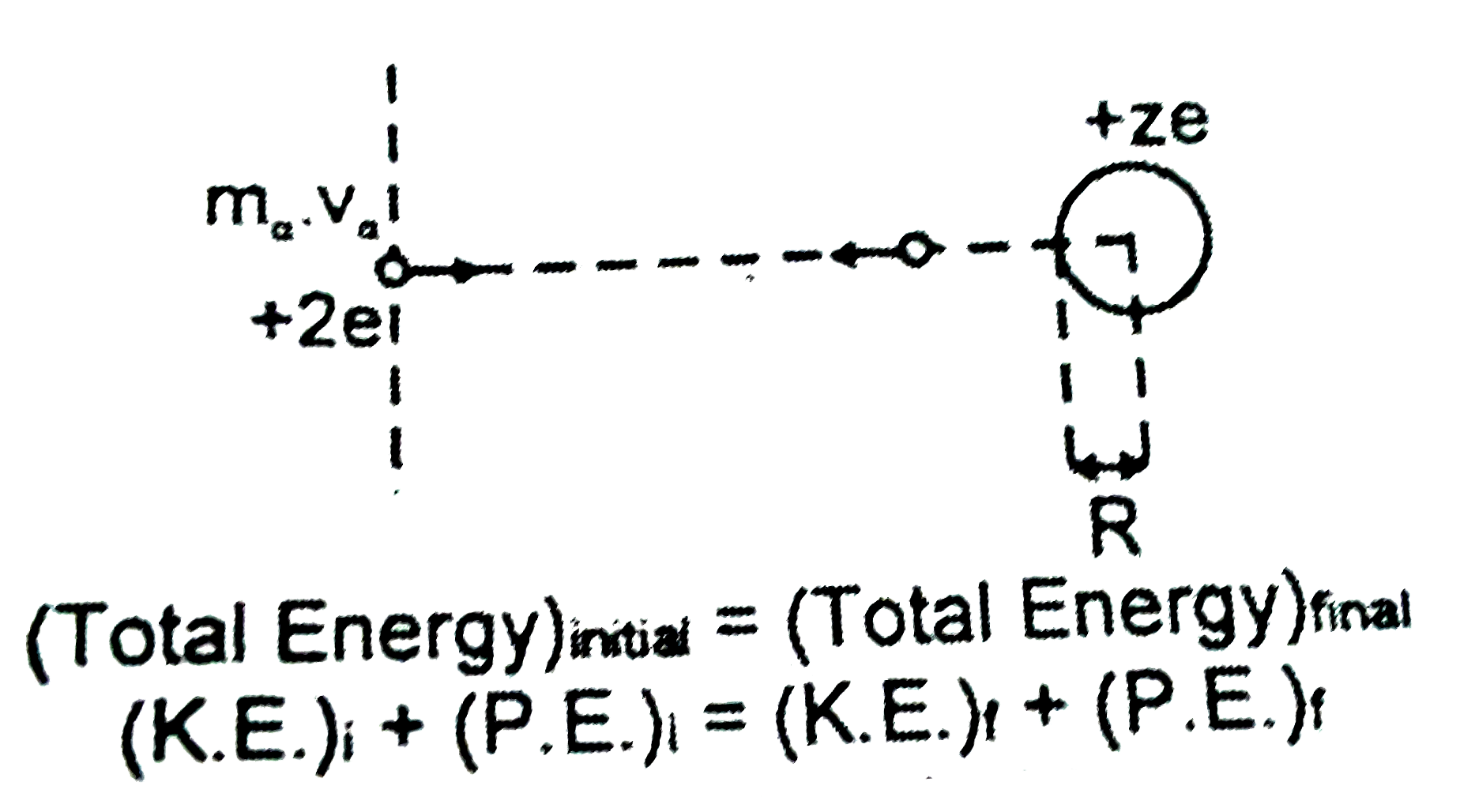
(P.E.)i=0, since P.E. of two charge system separated by infinite distance is zero. Finally the particle stops and then starts coming back.
12mαv2α+0=0+Kq1q2R⇒12mαv2α=K2e×ZeR⇒R=4KZe2mαv2α
Thus the radius of nucleus can be calculated using above equation. The nucleus is so small a particle that we can't define a sharp boundary for it
Radius of a particular nucleus is calculated by the projection of α-particle from infinity at a particular speed. Let this radius is the true radius . If the radius calculation for the same nucleus is made by another α-particle with half of the earlier speed, then the percentage error involved in the radius calculation is :
View Solution
