Coefficient of thermal conductivity has the dimensions:
[MLT−3K−1]
[ML3T3K2]
[ML3T−3K−2]
[M2L3T−3K2]
The correct Answer is:A
To determine the dimensions of the coefficient of thermal conductivity (k), we will start from the formula for the rate of heat flow through a material.
1. Understanding the Formula:
The rate of heat flow (Q) through a slab is given by the formula:
Q=k⋅A⋅Δθd⋅t
where:
- Q = rate of heat flow
- k = coefficient of thermal conductivity
- A = area through which heat is flowing
- Δθ = temperature difference
- d = thickness of the slab
- t = time
2. Identifying Dimensions:
- The dimension of heat flow Q is energy per unit time. The dimension of energy (work) is ML2T−2, so:
(Q)=ML2T−2
- The dimension of area A is:
(A)=L2
- The dimension of temperature difference Δθ is:
(Δθ)=K(Kelvin)
- The dimension of thickness d is:
(d)=L
- The dimension of time t is:
(t)=T
3. Rearranging the Formula:
We can rearrange the formula to solve for k:
k=Q⋅dA⋅Δθ⋅t
4. Substituting Dimensions:
Now, substituting the dimensions we identified:
(k)=(Q)⋅(d)(A)⋅(Δθ)⋅(t)
Substituting the dimensions:
(k)=(ML2T−2)⋅LL2⋅K⋅T
5. Simplifying the Expression:
Now, simplify the expression:
(k)=ML3T−2L2KT=ML3L2KT3=ML⋅K⋅T
Therefore, we can write:
(k)=ML−1T−3K−1
6. Final Result:
The dimensions of the coefficient of thermal conductivity k are:
(k)=MLT−3K−1
The rate of heat flow (Q) through a slab is given by the formula:
Q=k⋅A⋅Δθd⋅t
where:
- Q = rate of heat flow
- k = coefficient of thermal conductivity
- A = area through which heat is flowing
- Δθ = temperature difference
- d = thickness of the slab
- t = time
- The dimension of heat flow Q is energy per unit time. The dimension of energy (work) is ML2T−2, so:
(Q)=ML2T−2
- The dimension of area A is:
(A)=L2
- The dimension of temperature difference Δθ is:
(Δθ)=K(Kelvin)
- The dimension of thickness d is:
(d)=L
- The dimension of time t is:
(t)=T
We can rearrange the formula to solve for k:
k=Q⋅dA⋅Δθ⋅t
Now, substituting the dimensions we identified:
(k)=(Q)⋅(d)(A)⋅(Δθ)⋅(t)
Substituting the dimensions:
(k)=(ML2T−2)⋅LL2⋅K⋅T
Now, simplify the expression:
(k)=ML3T−2L2KT=ML3L2KT3=ML⋅K⋅T
Therefore, we can write:
(k)=ML−1T−3K−1
The dimensions of the coefficient of thermal conductivity k are:
(k)=MLT−3K−1
Topper's Solved these Questions
Similar Questions
The unit of thermal conductivity is :
View SolutionThe unit of thermal conductivity is :
View SolutionThe dimensions of coefficient of thermal conductivity is
View SolutionCoefficient of thermal conductivity is the product of heat, distance and reciprocal of (area x difference in temperature x time). The new value of a unit of coefficient of thermal conductivity, if fundamental units are 21.6 kg, 1 decimetre, 4 K and 1 minute will be ______ ×10−6 new units.
View SolutionTwo vessels of different materials are identical in size and wall-thickness. They are filled with equal quantities of ice at 0∘C. If the ice melts completely in 10 and 25 minutes respectively, compare the coefficients of thermal conductivity of the materials of the vessels.
View SolutionDefine coefficient of thermal conductivity or thermal conductivity of a substance.
View SolutionTwo bar of identical dimensions are kept as shown in figure. If K1 and K2are their coefficients of thermal conductivities, then equivalent K
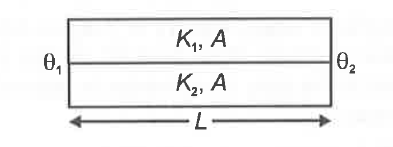 View Solution
View Solution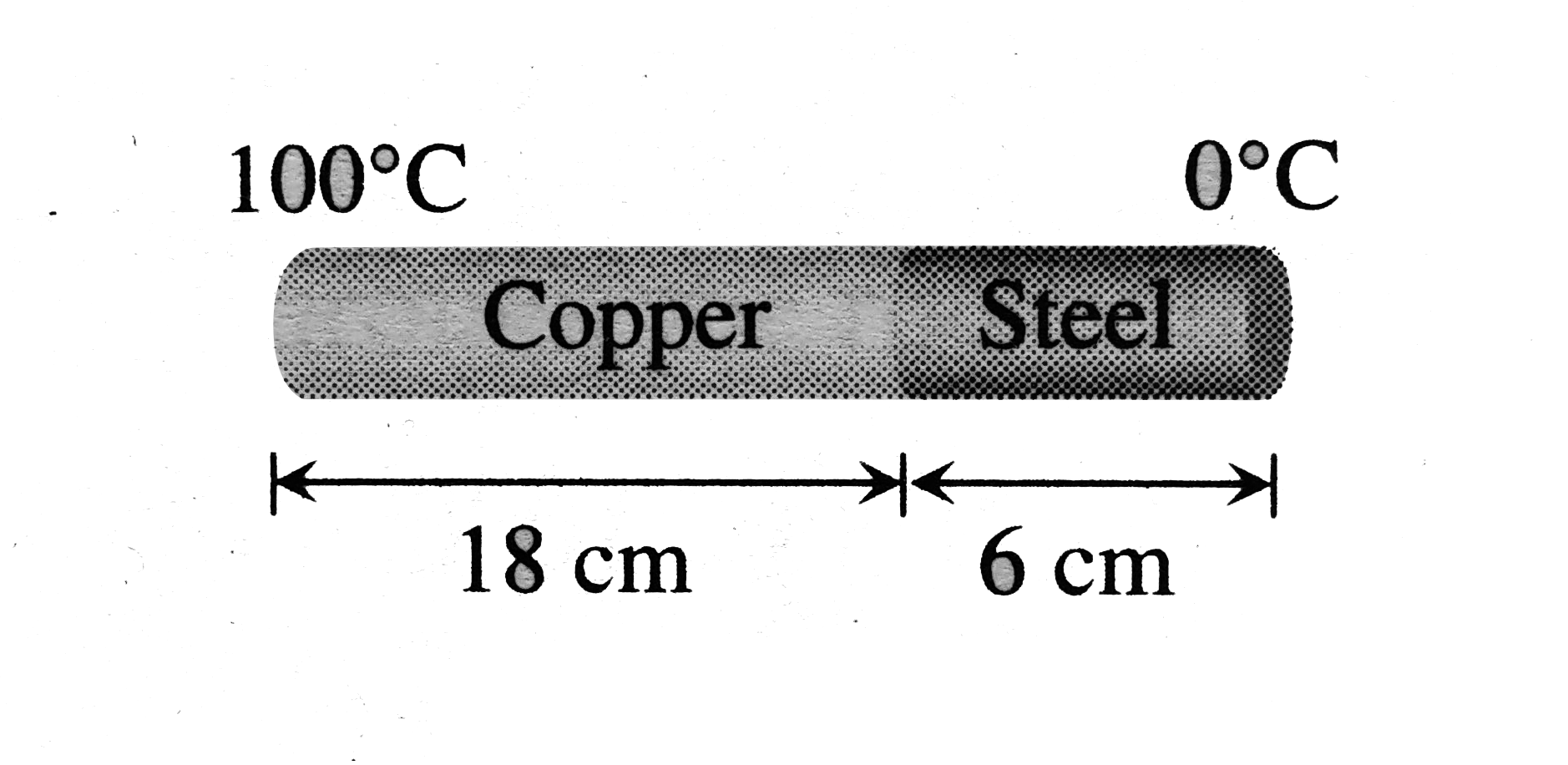
The coefficient of thermal conductivity of copper is nine times that of steel. In the composite cylindrical bar shown in Fig. what will be the temperature at the junction of copper and steel ?View SolutionA thermocole cubical icebox of side 30 cm has a thickness of 5.0 cm if 4.0 kg of ice are put ini the box, estimate the amount of ice remaining after 6 h. The outside temperature is 45∘C and coefficient of thermal conductivity of thermocole=0.01J/kg.
View SolutionObtain the dimensions of (1) coefficient of thermal conductivity (2) Gas constant (3) Boltzmann constant (4) Planck's constant.
View Solution

