A transverse wave travels on a taut steel wire with a velocity of when tension in it is 2.06×104N . When the tension is changed to T, the velocity changed to 3v. The value of T is close to :
10.2×102N
5.15×103N
2.50×104N
18.54×104N
The correct Answer is:D
To solve the problem, we need to understand the relationship between the velocity of a wave on a string and the tension in the string. The formula that relates these quantities is:
v=√Tμ
where:
- v is the velocity of the wave,
- T is the tension in the string,
- μ is the linear mass density of the string.
From this formula, we can derive that the square of the velocity is proportional to the tension:
v2∝T
This means that if we change the velocity, the tension will change according to the square of the velocity.
1. Initial Conditions:
- The initial tension is T1=2.06×104N.
- The initial velocity is v.
2. Final Conditions:
- The final velocity is v2=3v.
3. Using the Proportionality:
- Since v2∝T, we can write:
T2T1=(v2v1)2
- Substituting the values:
T2T1=(3vv)2=32=9
4. Calculating the New Tension:
- Rearranging gives:
T2=9T1
- Substituting the value of T1:
T2=9×(2.06×104N)=18.54×104N
5. Final Answer:
- Therefore, the value of T is approximately 1.854×105N.
- v is the velocity of the wave,
- T is the tension in the string,
- μ is the linear mass density of the string.
- The initial tension is T1=2.06×104N.
- The initial velocity is v.
- The final velocity is v2=3v.
- Since v2∝T, we can write:
T2T1=(v2v1)2
- Substituting the values:
T2T1=(3vv)2=32=9
- Rearranging gives:
T2=9T1
- Substituting the value of T1:
T2=9×(2.06×104N)=18.54×104N
- Therefore, the value of T is approximately 1.854×105N.
Topper's Solved these Questions
- JEE MAIN REVISION TEST - 27 - JEE -2020BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN REVISION TEST - 27 - JEE -2020EXERCISE - PHYSICS (SECTION 2)
5 Videos
- JEE MAIN REVISION TEST - 26 (2020)BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN REVISION TEST - 26 (2020)EXERCISE - PHYSICS (SECTION 2)
5 Videos
- JEE MAIN REVISION TEST - 28BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN REVISION TEST - 28EXERCISE - PHYSICS (SECTION-2)
5 Videos
Similar Questions
Velocity of a wave in a wire is v when tension in it is 2.06×104N. Find value of tension in wire when velocity of wave become v2.
View SolutionVelocity of a wave in a wire is v when tension in it is 2.06×104N. Find value of tension in wire when velocity of wave become v2.
View SolutionThe length of a metal wire is l1 when the tension in it is T1 and is l2 when the tension is T2. The natural length of the wire is
View SolutionThe length of a metal wire is l1 when the tension in it is T1 and is l2 when the tension is T2. The natural length of the wire is
View SolutionThe length of a metal wire is l1 when the tensionin it is T1andisl2 when the tension is T2. The natural length of the wire is
View SolutionThe length of a metal wire is l1 when the tension in it is T1 and is l2 when the tension is T2. Then natural length of the wire is
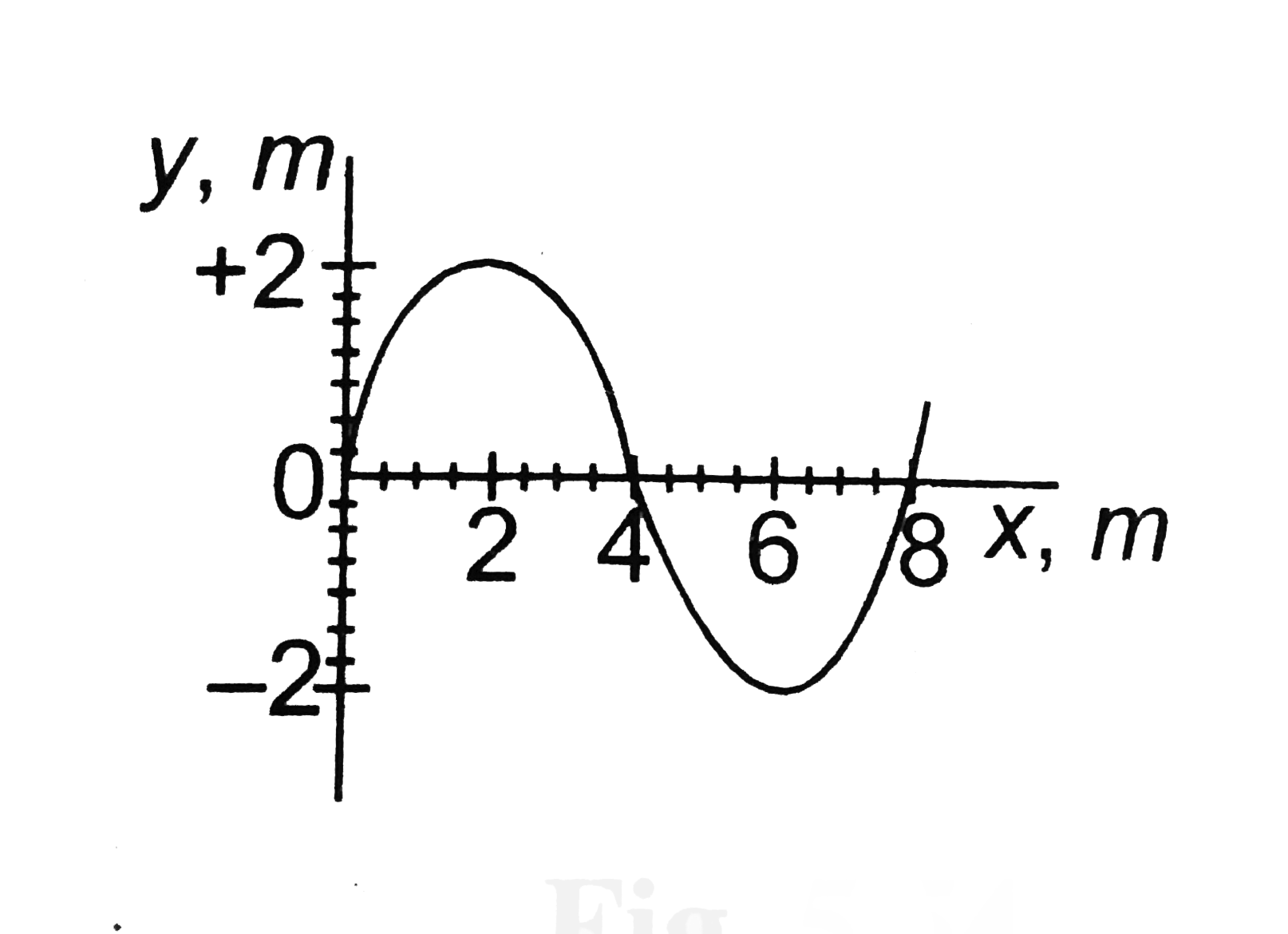
View SolutionThe graph shows a wave at t=0 travelling to the right with a velocity of 4m/s. The equation that best represents the wave is
View SolutionA transverse wave travelling on a taut string is represented by:
Y=0.01sin2π(10t−x)
Y and x are in meters and t in seconds. Then,
View SolutionA string of length 2x is stretched by 0.1 x and the velocity of a transverse wave along it is v. When it is stretched by 0.4x , the velocity of the wave is
View Solutionan elastic spring has a length l1 when tension in it is 4N. Its length is l2 when tension in it is 5N. What will be its length when tension in it is 9N?
View Solution
