If C is the middle point of AB and P is any point outside AB, then
−−→PA+−−→PB+−−→PC=0
−−→PA+−−→PB+2−−→PC=0
−−→PA+−−→PB+−−→PC
−−→PA+−−→PB=2−−→PC
The correct Answer is:D
Step by step video & image solution for If C is the middle point of AB and P is any point outside AB, then by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- VECTORS BOOK - VMC MODULES ENGLISHCHAPTER - VECTORS EXERCISE - JEE ADVANCED (ARCHIVE)
36 Videos
- VECTORS BOOK - VMC MODULES ENGLISHCHAPTER - VECTORS EXERCISE - JEE ADVANCED (ARCHIVE) (INTEGER TYPE)
4 Videos
- VECTORS BOOK - VMC MODULES ENGLISHCHAPTER - VECTORS EXERCISE - Numerical ValueType for JEE Main
15 Videos
- TRIGONOMETRIC IDENTITIES AND EQUATIONS BOOK - VMC MODULES ENGLISHCHAPTER - TRIGONOMETRIC IDENTITIES AND EQUATIONS EXERCISE - JEE Advanced (Archive)
11 Videos
Similar Questions
If C is the mid point of AB and P is any point outside AB then (A) −−→PA+−−→PB+−−→PC=0 (B) −−→PA+−−→PB+2−−→PC=→0 (C) −−→PA+−−→PB=−−→PC (D) −−→PA+−−→PB=2−−→PC
View SolutionIf C is the mid point of AB and P is any point outside AB then (A) −−→PA+−−→PB+−−→PC=0 (B) −−→PA+−−→PB+2−−→PC=→0 (C) −−→PA+−−→PB=−−→PC (D) −−→PA+−−→PB=2−−→PC
View SolutionIf C is the mid point of AB and P is any point outside AB then (A) −−→PA+−−→PB+−−→PC=0 (B) −−→PA+−−→PB+2−−→PC=→0 (C) −−→PA+−−→PB=−−→PC (D) −−→PA+−−→PB=2−−→PC
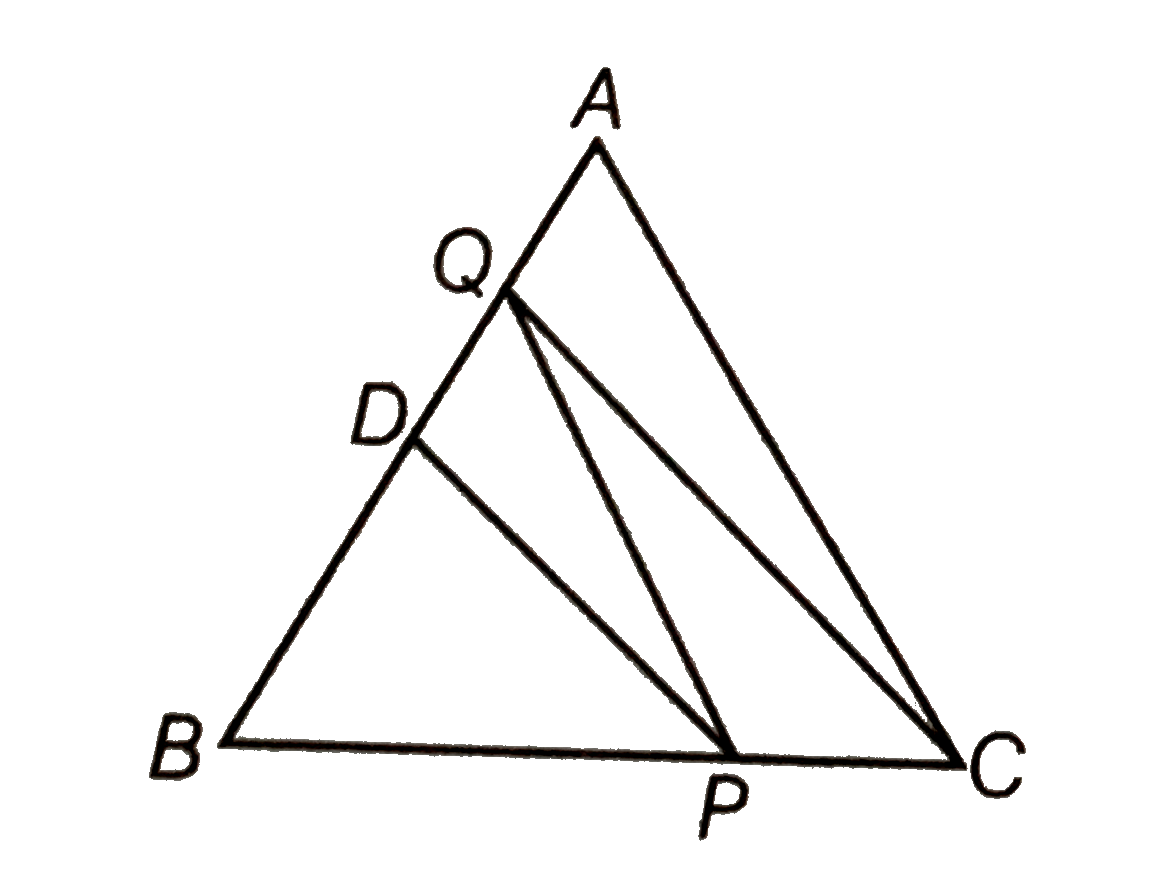
View SolutionIn Δ ABC, D is the mid-point of AB and P is any point on BC. If CQ∣∣PD meets AB and Q (shown in figure), then prove that
ar(ΔBPQ)=12ar(ΔABC).
View SolutionAB is a vertical pole. The end A is on the level ground .C is the middle point of AB. P is a point on the level ground . The portion BC subtends an angles β at P. If AP = nAB, then tan β=
View SolutionAB is a vertical pole. The end A is on the level ground .C is the middle point of AB. P is a point on the level ground . The portion BC subtends an angles β at P. If AP = nAB, then tan β=
View SolutionIn triangle ABC, D is mid-point of AB and P is any point on BC. If CQ parallel to PD meets AB at Q, prove that: 2× area (ΔBPQ)= area (ΔABC)
View SolutionIn the adjoining figure
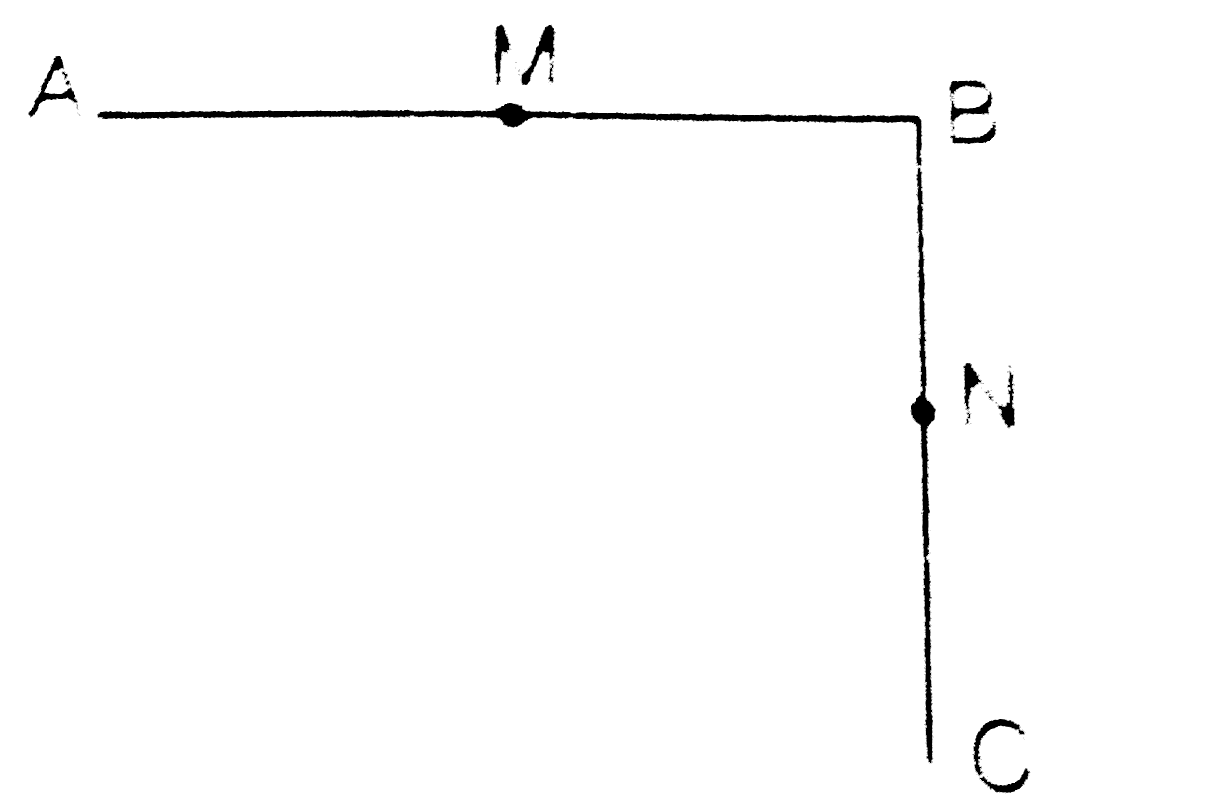
(i) AB=BC, M is the mid -point of AB and N is the mid- point of BC. Show that AM=NC.
(ii) BM=BN,M is the mid- point of AB and N is the mid - point of BC. Show that AB=BC.
View SolutionAB is vertical tower. The point A is on the ground and C is the middle point of AB. The part CB subtend an angle α at a point P on the ground. If AP=nAB, then tanα=
View SolutionABC is a triangle in which AB = AC and D is any point on BC. Prove that:
AB2−AD2=BD.CD
View Solution
