The maximum and minimum magnitude of the resultant of two given vectors are 17 units and 7 units respectively. If these tow vectors are at right angles to each other, the magnitude of their resultant is
14
16
18
13
The correct Answer is:d
a+b =17, a-b =7 a=12 , b =5
magnitude of and if they are r is
Topper's Solved these Questions
- BOOK - VMC MODULES ENGLISHCHAPTER - INTRODUCTION TO VECTORS & FORCES EXERCISE - JEE Main ( ARCHIVE ) ( LEVEL-1)12videos
INTRODUCTION TO VECTORS & FORCES
- BOOK - VMC MODULES ENGLISHCHAPTER - INTRODUCTION TO VECTORS & FORCES EXERCISE - JEE Advanced ( ARCHIVE LEVEL-2)12videos
INTRODUCTION TO VECTORS & FORCES
- BOOK - VMC MODULES ENGLISHCHAPTER - INTRODUCTION TO VECTORS & FORCES EXERCISE - level 175videos
INTRODUCTION TO VECTORS & FORCES
- BOOK - VMC MODULES ENGLISHCHAPTER - GRAVITATIONEXERCISE - JEE Advance (Archive) TRUE/FALSE1videos
GRAVITATION
- BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN - 5EXERCISE - PART I : PHYSICS (SECTION - 2)5videos
JEE MAIN - 5
Similar Questions
The maximum and minimum magnitude of the resultant of two given vectors are 17 units and 7 unit respectively. If these two vectors are at right angles to each other, the magnitude of their resultant is
View SolutionThe maximum and minimum magnitudes of the resultant of two vectors are 23 units and 7 units respectively. If these two vectors are acting at right angles to each other, the magnitude of their resultant will be
View Solution
Knowledge Check
- Question 1 - Select One
If the sum of two unit vectors is a unit vector, then the magnitude of their difference is :
A√3B√2C√5D1√2
The maximum and minimum magnitudes of the resultant of two vectors are 23 units and 7 units respectively. If these two vectors are acting at right angles to each other, the magnitude of their resultant will be
View SolutionThe maximum and minimum magnitudes of the resultant of two forces are 5 N and 5N respectively. Find the magnitude of resultant force when act orthogonally to each other.
View SolutionFind the magnitude and direction of the resultant of given vectors
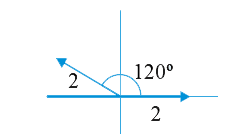 View Solution
View SolutionIf three unit vectors are inclined at an angle of 60∘ with each other, then the magnitude of their resultant vector will be
View SolutionThe ratio of maximum and minimum magnitudes of the resultant of two vectors →a and →b is 3:1. Now, |→a| is equal to
View SolutionThe ratio of maximum and minimum magnitudes of the resultant of two vectors →a and →b is 3:1. Now, |→a| is equal to
View SolutionThe ratio of maximum and minimum magnitude of the resultant of two vectors →A and →B is 3:2. The relation between A and B is
View Solution

