With what minimum speed must a particle be projected from origin so that it is able to pass through a given point (30m,40m) ? Take g=10m/s2
60 m/s
30 m/s
50 m/s
40 m/s
The correct Answer is:B
To solve the problem of determining the minimum speed at which a particle must be projected from the origin to pass through the point (30m, 40m), we can follow these steps:
Step 1: Understand the trajectory equation
The trajectory of a projectile can be described by the equation:
y=xtanθ−g2x2u2cos2θ
where:
- y is the height,
- x is the horizontal distance,
- g is the acceleration due to gravity,
- u is the initial speed,
- θ is the angle of projection.
Step 2: Substitute the known values
We need to find the minimum speed u such that the projectile passes through the point (30m, 40m). Here, x=30 m and y=40 m. We also know g=10m/s2.
Substituting these values into the trajectory equation gives:
40=30tanθ−102302u2cos2θ
This simplifies to:
40=30tanθ−1500u2cos2θ
Step 3: Use trigonometric identities
Using the identity sec2θ=1+tan2θ, we can express cos2θ in terms of tanθ:
cos2θ=11+tan2θ
Let z=tanθ. Then, we can rewrite the equation as:
40=30z−1500(1+z2)u2
Step 4: Rearranging the equation
Rearranging gives:
40u2=30zu2−1500(1+z2)
This can be rearranged to:
40u2−30zu2+1500+1500z2=0
This is a quadratic equation in terms of u2.
Step 5: Solve for u2
Using the quadratic formula u2=−b±√b2−4ac2a, where:
- a=40,
- b=−30z,
- c=1500+1500z2.
The discriminant D must be non-negative for real solutions:
D=(−30z)2−4⋅40⋅(1500+1500z2)
D=900z2−240000−240000z2
D=−239100z2−240000
Step 6: Set the discriminant to zero for minimum speed
To find the minimum speed, set D=0:
900z2−240000−240000z2=0
This leads to solving for z.
Step 7: Solve for z
Solving the quadratic equation will yield the values for z. After determining z, substitute back to find u2.
Step 8: Calculate u
Finally, substitute the value of z back into the equation for u2 to find the minimum speed u.
Final Result
After performing the calculations, we find that the minimum speed u required is:
u=30m/s
The trajectory of a projectile can be described by the equation:
y=xtanθ−g2x2u2cos2θ
where:
- y is the height,
- x is the horizontal distance,
- g is the acceleration due to gravity,
- u is the initial speed,
- θ is the angle of projection.
We need to find the minimum speed u such that the projectile passes through the point (30m, 40m). Here, x=30 m and y=40 m. We also know g=10m/s2.
40=30tanθ−102302u2cos2θ
This simplifies to:
40=30tanθ−1500u2cos2θ
Using the identity sec2θ=1+tan2θ, we can express cos2θ in terms of tanθ:
cos2θ=11+tan2θ
Let z=tanθ. Then, we can rewrite the equation as:
40=30z−1500(1+z2)u2
Rearranging gives:
40u2=30zu2−1500(1+z2)
This can be rearranged to:
40u2−30zu2+1500+1500z2=0
This is a quadratic equation in terms of u2.
Using the quadratic formula u2=−b±√b2−4ac2a, where:
- a=40,
- b=−30z,
- c=1500+1500z2.
D=(−30z)2−4⋅40⋅(1500+1500z2)
D=900z2−240000−240000z2
D=−239100z2−240000
To find the minimum speed, set D=0:
900z2−240000−240000z2=0
This leads to solving for z.
Solving the quadratic equation will yield the values for z. After determining z, substitute back to find u2.
Finally, substitute the value of z back into the equation for u2 to find the minimum speed u.
After performing the calculations, we find that the minimum speed u required is:
u=30m/s
Topper's Solved these Questions
- Motion in Two DimensionsBOOK - VMC MODULES ENGLISHCHAPTER - Motion in Two DimensionsEXERCISE - JEE Main (Archive)
19 Videos
- Motion in Two DimensionsBOOK - VMC MODULES ENGLISHCHAPTER - Motion in Two DimensionsEXERCISE - JEE Advanced (Archive)
19 Videos
- Motion in Two DimensionsBOOK - VMC MODULES ENGLISHCHAPTER - Motion in Two DimensionsEXERCISE - Long Answer Type
5 Videos
- Motion in Straight Line BOOK - VMC MODULES ENGLISHCHAPTER - Motion in Straight Line EXERCISE - IN-CHAPTER EXERCISE-J
10 Videos
- MOVING CHARGES & MAGNETISM BOOK - VMC MODULES ENGLISHCHAPTER - MOVING CHARGES & MAGNETISM EXERCISE - IN-CHAPTER EXERCISE-K
10 Videos
Similar Questions
A body is projected from the ground with some speed at some angle with the horizontal. Taking the horizontal and vertical direction to be x and y axis respectively and the point of projection as origin, calculate the minimum speed (in ms−1) of projection so that it can pass through a point whose x and y coordinates are 30 m and 40 m respectively? Take g=10ms−2
View SolutionTrack OABCD (as shown in figure ) is smooth. What minimum speed has to be given to a particle lying at point A, so that it can reach point C? (take g=10m/s2)
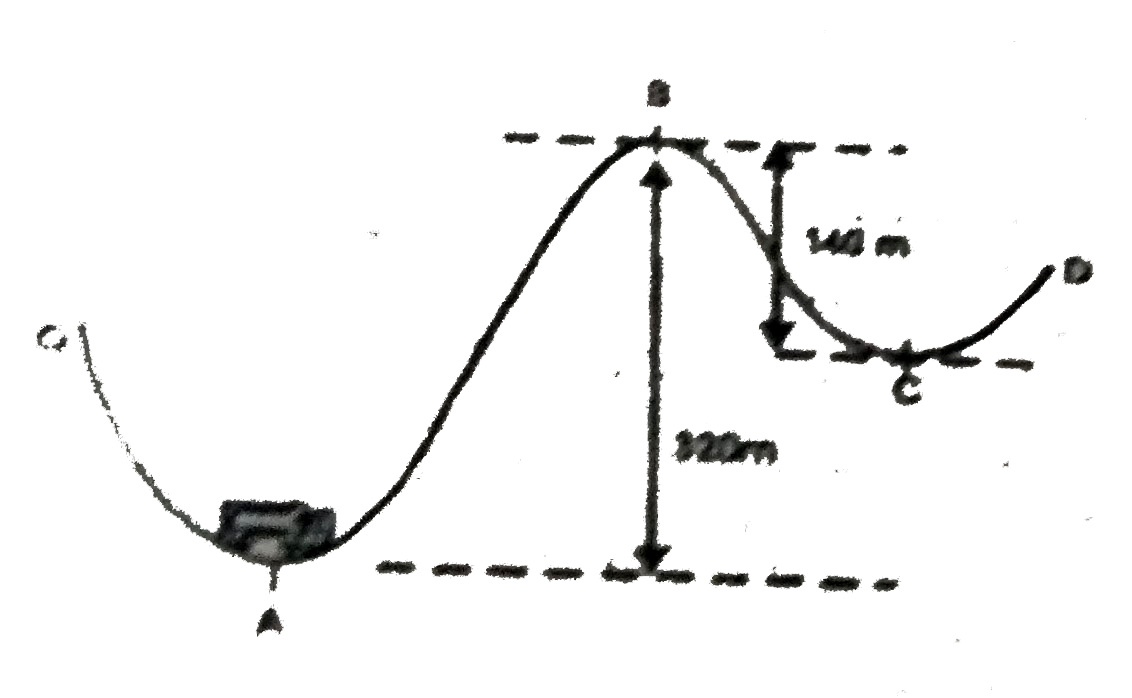
View SolutionA ball is projected vertically up with speed 20 m/s. Take g=10m/s2
View SolutionA particle is projected with velocity of 10ms at an angle of 15° with horizontal.The horizontal range will be(g=10ms2)
View SolutionA particle is projected from ground with velocity 20(√2)m/s at 45∘. At what time particle is at height 15 m from ground? (g=10m/s2)
View SolutionA particle is projected from ground with velocity 40√2m/s at 45∘. At time t=2s
View SolutionMatch the following

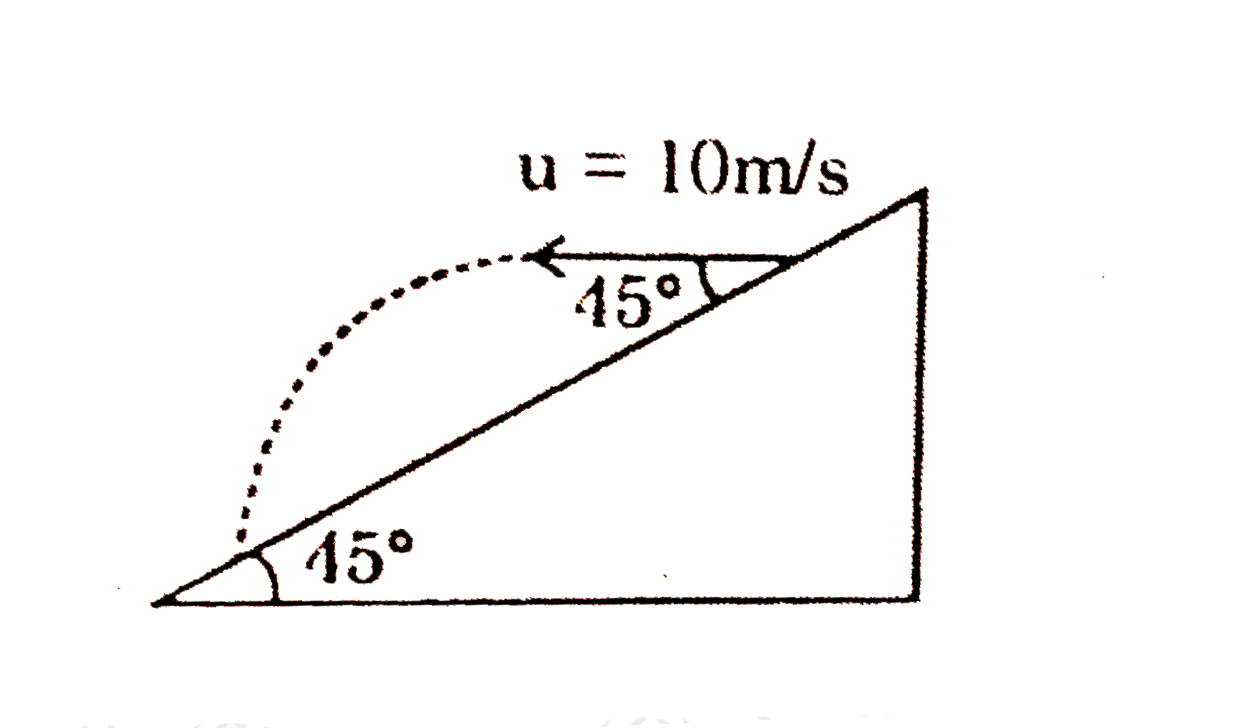
A particle is projected from a large fixed incline plane as shown. Here (Vertically downward) take g=10m/s2.
View SolutionA particle is thrown vertically up from the top of a building of height 20m with initial velocity u. A second particle is released from the same point 1 second later. If both particles reach the ground at the same instant ,3u=m/s. [Takeg=10m/s2]
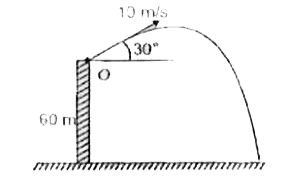
View SolutionA ball is projected from a point O as shown in figure it will strike the ground after (g = 10 m/s2 )
View SolutionA particle A is projected from the ground with an initial velocity of 10m/s at an angle of 60∘ with horizontal. From what height should an another particle B be projected horizontally with velocity 5m/s so that both the particles collide in ground at point C if both are projected simultaneously g=10m/s2.

View Solution