Question

If a body moving in circular path maintains constant speed of 10 ${\displaystyle m{s}^{–1}}$, then which of the following correctly describes relation between acceleration and radius ?

Options

A.
B.
C.
D.

Answer

A

Explanation

To solve the problem, we need to determine the relationship between acceleration and radius for a body moving in a circular path with a constant speed. Let's break it down step by step.

Step 1: Understand the types of acceleration in circular motion
In circular motion, there are two types of acceleration to consider:
1. Tangential Acceleration (a_t): This is related to the change in the speed of the object along the circular path. Since the speed is constant (10 m/s), the tangential acceleration is zero.
2. Centripetal Acceleration (a_c): This is the acceleration directed towards the center of the circular path, which keeps the object moving in a circle. It is given by the formula:
\( a_c = \frac{v^2}{r} \)
where \(v\) is the constant speed and \(r\) is the radius of the circular path.

Step 2: Substitute the known values
Since the speed \(v\) is constant at 10 m/s, we can substitute this value into the centripetal acceleration formula:
\( a_c = \frac{(10 \, \text{m/s})^2}{r} = \frac{100}{r} \)

Step 3: Analyze the relationship between acceleration and radius
From the equation \(a_c = \frac{100}{r}\), we can see that:
- As the radius \(r\) increases, the centripetal acceleration \(a_c\) decreases.
- Conversely, as the radius \(r\) decreases, the centripetal acceleration \(a_c\) increases.

This indicates that the centripetal acceleration is inversely proportional to the radius:
\( a_c \propto \frac{1}{r} \)

Step 4: Graphical representation
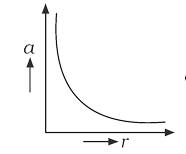
The relationship \(a_c \propto \frac{1}{r}\) suggests that if we plot acceleration on the y-axis and radius on the x-axis, the graph will be a hyperbola. Specifically, as \(r\) decreases, \(a_c\) increases, and as \(r\) increases, \(a_c\) decreases.

Conclusion
Thus, the correct description of the relationship between acceleration and radius for a body moving in a circular path with a constant speed of 10 m/s is that acceleration is inversely proportional to the radius, which can be represented graphically as a hyperbola.

Final Answer
The correct option that describes the relationship between acceleration and radius is Option A, which represents a hyperbola.

---