What is the angle between frictional force and instantaneous velocity of the body moving over a rough surface ?
To determine the angle between the frictional force and the instantaneous velocity of a body moving over a rough surface, we can follow these steps:
Step 1: Understand the Motion of the Body
- When a body moves over a rough surface, it experiences friction. The direction of the body's instantaneous velocity is the direction in which it is moving at that moment.
Step 2: Identify the Direction of Frictional Force
- The frictional force always acts in the direction opposite to the motion of the body. This is a fundamental property of friction: it opposes the relative motion between surfaces in contact.
Step 3: Analyze the Angle Between Forces
- Given that the instantaneous velocity vector points in the direction of motion, and the frictional force vector points in the opposite direction, we can visualize this scenario.
- If we denote the direction of the instantaneous velocity as a vector pointing to the right, the frictional force vector will point to the left.
Step 4: Calculate the Angle
- The angle between two vectors that point in opposite directions is 180 degrees. Therefore, the angle between the frictional force and the instantaneous velocity of the body is:
Angle=180∘
Conclusion
- The angle between the frictional force and the instantaneous velocity of a body moving over a rough surface is always 180 degrees.
---
- When a body moves over a rough surface, it experiences friction. The direction of the body's instantaneous velocity is the direction in which it is moving at that moment.
- The frictional force always acts in the direction opposite to the motion of the body. This is a fundamental property of friction: it opposes the relative motion between surfaces in contact.
- Given that the instantaneous velocity vector points in the direction of motion, and the frictional force vector points in the opposite direction, we can visualize this scenario.
- If we denote the direction of the instantaneous velocity as a vector pointing to the right, the frictional force vector will point to the left.
- The angle between two vectors that point in opposite directions is 180 degrees. Therefore, the angle between the frictional force and the instantaneous velocity of the body is:
- The angle between the frictional force and the instantaneous velocity of a body moving over a rough surface is always 180 degrees.
Topper's Solved these Questions
- BOOK - VMC MODULES ENGLISHCHAPTER - DYNAMICS OF A PARTICLEEXERCISE - LEVEL 0 (SHORT ANSWER TYPE - I)7videos
DYNAMICS OF A PARTICLE
- BOOK - VMC MODULES ENGLISHCHAPTER - DYNAMICS OF A PARTICLEEXERCISE - LEVEL 0 (SHORT ANSWER TYPE - ii)11videos
DYNAMICS OF A PARTICLE
- BOOK - VMC MODULES ENGLISHCHAPTER - DC CIRCUITEXERCISE - JEE ADVANCED ARCHIVE68videos
DC CIRCUIT
- BOOK - VMC MODULES ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTEXERCISE - IN-CHAPTER EXERCISE-G10videos
ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENT
Similar Questions
Knowledge Check
- Question 1 - Select One
The frictional force between two surfaces is independent of
ANature of the surfaceBSize of the bodyCArea of contactDMass of the body
In a situation the contact force by a rough horizontal surface on a body placed on it has constant magnitude. If the angle between this force and the vertical is decreased, the frictional force between the surface and the body will
View SolutionA small body of mass m moving with velocity v0 on rough horizontal surface, finally stops due to friction. Find, the mean power developed by the friction force during the motion of the body, if the frictional coefficient μ=0.27,m=1 kg and v0=1.5ms−1.
View SolutionA weight W rests on a rough horizontal plane,If the angle of friction is θ,the least force that can move the body along the plane will be
View SolutionThe angle between the resultant contact force and the normal force exerted by a body on the other is called the angle of friction. Show that it λ be the angle of friction and μ the coefficient of static friction. λ≤tan−1μ.
View SolutionA block of mass m is attached with a massless spring of force constant K. the block is placed over a rough inclined surface for which the coefficient of friction is μ=34 find the minimum value of M required to move the block up the place. (Neglect mass of string and pulley. Ignore friction in pulley).
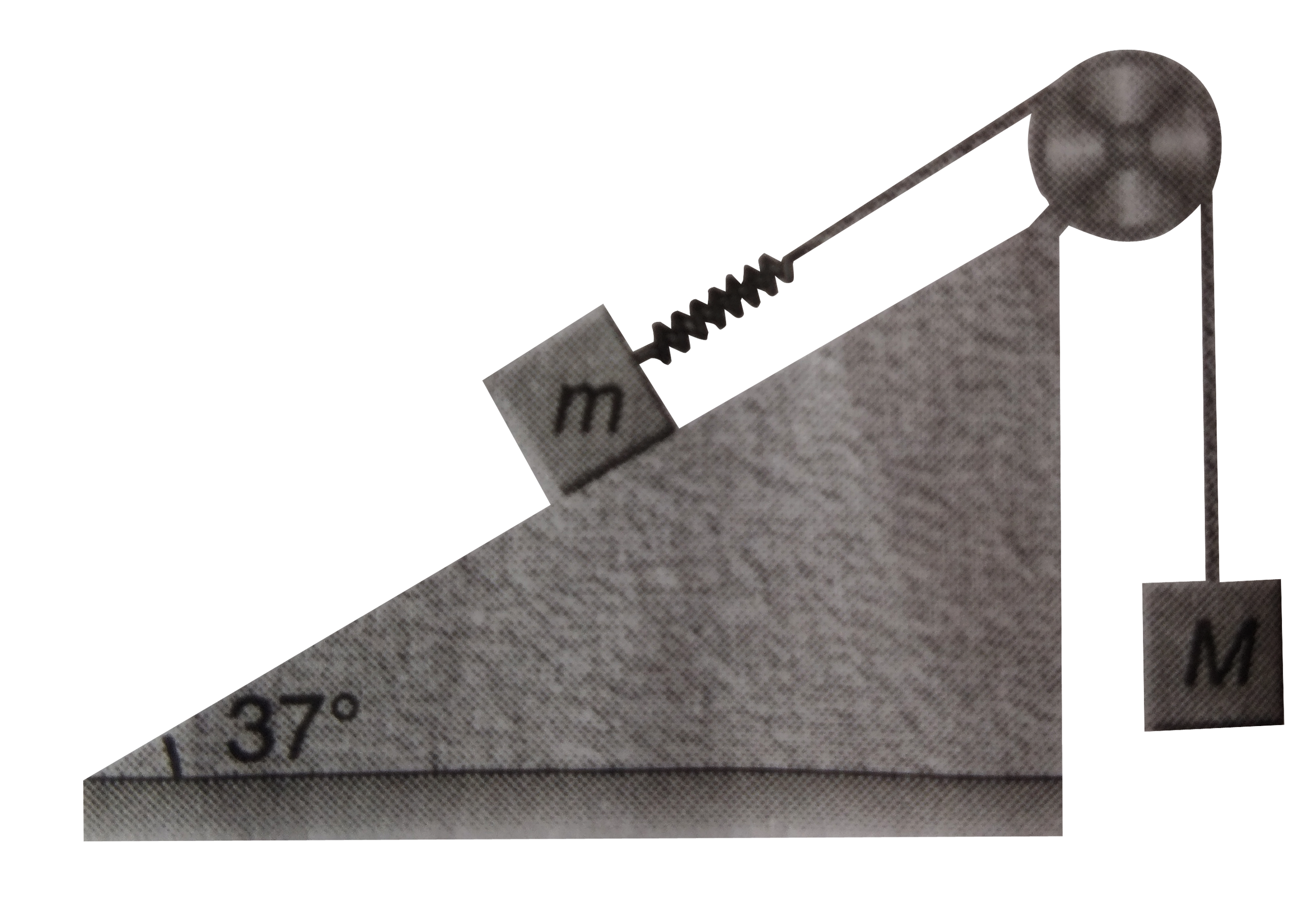 . View Solution
. View Solution(A) : When external force acting on a body moving on a rough horizontal surface is doubled, the acceleration of the body is doubled.
(R ) : The acceleration of a body moving on a rough horizontal surface is proportional to the resultant force acting on it.View SolutionA body is moving along a rough horizontal surface with an initial velocity 6m/s m If the body comes to rest after travelling 9 m , then the coefficient of sliding friction will be
View Solution

