At the top of a mountain a thermometer reads 70C and barometer reads 70cm of Hg. At the bottom of the mountain the barometer reads 76cm of Hg and thermometer reads 270C. The density of air at the top of mountain is "_" times the density at the bottom.
0.80
0.98
0.99
0.97
The correct Answer is:C
To find the ratio of the density of air at the top of the mountain to the density of air at the bottom, we can use the ideal gas law and the relationship between pressure, temperature, and density.
1. Understand the Ideal Gas Law:
The ideal gas law is given by the equation:
PV=nRT
where P is pressure, V is volume, n is the number of moles, R is the gas constant, and T is temperature.
2. Express Density in Terms of Pressure and Temperature:
We can express the number of moles n as:
n=mM
where m is the mass of the gas and M is the molar mass. Thus, we can rewrite the ideal gas law as:
PV=mMRT
Dividing both sides by V:
P=mV⋅RTM
Here, mV is the density ρ. Therefore, we can express density as:
P=ρ⋅RTM
Rearranging gives us:
ρ=PMRT
3. Set Up the Ratio of Densities:
We want to find the ratio of the density at the top of the mountain (ρt) to the density at the bottom of the mountain (ρb):
ρtρb=PtPb⋅TbTt
where Pt and Pb are the pressures at the top and bottom, and Tt and Tb are the temperatures at the top and bottom respectively.
4. Convert Temperatures to Kelvin:
- At the top: Tt=7∘C=7+273=280K
- At the bottom: Tb=27∘C=27+273=300K
5. Substitute the Given Values:
- Pressure at the top: Pt=70cmHg
- Pressure at the bottom: Pb=76cmHg
Now substituting these values into the density ratio:
ρtρb=7076⋅300280
6. Calculate the Ratio:
First, calculate 7076:
7076≈0.9211
Next, calculate 300280:
300280≈1.0714
Now multiply these two results:
ρtρb≈0.9211⋅1.0714≈0.987
7. Final Result:
The density of air at the top of the mountain is approximately 0.98 times the density at the bottom.
The ideal gas law is given by the equation:
PV=nRT
where P is pressure, V is volume, n is the number of moles, R is the gas constant, and T is temperature.
We can express the number of moles n as:
n=mM
where m is the mass of the gas and M is the molar mass. Thus, we can rewrite the ideal gas law as:
PV=mMRT
Dividing both sides by V:
P=mV⋅RTM
Here, mV is the density ρ. Therefore, we can express density as:
P=ρ⋅RTM
Rearranging gives us:
ρ=PMRT
We want to find the ratio of the density at the top of the mountain (ρt) to the density at the bottom of the mountain (ρb):
ρtρb=PtPb⋅TbTt
where Pt and Pb are the pressures at the top and bottom, and Tt and Tb are the temperatures at the top and bottom respectively.
- At the top: Tt=7∘C=7+273=280K
- At the bottom: Tb=27∘C=27+273=300K
- Pressure at the top: Pt=70cmHg
- Pressure at the bottom: Pb=76cmHg
ρtρb=7076⋅300280
First, calculate 7076:
7076≈0.9211
Next, calculate 300280:
300280≈1.0714
Now multiply these two results:
ρtρb≈0.9211⋅1.0714≈0.987
The density of air at the top of the mountain is approximately 0.98 times the density at the bottom.
Topper's Solved these Questions
- BOOK - VMC MODULES ENGLISHCHAPTER - GASEOUS STATE & THERMODYNAMICSEXERCISE - Level - 240videos
GASEOUS STATE & THERMODYNAMICS
- BOOK - VMC MODULES ENGLISHCHAPTER - GASEOUS STATE & THERMODYNAMICSEXERCISE - JEE MAIN (ARCHIVE )81videos
GASEOUS STATE & THERMODYNAMICS
- BOOK - VMC MODULES ENGLISHCHAPTER - GASEOUS STATE & THERMODYNAMICSEXERCISE - level-0 Short Answer Type – II24videos
GASEOUS STATE & THERMODYNAMICS
- BOOK - VMC MODULES ENGLISHCHAPTER - ENERGY & MOMENTUMEXERCISE - JEE ADVANCE (ARCHIVE) - TRUE/FALSE TYPE1videos
ENERGY & MOMENTUM
- BOOK - VMC MODULES ENGLISHCHAPTER - GRAVITATIONEXERCISE - JEE Advance (Archive) TRUE/FALSE1videos
GRAVITATION
Similar Questions
At the top of the mountain, the thermometer reads 0∘C and the barometer reads 710mmHg. At the bottom of the mountain the temperature is 30∘C and the pressure is 760mmHg. The ratio of the density of air at the top with that at the bottom is
View SolutionThe temperature at the foot of a mountain is 30∘C and pressure is 760mmHg, whereas at the top of the mountain these are 0∘C and 710mmHg . Compare the densities of air at the foot and top of the mountain.
View SolutionThe volume of an air bubble becomes three times as it rises from the bottom of a lake to its surface. Assuming atmospheric pressure to be 75 cm of Hg and the density of water to be 1/10 of the density of mercury, the depth of the lake is
View SolutionThe height of a mercury barometer is 75 cm at sea level and 50 cm at the top of a hill. Ration of density of mercury to that of air is 104. The height of the hill is
View SolutionA barometer kept in an elevator reads 76 cm when it is at rest. If the elevator goes up with increasing speed, the reading will be
View SolutionAt 27∘C and 7.0 atm pressure, the density of propene gas is :
View SolutionA barometer is faulty . When the true barometer reading are 73 cm and 75 cm of Hg , the faulty barometer reads 69 cm and 70 cm respectively (i) What is the total length of the barometer tube? (ii) What is the reading when the faulty barometer reads 69.5cm? (iii) What is the faulty barometer reading when the true barometer reads 74cm?
View SolutionThe readings of a thermometer at 0∘C and 100∘C are 50cm and 75cm of mercury column respectively. Find the temperature at which its reading is 80cm of mercury column ?
View SolutionThe readings of a thermometer at 0∘C and 100∘C are 50cm and 75cm of mercury column respectively. Find the temperature at which its reading is 80cm of mercury column ?
View SolutionThe length of a faulty barometer is 90 cm and there is a little air above the mercury. When the atmospheric pressure is 76cm of Hg it reads 74.8 cm. What will be the true atmospheric pressure if the reading on the barometer is 74.3cm ?
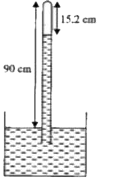 View Solution
View Solution

