Ideal mono-atomic gas is taken through process such that dQ = 3dU. The molar heat capacity for process is:
3R
4.5R
4R
2R
The correct Answer is:B
To find the molar heat capacity for the process where dQ=3dU for an ideal monoatomic gas, we can follow these steps:
Step 1: Understand the relationship between heat (dQ) and internal energy (dU)
Given that dQ=3dU, we can express the change in internal energy in terms of the number of moles and the molar heat capacity.
Step 2: Write the expression for change in internal energy (dU)
For an ideal monoatomic gas, the change in internal energy is given by:
dU=nCvdT
where n is the number of moles, Cv is the molar specific heat at constant volume, and dT is the change in temperature.
Step 3: Substitute the value of Cv
For a monoatomic ideal gas, the molar specific heat at constant volume Cv is:
Cv=32R
Thus, we can rewrite the equation for dU:
dU=n(32R)dT
Step 4: Write the expression for heat (dQ)
The heat added to the system can be expressed as:
dQ=nCdT
where C is the molar heat capacity for the process we are trying to find.
Step 5: Substitute dU into the equation for dQ
From the relationship dQ=3dU, we can substitute for dU:
dQ=3(n(32R)dT)
This simplifies to:
dQ=n(92R)dT
Step 6: Equate the two expressions for dQ
Now we have two expressions for dQ:
1. dQ=nCdT
2. dQ=n(92R)dT
Setting these equal to each other gives:
nCdT=n(92R)dT
Step 7: Cancel out common terms
Since n and dT are common in both sides, we can cancel them out:
C=92R
Step 8: Final answer
Thus, the molar heat capacity for the process is:
C=4.5R
Summary
The molar heat capacity for the process where dQ=3dU for an ideal monoatomic gas is 4.5R.
---
Given that dQ=3dU, we can express the change in internal energy in terms of the number of moles and the molar heat capacity.
For an ideal monoatomic gas, the change in internal energy is given by:
dU=nCvdT
where n is the number of moles, Cv is the molar specific heat at constant volume, and dT is the change in temperature.
For a monoatomic ideal gas, the molar specific heat at constant volume Cv is:
Cv=32R
Thus, we can rewrite the equation for dU:
dU=n(32R)dT
The heat added to the system can be expressed as:
dQ=nCdT
where C is the molar heat capacity for the process we are trying to find.
From the relationship dQ=3dU, we can substitute for dU:
dQ=3(n(32R)dT)
This simplifies to:
dQ=n(92R)dT
Now we have two expressions for dQ:
1. dQ=nCdT
2. dQ=n(92R)dT
nCdT=n(92R)dT
Since n and dT are common in both sides, we can cancel them out:
C=92R
Thus, the molar heat capacity for the process is:
C=4.5R
The molar heat capacity for the process where dQ=3dU for an ideal monoatomic gas is 4.5R.
Topper's Solved these Questions
- GASEOUS STATE & THERMODYNAMICSBook:VMC MODULES ENGLISHChapter:GASEOUS STATE & THERMODYNAMICSExercise:Level - 2
Explore 40 Videos
- GASEOUS STATE & THERMODYNAMICSBook:VMC MODULES ENGLISHChapter:GASEOUS STATE & THERMODYNAMICSExercise:JEE MAIN (ARCHIVE )
Explore 81 Videos
- GASEOUS STATE & THERMODYNAMICSBook:VMC MODULES ENGLISHChapter:GASEOUS STATE & THERMODYNAMICSExercise:level-0 Short Answer Type – II
Explore 24 Videos
- ENERGY & MOMENTUMBook:VMC MODULES ENGLISHChapter:ENERGY & MOMENTUMExercise:JEE ADVANCE (ARCHIVE) - TRUE/FALSE TYPE
Explore 1 Video
- GRAVITATIONBook:VMC MODULES ENGLISHChapter:GRAVITATIONExercise:JEE Advance (Archive) TRUE/FALSE
Explore 1 Video
Similar Questions
Ideal monoatomic gas is taken through a process dQ=2dU. Find the molar heat capacity (in terms of R) for the process? (where dQ is heat supplied and dU is change in internal energy)
View SolutionIdeal monoatomic gas is taken through a process dQ=2dU. Find the molar heat capacity (in terms of R) for the process? (where dQ is heat supplied and dU is change in internla energy)
View SolutionThe molar heat capacity for an ideal gas
View SolutionIdeal gas is taken through the process shown in the figure :
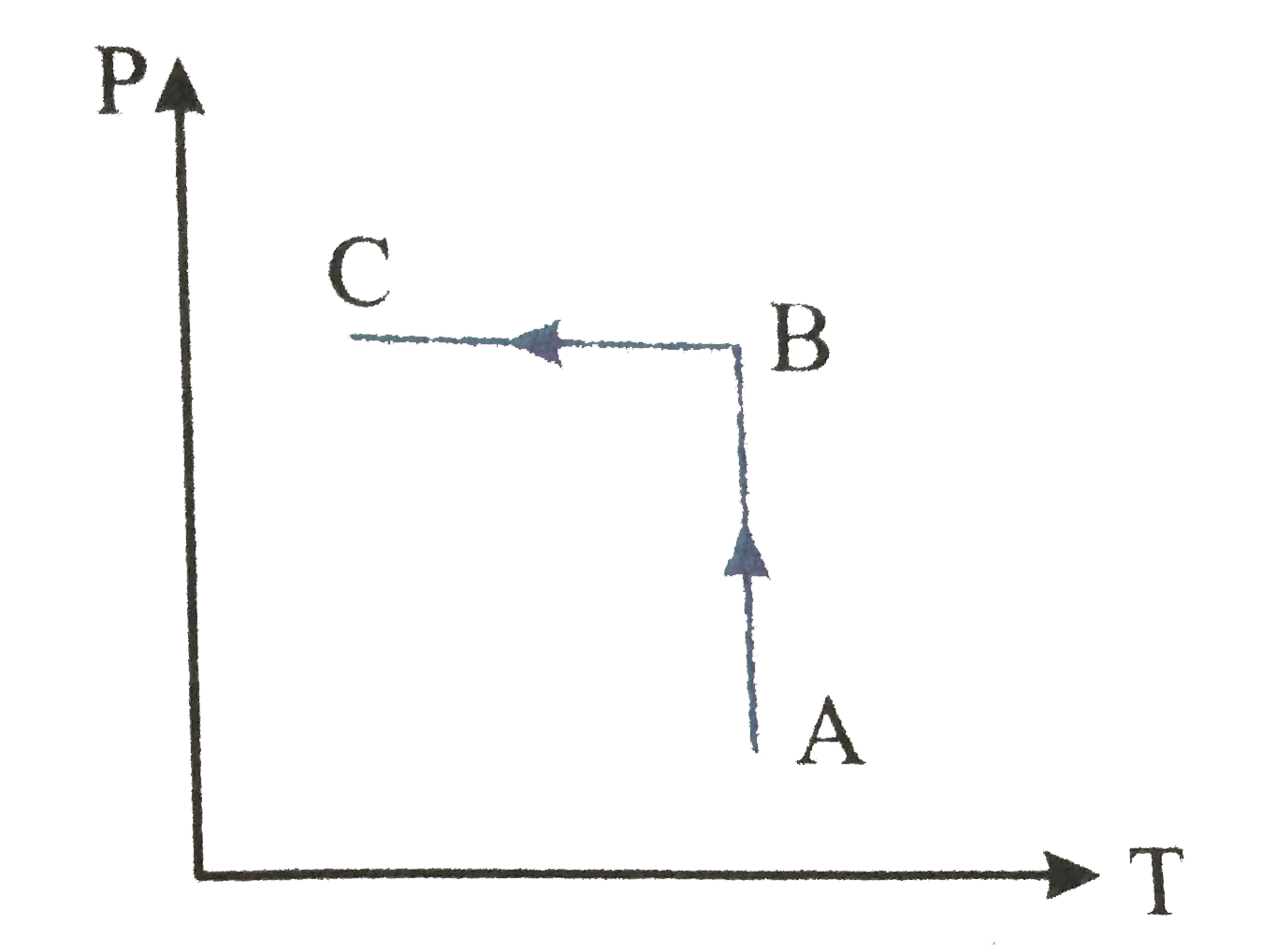
View SolutionIdeal gas is taken through the process shown in the figure :

View SolutionThe molar heat capacity for the process shown in fig. is

View SolutionOne mole of an ideal gas undergoes a process such that P∝1T. The molar heat capacity of this process is 4R.
View SolutionTwo moles of an ideal mono-atomic gas undergoes a thermodynamic process in which the molar heat capacity 'C' of the gas depends on absolute temperature as C=RTT0, where R is gas consant and T0 is the initial temperature of the gas. (V0 is the initial of the gas). Then answer the following questions:
The equation of process is
View Solution600J of heat is added to a monoatomic gas in a process in which the gas performs a work of 150J. The molar heat capacity for the process is
View SolutionThe molar heat capacity for the process shown in figure is
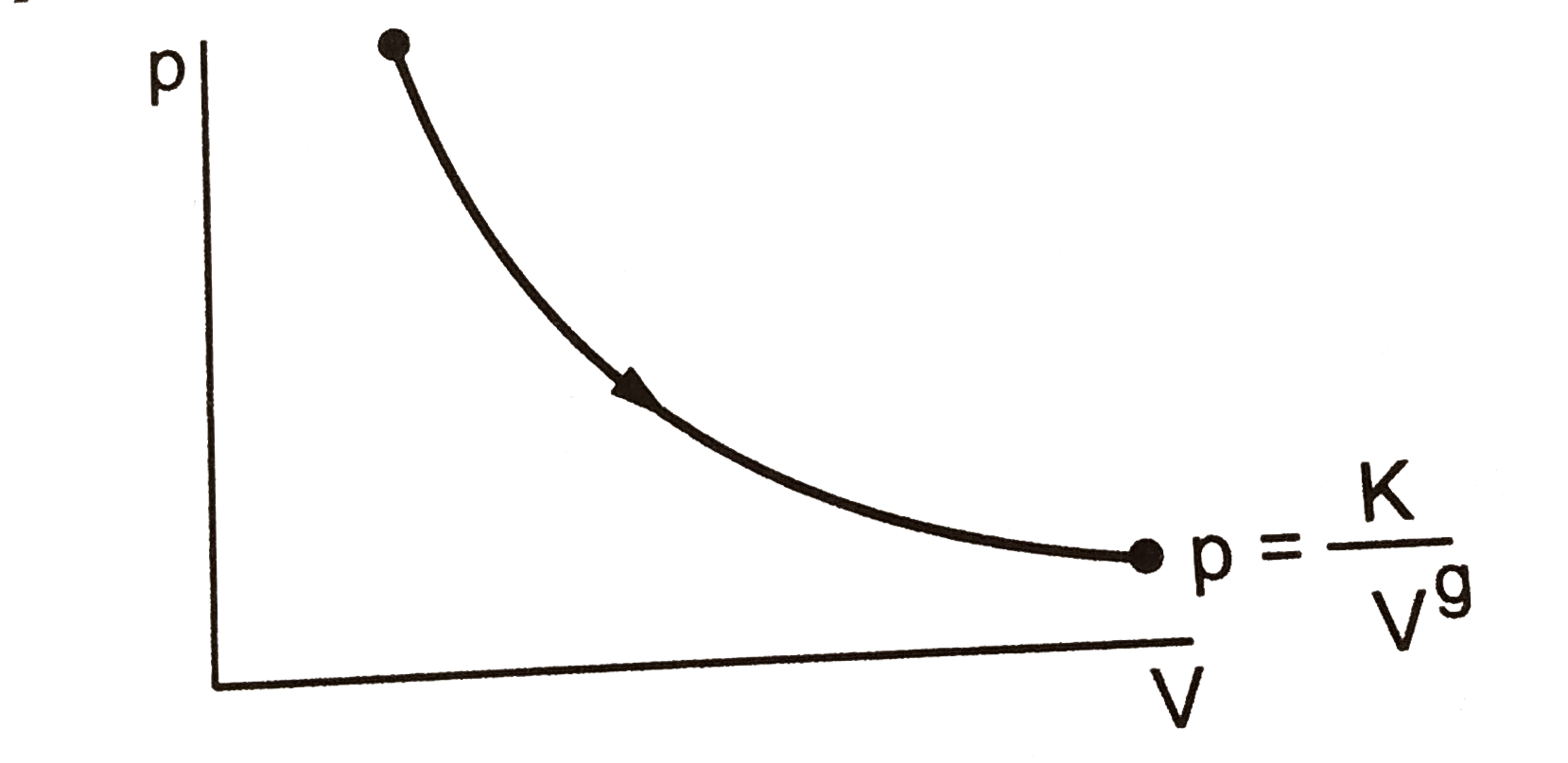
View Solution