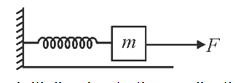
A block of mass m, lying on a smooth horizontal surface, is attached to a spring (of negligible mass) of spring constant k. The other end of the spring is fixed, as shown in the figure. The block is initally at rest in its equilibrium position. If now the block is pulled withe a constant force F, the maximum speed of the block is :

F√mk
πF√mk
Fπ√mk
2πF√mk
The correct Answer is:A
Initially, due to the application of constant force, the block starts accelerating due to the right & spring force starts increasing and the speed of the block starts increasing till the spring force becomes equal to constant force ‘F’. So speed is maximum when F=kx ⇒x=Fk.....(i)
usingW=ΔkE
ws+WF=12mv2⇒−12kx2+Fx=12mv2 ...(ii)
So when x=Fk,v=vmax.
so from (i)&(ii)⇒−12k(F2k2)+F(FK)=12mv2max⇒F22k=12mv2max⇒ Vmax=F√mk
Topper's Solved these Questions
- SIMPLE HARMONIC MOTION BOOK - VMC MODULES ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - 7-previous year question
46 Videos
- SIMPLE HARMONIC MOTION BOOK - VMC MODULES ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - LEVEL (2)
40 Videos
- ROTATIONAL MOTION BOOK - VMC MODULES ENGLISHCHAPTER - ROTATIONAL MOTION EXERCISE - JEE Advanced (Archive) (True/False Type)
3 Videos
- SYSTEM OF A PARTICLES & ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF A PARTICLES & ROTATIONAL MOTIONEXERCISE - IN-CHAPTER EXERCISE F
10 Videos
Similar Questions
A block of mass m is kept on a rough horizontal surface and is attached with a massless spring of force constant k. The minimum constant force applied on the other end of the spring of lift the block is
View SolutionA block of mass m moving with velocity v0 on a smooth horizontal surface hits the spring of constant k as shown. The maximum compression in spring is

View SolutionA block of mass m lies on a horizontal frictionless surface and is attached to one end of a horizontal spring (with spring constant k) whose other end is fixed . The block is intinally at rest at the position where the spring is unstretched (x=0). When a constant horizontal force →F in the positive direction of the x−axis is applied to it, a plot of the resulting kinetic energy of the block versus its position x is shown in figure. What is the magnitude of →F ?
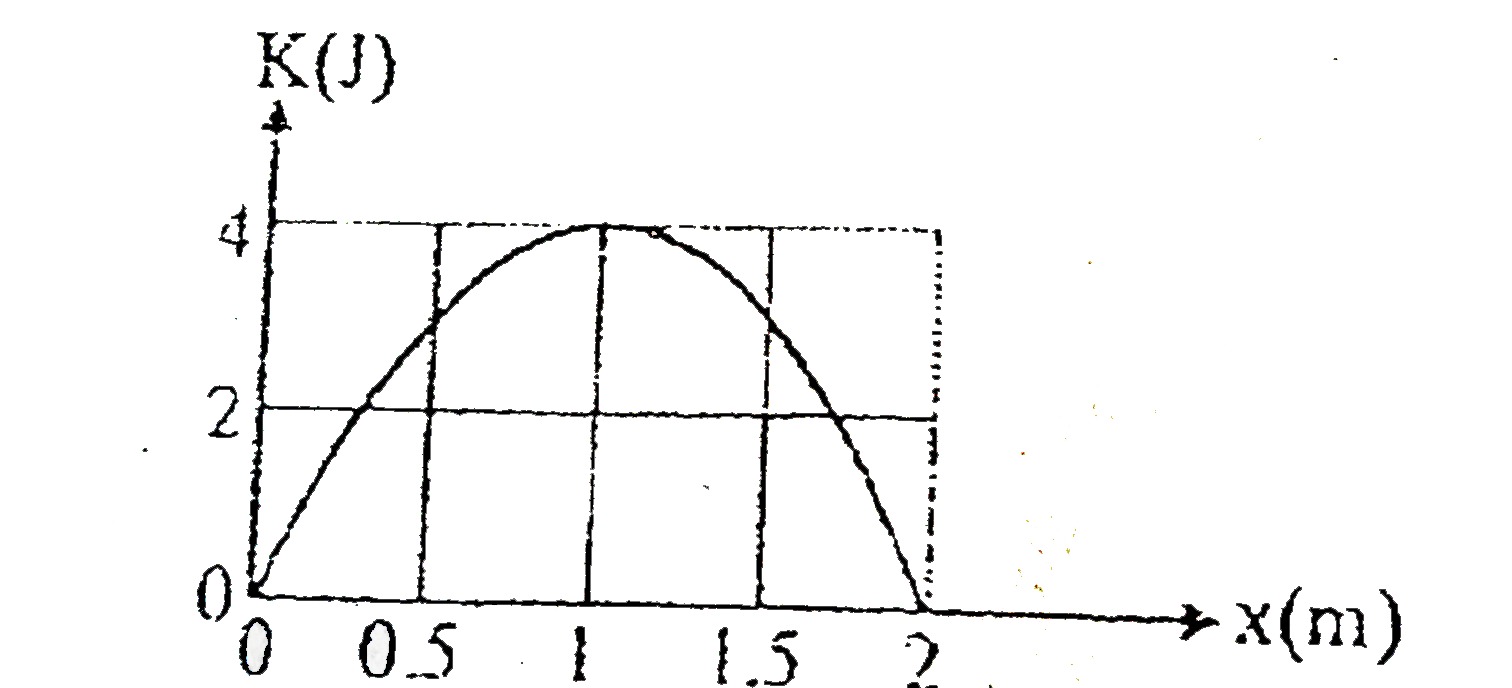
View SolutionA block of mass m compresses a spring iof stifffness k through a distacne l/2 as shown in the figure .If the block is not fixed to the spring the period of motion of the block is

View SolutionTwo identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in the figure. The time period of oscillation is

View SolutionA block of mass m is placed on a smooth horizontal floor is attached to one end of spring. The other end of the spring is attached to fixed support. When spring is vertical it is relaxed. Now the block is pulled to wards right by a force F, which is being increased gradually. When the speing makes angle 53∘ with the vertical, block leaves the floor.
Force constant of the spring is :-
View SolutionA block of mass m is placed on a smooth horizontal floor is attached to one end of spring. The other end of the spring is attached to fixed support. When spring is vertical it is relaxed. Now the block is pulled to wards right by a force F, which is being increased gradually. When the speing makes angle 53∘ with the vertical, block leaves the floor.
When the block leaves the table, the force F is :-
View SolutionFigure shown a block P of mass m resting on a smooth horizontal surface, attached to a spring of force constant k which is rigidly fixed on the wall on left side, shown in the figure . At a distance l to the right of the block there is a rigid wall. If block is pushed towards left so that spring is compressed by a distance 5l/3 and when released, if will starts its oscillations. If collision of block with the wall is considered to be perfectly elastic. Find the time period of oscillation of the block.

View SolutionA block of mass m is placed on a smooth horizontal floor is attached to one end of spring. The other end of the spring is attached to fixed support. When spring is vertical it is relaxed. Now the block is pulled to wards right by a force F, which is being increased gradually. When the speing makes angle 53∘ with the vertical, block leaves the floor.

When blocks leaves the table, the normal force on it from table is
View SolutionA block of mass m is attached to one end of a mass less spring of spring constant k. the other end of spring is fixed to a wall the block can move on a horizontal rough surface. The coefficient of friction between the block and the surface is μ then the compession of the spring for which maximum extension of the spring becomes half of maximum compression is .
View Solution
