A simple pendulum , made of a string of length l and a bob of mass m, is released from a small angle it strikes a block of mass M, kept on a horizontal surface at its lowest point of oscil,ations , elastically , it bounces back and goes up to an angle then M is given by :
The correct Answer is:B
To solve the problem, we will analyze the motion of the pendulum before and after it strikes the block. We will use the principles of conservation of momentum and energy in elastic collisions.
1. Understanding the Initial Conditions:
- A simple pendulum of length l and mass m is released from a small angle θ0.
- At the lowest point of its swing, the pendulum has maximum speed.
2. Finding the Speed of the Pendulum at the Lowest Point:
- The speed v of the pendulum bob at the lowest point can be derived from energy conservation:
v=√2gl(1−cosθ0)
- Here, g is the acceleration due to gravity.
3. Collision with the Block:
- The pendulum bob strikes a block of mass M at the lowest point. This is an elastic collision.
- Let Vm be the velocity of the block after the collision, and v1 be the velocity of the pendulum bob after the collision.
4. Applying Conservation of Momentum:
- Before the collision:
Total momentum=mv
- After the collision:
Total momentum=MVm+mv1
- Setting these equal gives:
m√2gl(1−cosθ0)=MVm+mv1(1)
5. Applying Conservation of Kinetic Energy:
- Since the collision is elastic, kinetic energy is conserved:
12mv2=12MV2m+12mv21
- This simplifies to:
m(2gl(1−cosθ0))=MV2m+mv21(2)
6. Finding the Velocities After Collision:
- The velocities v1 and Vm can be expressed in terms of the angles θ0 and θ1 (the angle the pendulum reaches after the collision):
v1=√2gl(1−cosθ1)
7. Substituting Back into the Equations:
- Substitute v1 into equation (1) and rearrange to isolate Vm:
MVm=m√2gl(1−cosθ0)−m√2gl(1−cosθ1)
8. Using the Elastic Collision Relation:
- In elastic collisions, the relationship between the velocities can be expressed as:
Vm=m−mM+m⋅(some terms)
9. Final Expression for Mass Ratio:
- After simplifying the equations and using trigonometric identities, we arrive at:
Mm=θ0−θ1θ0+θ1
Final Answer:
Mm=θ0−θ1θ0+θ1
- A simple pendulum of length l and mass m is released from a small angle θ0.
- At the lowest point of its swing, the pendulum has maximum speed.
- The speed v of the pendulum bob at the lowest point can be derived from energy conservation:
v=√2gl(1−cosθ0)
- Here, g is the acceleration due to gravity.
- The pendulum bob strikes a block of mass M at the lowest point. This is an elastic collision.
- Let Vm be the velocity of the block after the collision, and v1 be the velocity of the pendulum bob after the collision.
- Before the collision:
Total momentum=mv
- After the collision:
Total momentum=MVm+mv1
- Setting these equal gives:
m√2gl(1−cosθ0)=MVm+mv1(1)
- Since the collision is elastic, kinetic energy is conserved:
12mv2=12MV2m+12mv21
- This simplifies to:
m(2gl(1−cosθ0))=MV2m+mv21(2)
- The velocities v1 and Vm can be expressed in terms of the angles θ0 and θ1 (the angle the pendulum reaches after the collision):
v1=√2gl(1−cosθ1)
- Substitute v1 into equation (1) and rearrange to isolate Vm:
MVm=m√2gl(1−cosθ0)−m√2gl(1−cosθ1)
- In elastic collisions, the relationship between the velocities can be expressed as:
Vm=m−mM+m⋅(some terms)
- After simplifying the equations and using trigonometric identities, we arrive at:
Mm=θ0−θ1θ0+θ1
Mm=θ0−θ1θ0+θ1
Topper's Solved these Questions
- SIMPLE HARMONIC MOTION BOOK - VMC MODULES ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - 7-previous year question
46 Videos
- SIMPLE HARMONIC MOTION BOOK - VMC MODULES ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - LEVEL (2)
40 Videos
- ROTATIONAL MOTION BOOK - VMC MODULES ENGLISHCHAPTER - ROTATIONAL MOTION EXERCISE - JEE Advanced (Archive) (True/False Type)
3 Videos
- SYSTEM OF A PARTICLES & ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF A PARTICLES & ROTATIONAL MOTIONEXERCISE - IN-CHAPTER EXERCISE F
10 Videos
Similar Questions
A simple pendulm has a length L and a bob of mass M. The bob is vibrating with amplitude a .What is the maximum tension in the string?
View SolutionA block of mass m is placed at rest on a smooth wedge of mass M placed at rest on a smooth horizontal surface. As the system is released

View SolutionWhat is the period of oscillation of a simple pendulum if its bob is made of ice ?
View SolutionA simple pendulum with a bob of mass m is suspended from the roof of a car moving with horizontal acceleration a
View SolutionA simple pendulum of mass m and length L is held in horizontal position. If it is released from this position, then find the angular momentum of the bob about the point of suspension when it is vertically below the point of suspension.
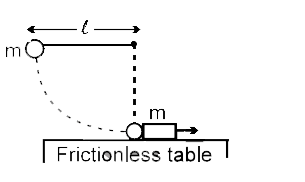
View SolutionThe bob of a simple pendulum of length l dropped from a horizontal position strikes a block of the same mass elastically, placed on a horizontal table (frictionless) as shown in the diagram, the block shall have kinetic energy-
View SolutionA stone tied to an inextensible string of length l=1m is kept horizontal. If it is released, find the angular speed of the stone when the string makes an angle θ=30∘ with horizontal.
View SolutionA rod of mass m is released on smooth horizontal surface making angle θ with horizontal. Then which of the following statement is correct ?
View SolutionA pendulum consists of a small mass m at the end of a string of length L. The pendulum is pulled aside making an angle θ with the vertical and released. Taking the suspension point as the axis, at the instant of release
View SolutionA bob of mass m attached with a string of length l tied to a point on ceiling is released from a position when its string is horizontal. At the bottom most point of its motion, an identical mass m gently stuck to it. Find the maximum angle from the vertical to which it rotates.
View Solution
