The instantaneous velocity of a particle moving in a straight line is given as a function of time by: v=v0(1−(tt0)n) where t0 is a constant and n is a positive integer . The average velocity of the particle between t=0 and t=t0 is 3v04.Then , the value of n is
The correct Answer is:C
To solve the problem, we need to find the value of n given the instantaneous velocity function and the average velocity condition. Let's break down the solution step by step.
Step 1: Write down the given instantaneous velocity function
The instantaneous velocity of the particle is given by:
v=v0(1−(tt0)n)
Step 2: Express displacement in terms of velocity
We know that instantaneous velocity can be expressed as:
v=dxdt
Thus, we can write:
dx=v0(1−(tt0)n)dt
Step 3: Integrate to find displacement
Integrate both sides from t=0 to t=t0 to find the displacement x:
x=∫t00v0(1−(tt0)n)dt
This can be split into two integrals:
x=v0∫t00dt−v0∫t00(tt0)ndt
Step 4: Evaluate the integrals
1. The first integral:
∫t00dt=t0
So, the first part becomes:
v0t0
2. The second integral:
∫t00(tt0)ndt=1tn0∫t00tndt=1tn0⋅tn+10n+1=t0n+1
Thus, the second part becomes:
v0⋅t0n+1
Step 5: Combine the results
Putting it all together, we have:
x=v0t0−v0⋅t0n+1=v0t0(1−1n+1)=v0t0⋅nn+1
Step 6: Calculate the average velocity
The average velocity Vavg over the interval t=0 to t=t0 is given by:
Vavg=xt0=v0t0⋅nn+1t0=v0⋅nn+1
Step 7: Set the average velocity equal to the given value
We know from the problem that:
Vavg=3v04
Setting these equal gives:
v0⋅nn+1=3v04
Step 8: Simplify the equation
Dividing both sides by v0 (assuming v0≠0):
nn+1=34
Step 9: Cross-multiply to solve for n
Cross-multiplying gives:
4n=3(n+1)
Expanding the right side:
4n=3n+3
Subtracting 3n from both sides:
n=3
Conclusion
Thus, the value of n is:
3
The instantaneous velocity of the particle is given by:
v=v0(1−(tt0)n)
We know that instantaneous velocity can be expressed as:
v=dxdt
Thus, we can write:
dx=v0(1−(tt0)n)dt
Integrate both sides from t=0 to t=t0 to find the displacement x:
x=∫t00v0(1−(tt0)n)dt
This can be split into two integrals:
x=v0∫t00dt−v0∫t00(tt0)ndt
1. The first integral:
∫t00dt=t0
So, the first part becomes:
v0t0
∫t00(tt0)ndt=1tn0∫t00tndt=1tn0⋅tn+10n+1=t0n+1
Thus, the second part becomes:
v0⋅t0n+1
Putting it all together, we have:
x=v0t0−v0⋅t0n+1=v0t0(1−1n+1)=v0t0⋅nn+1
The average velocity Vavg over the interval t=0 to t=t0 is given by:
Vavg=xt0=v0t0⋅nn+1t0=v0⋅nn+1
We know from the problem that:
Vavg=3v04
Setting these equal gives:
v0⋅nn+1=3v04
Dividing both sides by v0 (assuming v0≠0):
nn+1=34
Cross-multiplying gives:
4n=3(n+1)
Expanding the right side:
4n=3n+3
Subtracting 3n from both sides:
n=3
Thus, the value of n is:
3
Topper's Solved these Questions
- KINEMATICS OF A PARTICLE Book:VMC MODULES ENGLISHChapter:KINEMATICS OF A PARTICLE Exercise:JEE MAIN (archive)
Explore 19 Videos
- KINEMATICS OF A PARTICLE Book:VMC MODULES ENGLISHChapter:KINEMATICS OF A PARTICLE Exercise:JEE Advanced (archive)
Explore 14 Videos
- KINEMATICS OF A PARTICLE Book:VMC MODULES ENGLISHChapter:KINEMATICS OF A PARTICLE Exercise:LEVEL 1
Explore 75 Videos
- JEE MAIN REVISON TEST-23Book:VMC MODULES ENGLISHChapter:JEE MAIN REVISON TEST-23Exercise:PHYSICS (SECTION 2)
Explore 1 Video
- LAWS OF MOTION Book:VMC MODULES ENGLISHChapter:LAWS OF MOTION Exercise:IMPECCABLE
Explore 53 Videos
Similar Questions
The velocity of a particle is zero at t=0
View SolutionThe velocity of an object moving rectilinearly is given as a function of time by v=4t−3t2 where v is in m/s and t is in seconds. The average velocity if particle between t=0 to t=2 seconds is
View SolutionThe velocity 'v' of a particle moving along straight line is given in terms of time t as v=3(t2−t) where t is in seconds and v is in m/s.
The speed is minimum after t=0 second at instant of time
View SolutionThe velocity 'v' of a particle moving along straight line is given in terms of time t as v=3(t2−t) where t is in seconds and v is in m/s.
The distance travelled by particle from t=0 to t=2seconds is :
View SolutionThe velocity 'v' of a particle moving along straight line is given in terms of time t as v=3(t2−t) where t is in seconds and v is in m/s.
The displacement of aprticle from t=0 to t=2 seconds is :
View SolutionThe velocity time graph of a particle moving in a straight line is given line is given in the figure. Then starting from t=0, the particle
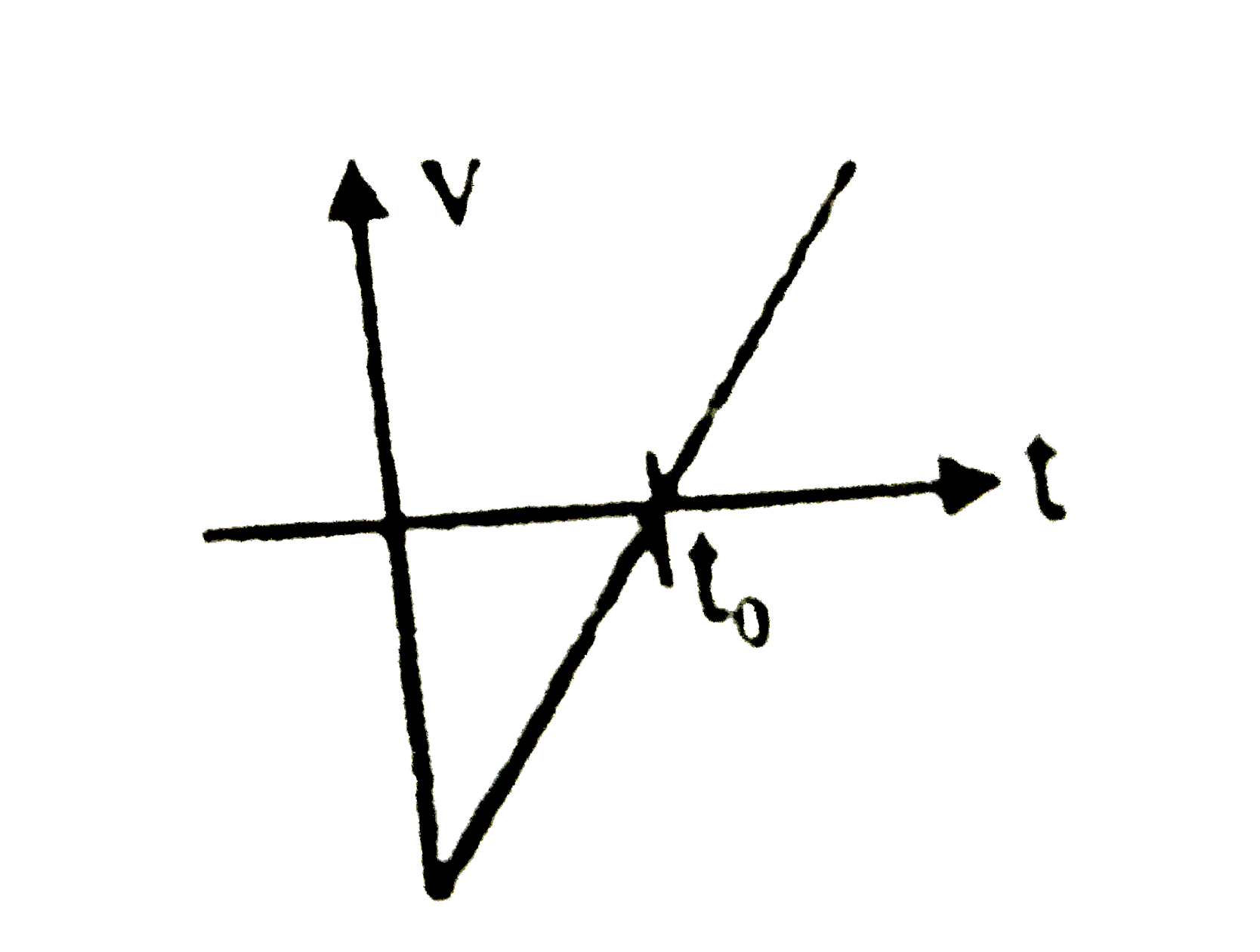
View SolutionA particle moving along x-axis has acceleration f, at time t, given by f=f0(1−tT), where f0 and T are constant.
The particle at t=0 has zero velocity. In the time interval between t=0 and the instant when f=0, the particle's velocity (vx) is :
View SolutionA particle moving along x-axis has acceleration f, at time t, given by f=f0(1−tT), where f0 and T are constants. The particle at t=0 has zero velocity. In the time interval between t=0 and the instant when f=0, the particle's velocity (vx) is
View SolutionAcceleration time graph of a particle moving along a straight line is given. Then average acceleration between t=0 & t=4 is :−
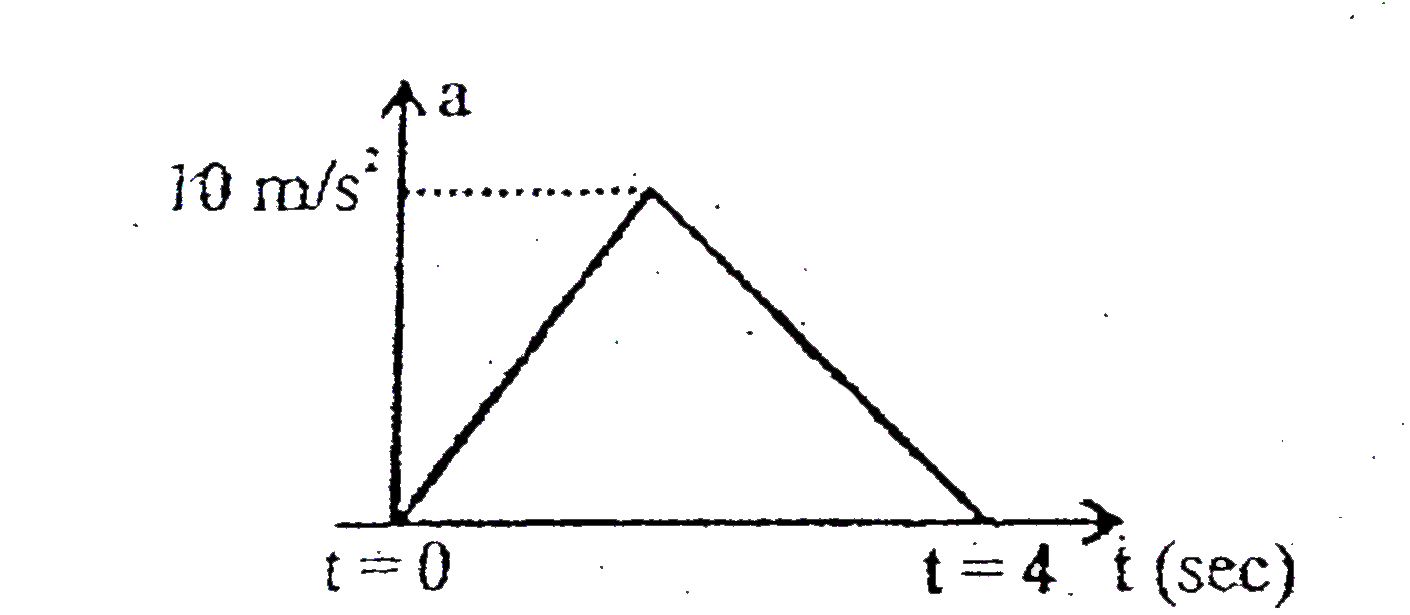
View Solutionvelocity-time graph of a particle moving in a straight line is as shown in figure. At time t=0, velocity of the particle is zero. Find
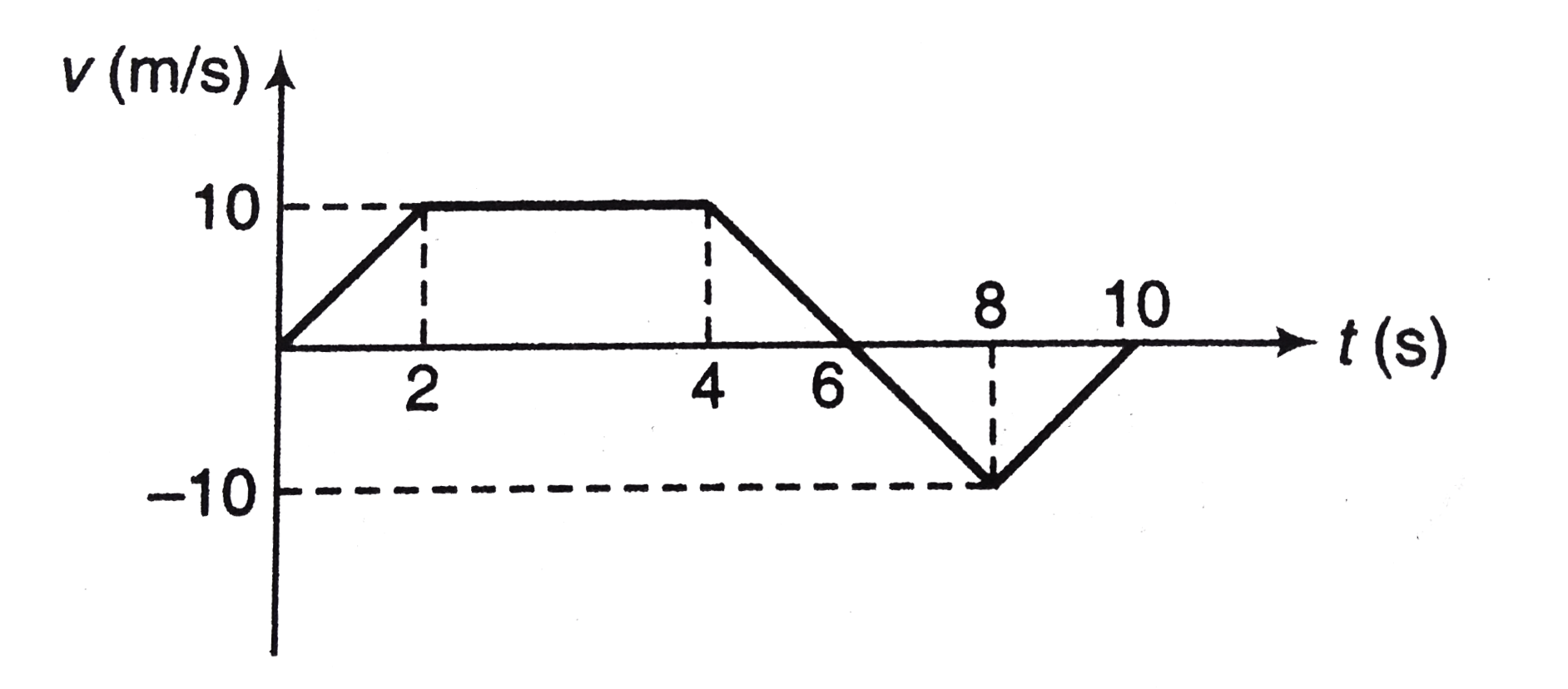
(a) average acceleration in a time interval from t=6s to t=12s,
(b) velocity of the particle at t=14s.
View Solution
