A man of mass m stands on a long flat car of mass M , moving with velocity V.If he now begins to run with velocity u, with respect to the car , in the same direction as V, the the velocity of the car will be
(a)V−mu/M
(b)V−mu/(m+M)
(c)V+mu/(m+M)
(d)V−ut(M−m)/(M+m)
The correct Answer is:B
To solve the problem, we will use the principle of conservation of momentum. Let's break down the steps:
1. Identify the Initial Momentum:
The initial momentum of the system (man + car) can be expressed as:
Pinitial=(M+m)V
where M is the mass of the car, m is the mass of the man, and V is the initial velocity of the car.
2. Define the Final Velocities:
Let vc be the final velocity of the car after the man starts running, and vm be the final velocity of the man. Since the man runs with velocity u with respect to the car, we can express the man's velocity as:
vm=vc+u
3. Write the Final Momentum:
The final momentum of the system after the man starts running is:
Pfinal=Mvc+mvm=Mvc+m(vc+u)=Mvc+mvc+mu
Simplifying this gives:
Pfinal=(M+m)vc+mu
4. Apply Conservation of Momentum:
According to the conservation of momentum, the initial momentum must equal the final momentum:
(M+m)V=(M+m)vc+mu
5. Rearranging the Equation:
Rearranging the equation to solve for vc:
(M+m)vc=(M+m)V−mu
vc=(M+m)V−muM+m
6. Final Expression for the Velocity of the Car:
Thus, the final velocity of the car vc is:
vc=V−muM+m
Conclusion:
The velocity of the car after the man starts running is given by:
vc=V−muM+m
The initial momentum of the system (man + car) can be expressed as:
Pinitial=(M+m)V
where M is the mass of the car, m is the mass of the man, and V is the initial velocity of the car.
Let vc be the final velocity of the car after the man starts running, and vm be the final velocity of the man. Since the man runs with velocity u with respect to the car, we can express the man's velocity as:
vm=vc+u
The final momentum of the system after the man starts running is:
Pfinal=Mvc+mvm=Mvc+m(vc+u)=Mvc+mvc+mu
Simplifying this gives:
Pfinal=(M+m)vc+mu
According to the conservation of momentum, the initial momentum must equal the final momentum:
(M+m)V=(M+m)vc+mu
Rearranging the equation to solve for vc:
(M+m)vc=(M+m)V−mu
vc=(M+m)V−muM+m
Thus, the final velocity of the car vc is:
vc=V−muM+m
The velocity of the car after the man starts running is given by:
vc=V−muM+m
Topper's Solved these Questions
- ENERGY & MOMENTUMBOOK - VMC MODULES ENGLISHCHAPTER - ENERGY & MOMENTUMEXERCISE - LEVEL - 2 PARAGRAPH QUESTIONS
2 Videos
- ENERGY & MOMENTUMBOOK - VMC MODULES ENGLISHCHAPTER - ENERGY & MOMENTUMEXERCISE - JEE MAIN (ARCHIVE)
53 Videos
- ENERGY & MOMENTUMBOOK - VMC MODULES ENGLISHCHAPTER - ENERGY & MOMENTUMEXERCISE - Level - 1 PARAGRAPH QUESTIONS
3 Videos
- ELECTROSTATICSBOOK - VMC MODULES ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - JEE Advanced (Archive)
89 Videos
- GASEOUS STATE & THERMODYNAMICSBOOK - VMC MODULES ENGLISHCHAPTER - GASEOUS STATE & THERMODYNAMICSEXERCISE - JEE ADVANCED (ARCHIVE )
111 Videos
Similar Questions
A girl of mass 50 kg stands on a railroad car of mass 75 kg moving with velocity 20ms−1 . Now , the girl begins to run with a velocity of 10ms−1 with respect to the car in the same direction , as that of the car. The velocity of the car at this instant will be
View SolutionWhat is the momentum of an object of mass m , moving with a velocityv ?
View SolutionA man of mass m moves on a plank of mass M with a constant velocity u with respect to the plank, as shown in figure.
a.If the plank rests on smooth horizontal surface, determine the velocity of the plank. .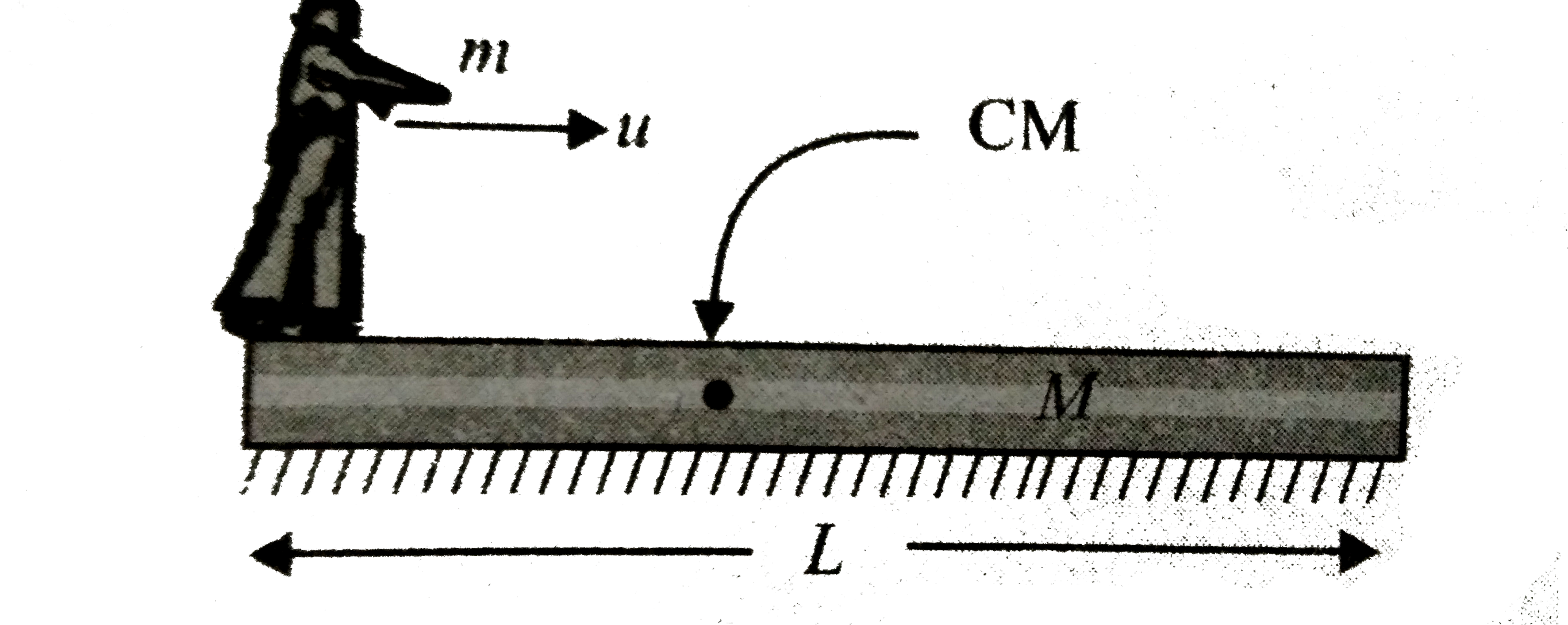
View Solutiona. A rail road car of mass M is moving without friction on a straight horizontal track with a velocity ct. A man of mass m lands on it normally from a helicopter. What will be the new velocity of the car? ltbgt b. If now the man begins to run on it with speed um with respect to car in a direction opposite to motion of the car, what will be the new velocity of the car?
View SolutionA motorcylcle is moving with a velocity 80km/hr ahead of a car moving with a velocity of 65 km/hr in the same direction. What is the relative velocity of the motorcyle with respect ot the car:-
View SolutionA man of mass m starts moving on a plank of mass M with constant velocity v with respect to plank. If the plank lies on a smooth horizontal surface, then velocity of plank with respect to ground is
View SolutionA flat car of mass M is at rest on a frictionless floor with a child of mass m standing at its edge. If the child jumps off from the car towards right with an initial velocity u, with respect to the car, find the velocity of the car after its jump.
View SolutionA man of mass m is standing on a stationary flat car of mass M. The car can move without friction along horizontal rails. The man starts walking with velocity v relative to the car. Work done by him
View SolutionThe minimum stopping distance of a car moving with velocity u is x. If the car is moving with velocity 2v, then the minimum stopping distance will be.
View SolutionA car is moving with velocity →vc towards north and a train is moving towards east with velocity →vt . Find the direction of relative velocity of car with respect to train.
View Solution
