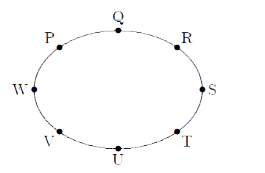
A planet travels in an elliptical orbit about a star as shown. At what pair of points is the speed of the planet the same?
A Pitot tube (also called Prandt tube or Wind speed indicator) consists of a bent tube ABCD with small apertures at A and D. This tube is inserted in pipe P through which the fluid (air in this case) is flowing. Let ρ be the density of air. The plane of the aperture A is parallel to the direction of air flow. As such, the velocity (v1) of air at A is the same as its velocity in pipe (which is equal to the velocity v of plane relative to air). Let P1 be the pressure at A. The plane of the aperture at D is normal to the direction of air-flow in the pipe. The velocity (v2) of air-flow rapidly falls to zero at D and the pressure increases to P2 . Applying Bernoulli’s theorem to the horizontal flow at A and D, we get
P1+12ρv21=P2+12ρv22orv=√2(P1−P2)ρ) (as v1=v and v2=0 )
but P1−P2=hσg where σ is the density of the liquid in the tube ABCD.
As h=40cm=0.4m,σ=0.8×1000kg/m3,ρ=1kg/m3,v=√2×0.4(0.8×1000)9.81m/s=79.2m/s
Topper's Solved these Questions
- BOOK - VMC MODULES ENGLISHCHAPTER - LIQUIDSEXERCISE - LEVEL -190videos
LIQUIDS
- BOOK - VMC MODULES ENGLISHCHAPTER - LIQUIDSEXERCISE - LEVEL -250videos
LIQUIDS
- BOOK - VMC MODULES ENGLISHCHAPTER - LIQUIDSEXERCISE - LEVEL-0 ( Short Answer Type - I )7videos
LIQUIDS
- BOOK - VMC MODULES ENGLISHCHAPTER - LAWS OF MOTION EXERCISE - IMPECCABLE53videos
LAWS OF MOTION
- BOOK - VMC MODULES ENGLISHCHAPTER - MAGNETIC EFFECTS OF CURRENT EXERCISE - JEE Advanced (Archive)78videos
MAGNETIC EFFECTS OF CURRENT
Similar Questions
Knowledge Check
- Question 1 - Select One
A planet revolves in elliptical orbit around the sun. (see figure). The linear speed of the planet will be maximum at
 AABBCCDD
AABBCCDD - Question 2 - Select One
A planet orbits the sun in an elliptical path as shown in the figure. Let vp and vA be spped of the planet when at perohelion and aphelion respectively. Which of the following relations is correct ?
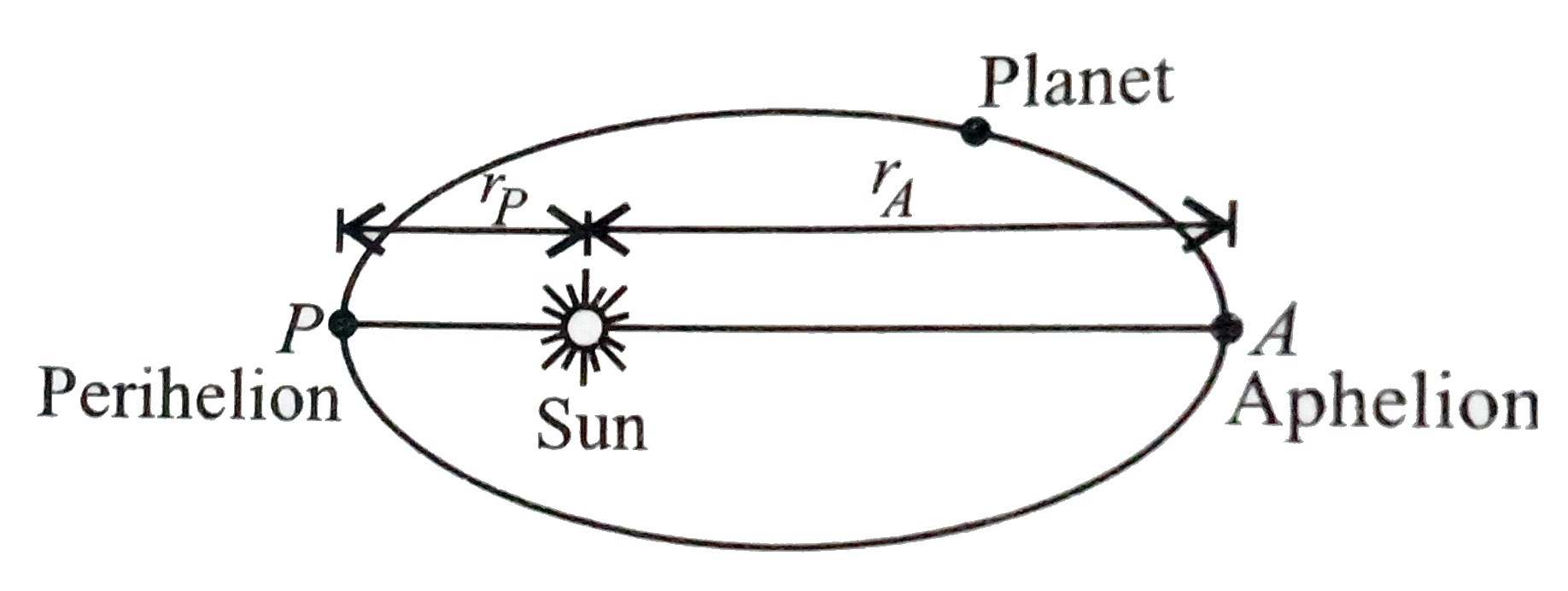 ArprA=vAvpBrprA=vpvACrprA=√vpvADrprA=√vAvp
ArprA=vAvpBrprA=vpvACrprA=√vpvADrprA=√vAvp - Question 3 - Select One
A planet revolves around the sun in elliptical orbit of eccentricity 'e'. If 'T' is the time period of the planet then the time spent by the planet between the ends of the minor axis and major axis close to the sun is
ATπ2eBT(2eπ−1)CTe2πDT(14−e2π)
A planet of mass m is moving in an elliptical orbit about the sun (mass of sun = M). The maximum and minimum distances of the planet from the sun are r1 and r2 respectively. The period of revolution of the planet wil be proportional to :
View SolutionA planet is moving round the sun in an elliptical orbit as shows. As the planet moves from A to B
 View Solution
View SolutionA planet is revolving in an elliptical orbit around the sun as shown in the figure. The areal velocity (area swapped by the radius vector with respect to sun in unit time) is:
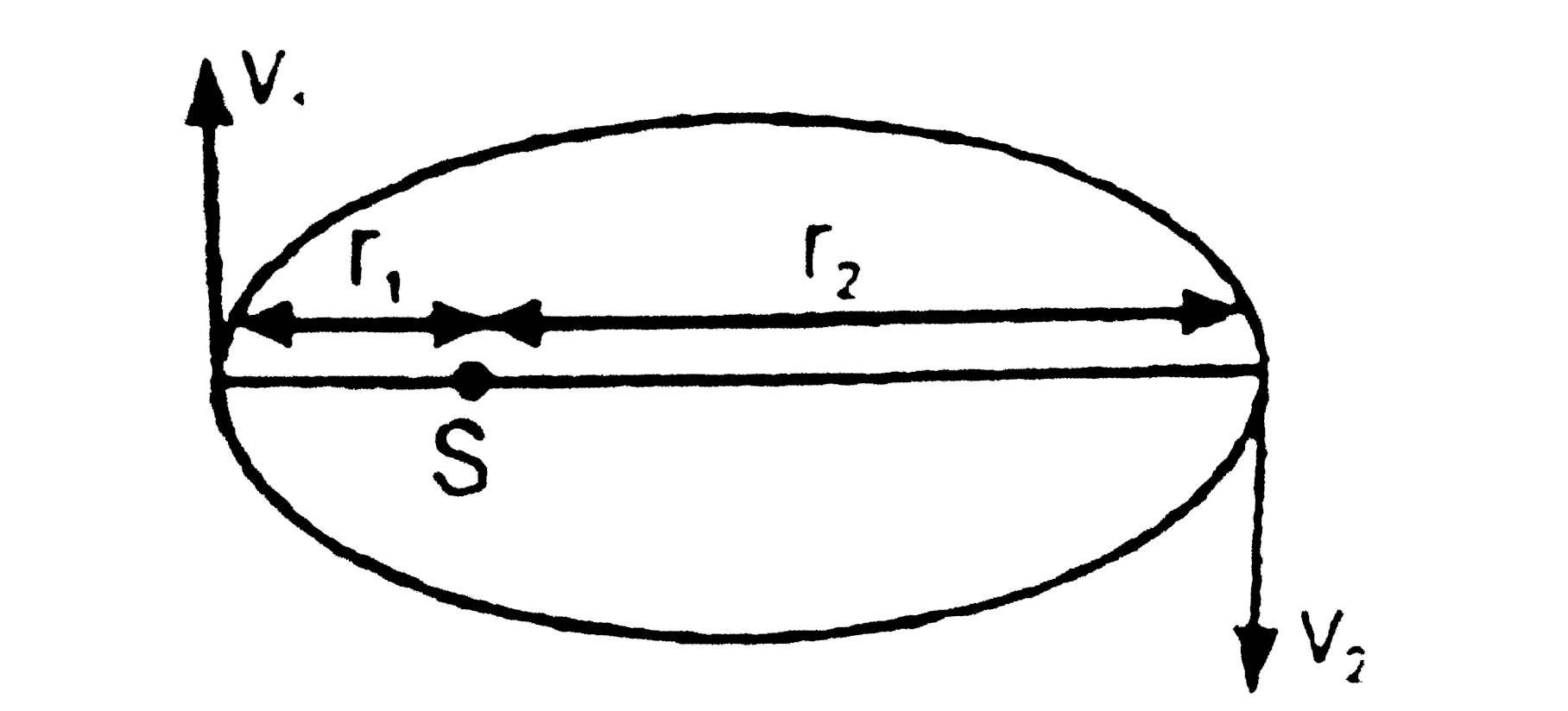 View Solution
View SolutionIn the figure shown a planet moves in an elliptical orbit around the sun. Compare the speeds, linear and angular momenta at the points A and B.
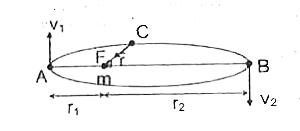 View Solution
View SolutionA planet of mass m is in an elliptical orbit about the sun (m << Msun) with an orbital period T. If A be the area of orbit, then its angular momentum would be:
View Solution

