Two rods of different materials having coefficient of thermal expansion α1,α2 and young's modulii Y1,Y2 respectively are fixed between two rigid massive walls. The rods are heated such that they undergo the same increase in temperature. There is no bending of rods. If α1:α2=2:3, the thermal stresses developed in the two rods are equal provided Y1:Y2 is equal to
2:3
1:1
3:2
4:9
The correct Answer is:C
To solve the problem step by step, we will analyze the thermal stresses developed in two rods made of different materials when they are subjected to the same increase in temperature.
Step 1: Understand the Problem
We have two rods fixed between rigid walls, and they are heated such that they undergo the same increase in temperature (ΔT). The coefficients of thermal expansion for the two rods are given as α₁ and α₂, and their Young's moduli are Y₁ and Y₂. We need to find the ratio of Young's moduli (Y₁ : Y₂) under the condition that the thermal stresses in both rods are equal.
Step 2: Write the Expression for Thermal Stress
The thermal stress (σ) in a rod can be expressed using the formula:
σ=Y⋅strain
Where strain (ε) is given by:
strain=ΔLL0=α⋅ΔT
Here, ΔL is the change in length, L₀ is the original length, α is the coefficient of thermal expansion, and ΔT is the change in temperature.
Step 3: Express Thermal Stress for Both Rods
For rod 1:
σ1=Y1⋅α1⋅ΔT
For rod 2:
σ2=Y2⋅α2⋅ΔT
Step 4: Set the Thermal Stresses Equal
Since the thermal stresses in both rods are equal, we can set the two expressions for stress equal to each other:
Y1⋅α1⋅ΔT=Y2⋅α2⋅ΔT
Step 5: Cancel Out ΔT
Since ΔT is the same for both rods and is not equal to zero, we can cancel it from both sides:
Y1⋅α1=Y2⋅α2
Step 6: Rearrange the Equation
Rearranging the equation gives us:
Y1Y2=α2α1
Step 7: Substitute the Given Ratio of α
We are given that the ratio of the coefficients of thermal expansion is:
α1α2=23
This implies:
α2α1=32
Step 8: Substitute into the Young's Moduli Ratio
Now substituting this ratio into our rearranged equation:
Y1Y2=32
Conclusion
Thus, the ratio of Young's moduli is:
Y1:Y2=3:2
We have two rods fixed between rigid walls, and they are heated such that they undergo the same increase in temperature (ΔT). The coefficients of thermal expansion for the two rods are given as α₁ and α₂, and their Young's moduli are Y₁ and Y₂. We need to find the ratio of Young's moduli (Y₁ : Y₂) under the condition that the thermal stresses in both rods are equal.
The thermal stress (σ) in a rod can be expressed using the formula:
σ=Y⋅strain
Where strain (ε) is given by:
strain=ΔLL0=α⋅ΔT
Here, ΔL is the change in length, L₀ is the original length, α is the coefficient of thermal expansion, and ΔT is the change in temperature.
For rod 1:
σ1=Y1⋅α1⋅ΔT
σ2=Y2⋅α2⋅ΔT
Since the thermal stresses in both rods are equal, we can set the two expressions for stress equal to each other:
Y1⋅α1⋅ΔT=Y2⋅α2⋅ΔT
Since ΔT is the same for both rods and is not equal to zero, we can cancel it from both sides:
Y1⋅α1=Y2⋅α2
Rearranging the equation gives us:
Y1Y2=α2α1
We are given that the ratio of the coefficients of thermal expansion is:
α1α2=23
This implies:
α2α1=32
Now substituting this ratio into our rearranged equation:
Y1Y2=32
Thus, the ratio of Young's moduli is:
Y1:Y2=3:2
Topper's Solved these Questions
- PROPERTIES OF MATTERBOOK - VMC MODULES ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - Level -2
50 Videos
- PROPERTIES OF MATTERBOOK - VMC MODULES ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - JEE Main (Archive) Level - 1
48 Videos
- PROPERTIES OF MATTERBOOK - VMC MODULES ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - JEE Advanced (Archive) Level - 2 (MATRIX MATCH TYPE)
1 Videos
- MOVING CHARGES & MAGNETISM BOOK - VMC MODULES ENGLISHCHAPTER - MOVING CHARGES & MAGNETISM EXERCISE - IN-CHAPTER EXERCISE-K
10 Videos
- QUIZBOOK - VMC MODULES ENGLISHCHAPTER - QUIZEXERCISE - PHYSICS
30 Videos
Similar Questions
Two rods of different materials having coefficients of thermal expansion α1, α2 and Young's modulii Y1, Y2 respectively are fixed between two rigid massive walls. The rods are heated such that they undergo the same increase in temperature. There is no bending of the rods. If α1:α2=2:3, the thermal stresses developed in the two rods are equal provided Y1:Y2 is equal to
View SolutionTwo rods of different materials having coefficient of thermal expansion α1 and α2 and Young's modulus Y1 and Y2 respectively are fixed between two rigid supports separately. The rods are heated such that they undergo same temperature increment. If thermal stress developed in two rods are equal, then Y1:Y2 is
View SolutionTwo rods of lengths l1andl2 are made of materials having coefficients of linear expansion α1andα2 respectively. What could be the relation between above values, if the difference in the lengths of the two rods does not depends on temperature variation?
View SolutionTwo rods of length l1 and l2 are made of material whose coefficient of linear expansion are α1 and α2 , respectively. The difference between their lengths will be independent of temperatiure if l1/l2 is to
View SolutionTwo metallic rods of length l and 3l have coefficient of linear expansion α and 3α respectively. The coefficient of linear expansion ofr their series combinations, is
View SolutionThere are two rods of length l1 and l2 and coefficient of linear expansions are α1 and α2 respectively.
Find equivalent coefficient of thermal expansion for their combination in series.
View SolutionTwo rods are joined between fixed supports as shown in the figure. Condition for no change in the length of individual rods with the increase of temperature will be
(α1,α2= linear expansion coefficient
A1,A2= Area of rods
Y1,Y2= Young modulus )
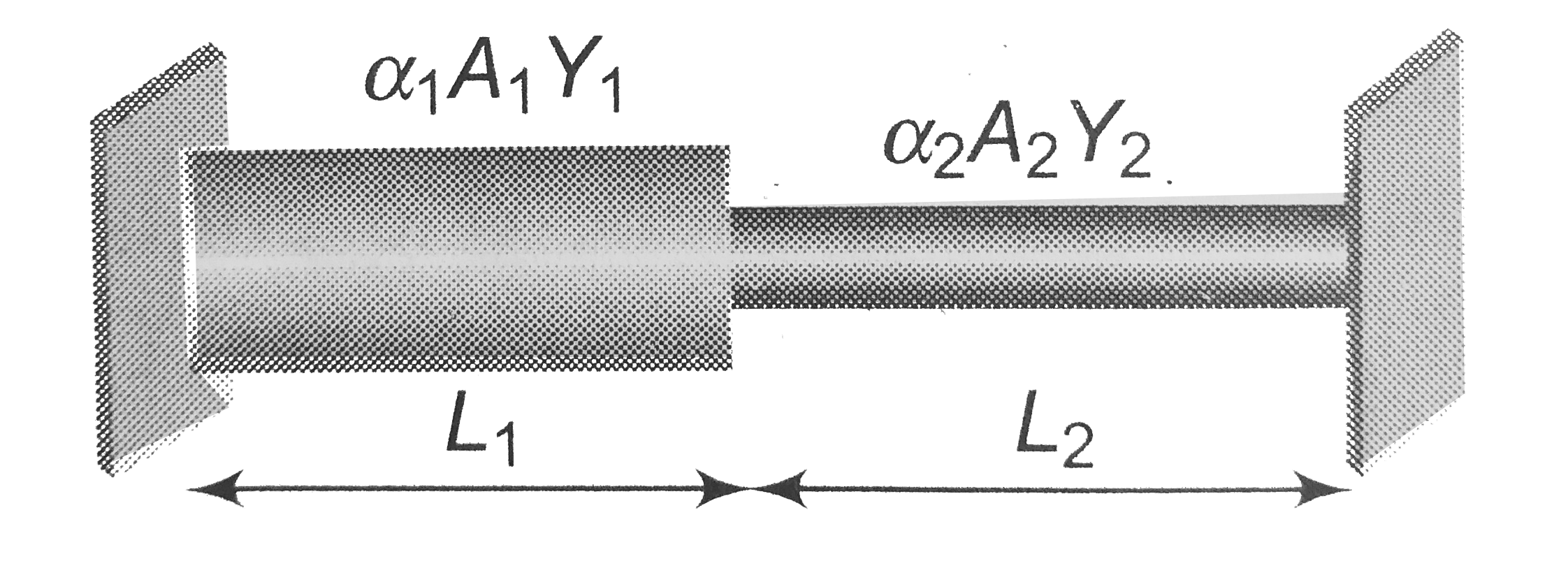
View SolutionTwo rods are joined between fixed supports as shown in the figure. Condition for no change in the length of individual rods with the increase of temperature will be
(α1,α2= linear expansion coefficient
A1,A2= Area of rods
Y1,Y2= Young modulus )

View SolutionTwo rods are joined between fixed supports as shown in the figure. Condition for no change in the length of individual rods with the increase of temperature will be
(α1,α2= linear expansion coefficient
A1,A2= Area of rods
Y1,Y2= Young modulus )

View SolutionTwo rods of different materials are placed between massive walls as shown in figure. The cross section of the rods is A, their moduil of elastricity are E1 and E2 respectively. If rods are heated by t degrees, then (coefficients of liner expansion of material of rods are α1 and α2 respectively)
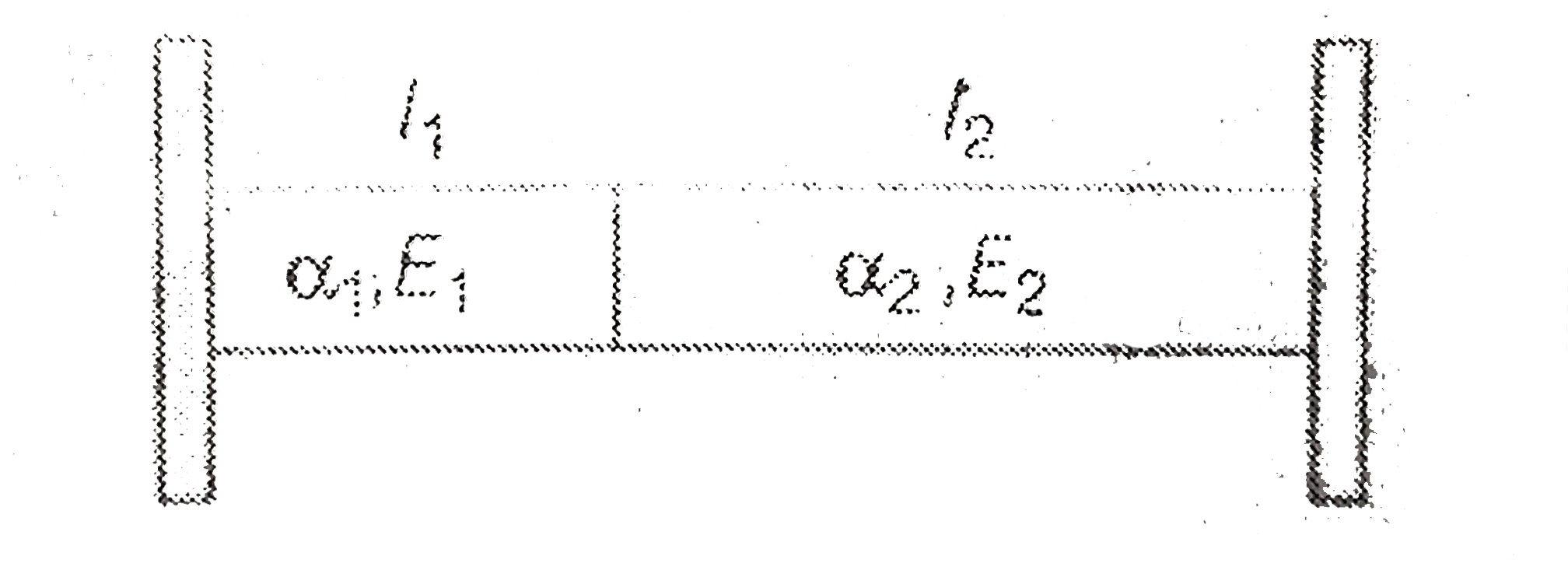
View Solution
