A cube of side 40 cm has its upper face displaced by 0.1 mm by a tangential force of 8 Kn. The shearing modulus of cube is :-
2×109Nm−2
4×109Nm−2
8×109Nm−2
16×109Nm−2
The correct Answer is:A
To find the shearing modulus of the cube, we will use the formula for shearing modulus (also known as the modulus of rigidity), which is given by:
G=Shearing StressShearing Strain
Step 1: Calculate the Shearing Stress
Shearing stress (τ) is defined as the tangential force (F) applied per unit area (A).
The formula for shearing stress is:
τ=FA
Given:
- Force, F=8 kN=8×103 N
- The area of the upper face of the cube, A=side2=(40 cm)2=(0.4 m)2=0.16 m2
Now, substituting the values into the formula:
τ=8×103 N0.16 m2=50000 N/m2=5×104 N/m2
Step 2: Calculate the Shearing Strain
Shearing strain (γ) is defined as the displacement (ΔL) divided by the original length (L).
The formula for shearing strain is:
γ=ΔLL
Given:
- Displacement, ΔL=0.1 mm=0.1×10−3 m
- Original length (side of the cube), L=40 cm=0.4 m
Now, substituting the values into the formula:
γ=0.1×10−3 m0.4 m=0.1×10−30.4=0.25×10−3=2.5×10−4
Step 3: Calculate the Shearing Modulus
Now that we have both shearing stress and shearing strain, we can calculate the shearing modulus (G):
G=τγ
Substituting the values we calculated:
G=5×104 N/m22.5×10−4=2×108 N/m2
Final Answer
The shearing modulus of the cube is:
G=2×108 N/m2
Shearing stress (τ) is defined as the tangential force (F) applied per unit area (A).
- Force, F=8 kN=8×103 N
- The area of the upper face of the cube, A=side2=(40 cm)2=(0.4 m)2=0.16 m2
Shearing strain (γ) is defined as the displacement (ΔL) divided by the original length (L).
- Displacement, ΔL=0.1 mm=0.1×10−3 m
- Original length (side of the cube), L=40 cm=0.4 m
Now that we have both shearing stress and shearing strain, we can calculate the shearing modulus (G):
The shearing modulus of the cube is:
Topper's Solved these Questions
- PROPERTIES OF MATTERBOOK - VMC MODULES ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - Level -2
50 Videos
- PROPERTIES OF MATTERBOOK - VMC MODULES ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - JEE Main (Archive) Level - 1
48 Videos
- PROPERTIES OF MATTERBOOK - VMC MODULES ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - JEE Advanced (Archive) Level - 2 (MATRIX MATCH TYPE)
1 Videos
- MOVING CHARGES & MAGNETISM BOOK - VMC MODULES ENGLISHCHAPTER - MOVING CHARGES & MAGNETISM EXERCISE - IN-CHAPTER EXERCISE-K
10 Videos
- QUIZBOOK - VMC MODULES ENGLISHCHAPTER - QUIZEXERCISE - PHYSICS
30 Videos
Similar Questions
A 4 cm cube has its upper face displaced by 0.1 mm by a tangential force of 8 kN. Calculate the shear modulus of the cube.
View SolutionA 0.05m cube has its upper face displaced by 0.2cm by a tangential force of 8N. Calculate the shearing strain, shearing stress and modulus of rigidity of the material of the cube.
View Solution
Knowledge Check
The metal cube of side 10 cm is subjected to a shearing stress of 104Nm−2. The modulus of rigidity if the top of the cube is displaced by 0.05 cm with respect to its bottom is
A2×106Nm−2B10x5Nm−2C1×107Nm−2D4×105Nm−2
A shearing force of 106 N is applied on the top surface of a fixed cube of side 10 cm. If the top surface is displaced by 0.33 mm, find rigidity modulus of the material of the cube.
View SolutionAs shown in the figure forces of 105 N each are applied in opposite direction on the upper and lower faces of a cube of side 10 cm shifting the upper face parallel to itselft by 0.5 cm if the side of another cube of the same material is 20cm, then under similar conditions as above the displacement will be
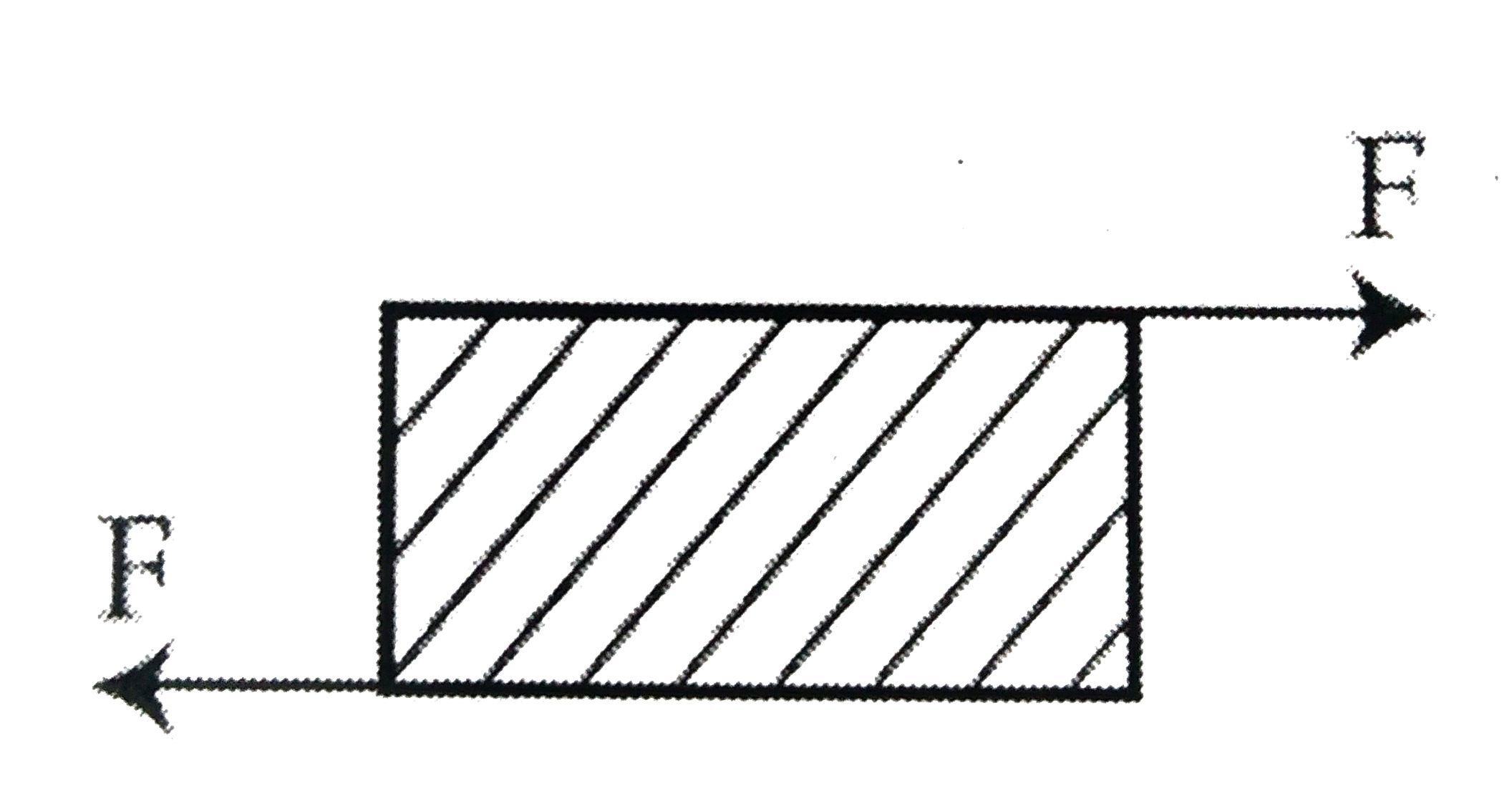
View SolutionA rubber cube of side 5cm has one side fixed while a tangential force equal to 1800N is applied to opposite face. Find the shearing strain and the lateral displacement of the strained face. Modulus of rigidity for rubber is 2.4×106N/m2.
View SolutionA tangential force equal to the weight of 500kg is applied to the opposite face of a cube of aluminium of side 10cm has its one face fixed. Find the shearing strain produced. Rigidity modulus of aluminium is 2.5×1010Nm−2
View SolutionA lead cube of side 20 cm is subjected under a shearing force of magnitude 5.6x106N. If the lower face of the cube is fixed, then the displacement in the upper face is (If shear modulus of lead is 5.6⋅106Nm2)
View SolutionA metal cube of side 20cm is subjected to a shearing force of 4000N. The top face is displaced through 0.50 cm with respect to the bottom. Find the rigidity modulus of the metal.
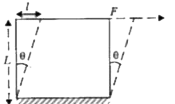
View SolutionA cube of sponge rubber with edge length 5cm has a force of 2N applied horizontally to the top face (parallel to an edge) while the bottom face is held fixed. If the top face is displaced horizontally through a distance of 1mm, find the shear modulus for the sponge rubber. (in Nm2)
View Solution
