Keeping the voltage of the charging source constant, what would be the percentage change in the energy stored in a parallel plate capcitor, if the separation between in plates were to be decreased by 10% ?
To solve the problem of finding the percentage change in the energy stored in a parallel plate capacitor when the separation between the plates is decreased by 10%, we can follow these steps:
Step 1: Understand the formula for energy stored in a capacitor
The energy (U) stored in a parallel plate capacitor is given by the formula:
U=12CV2
where C is the capacitance and V is the voltage.
Step 2: Write the expression for capacitance
The capacitance C of a parallel plate capacitor is given by:
C=ε0Ad
where:
- ε0 is the permittivity of free space,
- A is the area of the plates,
- d is the separation between the plates.
Step 3: Substitute capacitance into the energy formula
Substituting the expression for capacitance into the energy formula, we get:
U=12(ε0Ad)V2=12ε0AV2d
Step 4: Analyze the change in separation
If the separation d is decreased by 10%, the new separation d′ can be expressed as:
d′=d−0.1d=0.9d
Step 5: Calculate the new energy stored
Substituting d′ into the energy formula:
U′=12ε0AV2d′=12ε0AV20.9d=12ε0AV2d⋅10.9=U⋅10.9
Step 6: Determine the percentage change in energy
Now, we can find the percentage change in energy:
Percentage change=(U′−UU)×100%
Substituting U′:
=⎛⎝U⋅10.9−UU⎞⎠×100%
=⎛⎝10.9−11⎞⎠×100%
=(1−0.90.9)×100%
=(0.10.9)×100%
≈11.11%
Conclusion
The percentage change in the energy stored in the capacitor when the separation between the plates is decreased by 10% is approximately 11.11% increase.
---
The energy (U) stored in a parallel plate capacitor is given by the formula:
U=12CV2
where C is the capacitance and V is the voltage.
The capacitance C of a parallel plate capacitor is given by:
C=ε0Ad
where:
- ε0 is the permittivity of free space,
- A is the area of the plates,
- d is the separation between the plates.
Substituting the expression for capacitance into the energy formula, we get:
U=12(ε0Ad)V2=12ε0AV2d
If the separation d is decreased by 10%, the new separation d′ can be expressed as:
d′=d−0.1d=0.9d
Substituting d′ into the energy formula:
U′=12ε0AV2d′=12ε0AV20.9d=12ε0AV2d⋅10.9=U⋅10.9
Now, we can find the percentage change in energy:
Percentage change=(U′−UU)×100%
Substituting U′:
=⎛⎝U⋅10.9−UU⎞⎠×100%
=⎛⎝10.9−11⎞⎠×100%
=(1−0.90.9)×100%
=(0.10.9)×100%
≈11.11%
The percentage change in the energy stored in the capacitor when the separation between the plates is decreased by 10% is approximately 11.11% increase.
Topper's Solved these Questions
- BOOK - VMC MODULES ENGLISHCHAPTER - CAPACITORSEXERCISE - LEVEL 0 (LONG ANSWER TYPE)3videos
CAPACITORS
- BOOK - VMC MODULES ENGLISHCHAPTER - CAPACITORSEXERCISE - LEVEL 1 (MCQs)60videos
CAPACITORS
- BOOK - VMC MODULES ENGLISHCHAPTER - CAPACITORSEXERCISE - JEE Advance ( Archive ) LEVEL 481videos
CAPACITORS
- BOOK - VMC MODULES ENGLISHCHAPTER - BASIC MATHEMATICS & VECTORSEXERCISE - Impeccable50videos
BASIC MATHEMATICS & VECTORS
- BOOK - VMC MODULES ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - IN-CHAPTER EXERCISE-F10videos
CURRENT ELECTRICITY
Similar Questions
What is the area of the plates of a 2 farad parallel plate air capacitor, given that the separation between the plates is 0.5 cm?
View SolutionWhat is the area of the plates of a 2 farad parallel plate air capacitor, given that the separation between the plates is 0.5 cm?
View SolutionUsing the concept of energy density, find the total energy stored in a
a. parallel plate capacitor
b. charged spherical conductor.View SolutionThe separation between the plates of a charged parallel-plate capacitor is increased. The force between the plates
View SolutionA parallel plate capacitor is charged from a cell and then isolated from it. The separation between the plates is now increased
View SolutionA parallel plate capacitor is charged from a cell and then isolated from it. The separation between the plates is now increased
View SolutionWould the charge change if the plates were pulled without battery ?
View SolutionConsider the arrangement of three plates X,Y and Z each of the area A and separation d. Find the energy stored when the plates are fully charged.
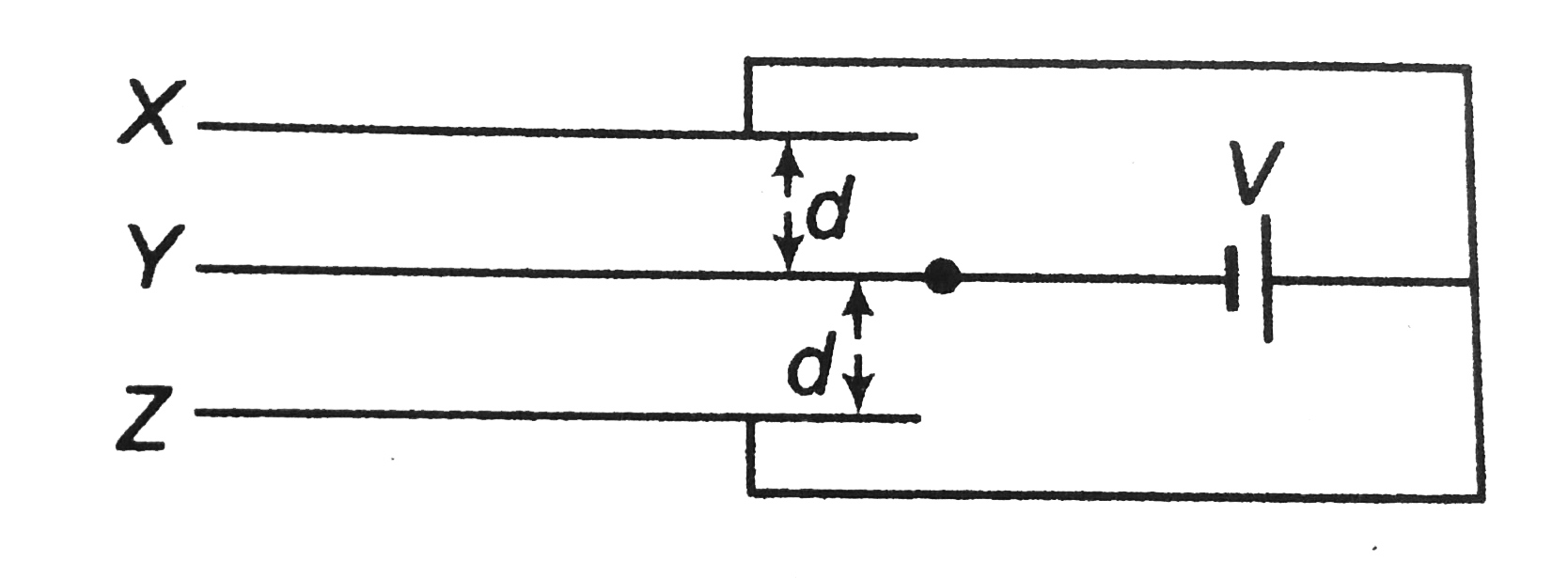 View Solution
View SolutionThe separation between the plates of a charged parallel-plate capacitor is increased. Which of the following quantities will change?
View SolutionA parallel plate capacitor of capacity C0 is charged to a potential V0,E1 is the energy stored in the capacitor when the battery is disconnected and the plate separation is doubled, and E2 is the energy stored in the capacitor when the charging battery is kept connected and the separation between the capacitor plates is dounled. find the ratio E1/E2.
View Solution

