Will current flow more easily through a thick wire or a thin wire of the same material when connected to the same source ? Why ?
To determine whether current will flow more easily through a thick wire or a thin wire of the same material when connected to the same source, we can analyze the relationship between resistance and cross-sectional area.
1. Understanding Current Flow:
Current (I) is the flow of electric charge. The amount of current that flows through a conductor depends on the resistance (R) of that conductor and the voltage (V) applied across it, according to Ohm's Law:
I=VR
2. Resistance and Cross-Sectional Area:
The resistance of a wire is influenced by its material, length (L), and cross-sectional area (A). The formula for resistance is given by:
R=ρLA
where ρ is the resistivity of the material.
3. Comparing Thick and Thin Wires:
- A thick wire has a larger cross-sectional area (A) compared to a thin wire.
- Since resistance (R) is inversely proportional to the cross-sectional area (A), a thick wire will have a lower resistance than a thin wire:
Rthick<Rthin
4. Effect on Current:
Given that the voltage (V) is the same for both wires when connected to the same source, we can substitute the resistances into Ohm's Law:
Ithick=VRthickandIthin=VRthin
Since Rthick<Rthin, it follows that:
Ithick>Ithin
This means that more current will flow through the thick wire than through the thin wire.
5. Conclusion:
Therefore, we conclude that current will flow more easily through a thick wire than through a thin wire of the same material when connected to the same voltage source. This is primarily due to the lower resistance of the thick wire.
Current (I) is the flow of electric charge. The amount of current that flows through a conductor depends on the resistance (R) of that conductor and the voltage (V) applied across it, according to Ohm's Law:
I=VR
The resistance of a wire is influenced by its material, length (L), and cross-sectional area (A). The formula for resistance is given by:
R=ρLA
where ρ is the resistivity of the material.
- A thick wire has a larger cross-sectional area (A) compared to a thin wire.
- Since resistance (R) is inversely proportional to the cross-sectional area (A), a thick wire will have a lower resistance than a thin wire:
Rthick<Rthin
Given that the voltage (V) is the same for both wires when connected to the same source, we can substitute the resistances into Ohm's Law:
Ithick=VRthickandIthin=VRthin
Since Rthick<Rthin, it follows that:
Ithick>Ithin
This means that more current will flow through the thick wire than through the thin wire.
Therefore, we conclude that current will flow more easily through a thick wire than through a thin wire of the same material when connected to the same voltage source. This is primarily due to the lower resistance of the thick wire.
Topper's Solved these Questions
Similar Questions
An electric current is passed through a circuit containing two wires of the same material connected in parallel. If the lengths and radii of the wire are in the ratio 43 and 23, then the ratio of the currents passing through the wires will be
View SolutionAssertion: Through the same current flows through the line wires and the filament of the bulb but heat produced in the filament is much higher than that in line wires.
Reason: The filament of bulbs is made of a material of high resistance and high melting point.
View Solution
Knowledge Check
If a current of 1.5 ampere flows through a metallic wire for 3 hours, then how many electrons would flow through the wire?
A2.25×1022 electronsB1.13×1023 electronsC1.01×1023 electronsD4.5×1023 electronsThe conduction current is the same as displacement current when the source is
Aac onlyBdc onlyCeither ac or dcDneither dc nor ac
Two heating coils, one of fine wire and the other of thick wire of the same material and of the same length are connected in series and in parallel. Which of the following statement is correct ?
View SolutionA current .I. flows through a metallic wire of radius .r. and the free electrons in it drift with a velocity vd. Calculate the drift velocity of the free electrons through the wire of the same material, having double the radius, when same current flows through it.
View SolutionIf a current of 0.5A flows through a metallic wire for 2 hours, then how many electrons would flow through the wire ?
View SolutionIf a current of 0.5A flows through a metallic wire for 2 hours, then how many electrons would flow through the wire ?
View SolutionA current of 4 A flows in a coil when it is connected to a 12V dc source. When the same coil is connected to an a.c. source (12 V, 8 Hz), a current of 2.4 A flows in the coil. Calculate coefficient of self inductance (L) of the coil.
View SolutionA triangular system of mass 100 gm consisting of 3 wires of length, as shown in Fig and length of AO as 4 units, are placed in the magnetic field of 1T. The current of 1 A flows through wire AO. The wires are of same material and cross-sectional area. In which direction will the system move?
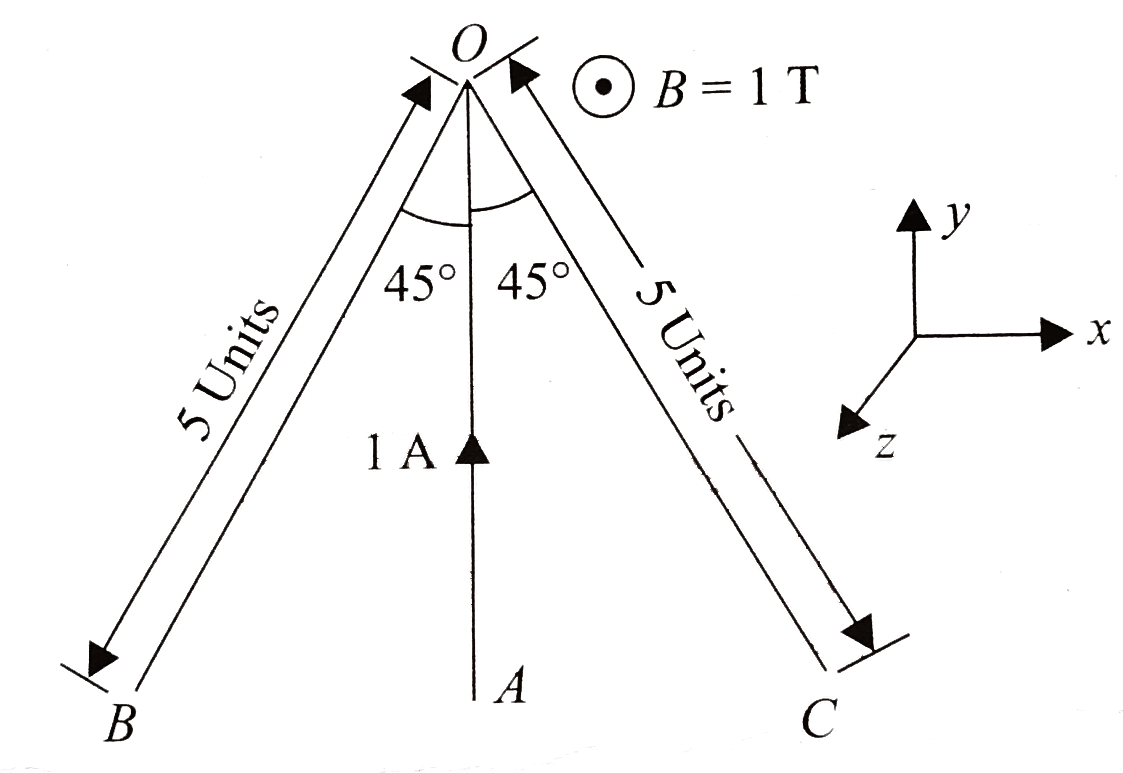
View Solution
