Question

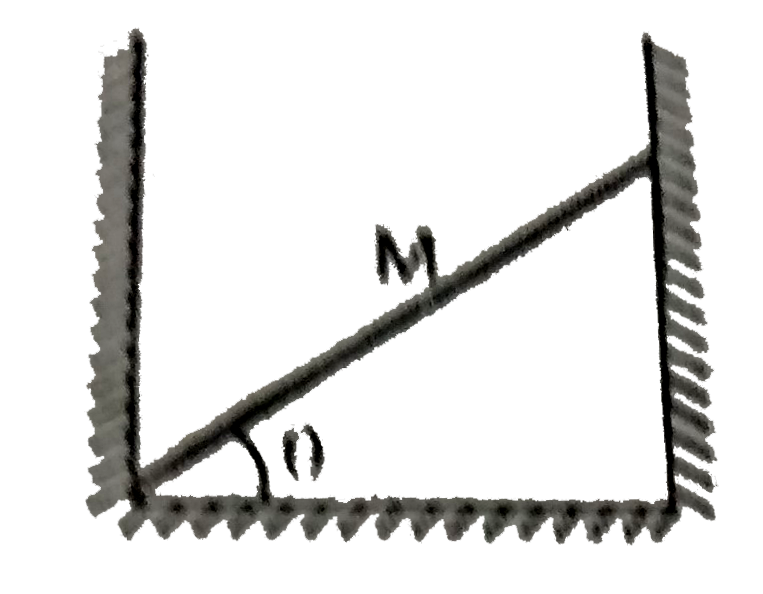
A uniform wooden stick of mass 1.6 kg and length l rests in an inclined mannar on a smooth, vertical wall of height $h(<l)$ such that a small portion of the stick extends beyond the wall. The reaction force of th wall on the stick is perpendicular to the stick. The stick makes an angle of ${30}^{\circ }$ with the wall and the bottom of the stick is on a rough floor. The reaction of the wall on the stick is equal in magnitude to the reaction of the floor on the stick. The ratio h/l and the friectional force f at the bottom of the stick are $(g=10m{s}^2)$

Options

A. `h/1 = sqrt(3)/16, f = (16sqrt(3))/3` N
B. `h/1 = 3/16, f = (16sqrt(3))/3 N`
C. `h/1 = (3sqrt(3))/16, f = (8sqrt(3))/3` N
D. `h/1 =(3sqrt(3))/16, f = (16sqrt(3))/3` N

Answer

D

Explanation

To solve the problem, we will analyze the forces and torques acting on the wooden stick. Here’s a step-by-step breakdown of the solution:

Step 1: Identify the Forces Acting on the Stick
- The weight of the stick (W = mg = 1.6 kg * 10 m/s² = 16 N) acts downward at the center of the stick.
- The normal force from the wall (N₁) acts perpendicular to the stick.
- The normal force from the floor (N₂) acts upward at the bottom of the stick.
- The frictional force (f) acts horizontally at the bottom of the stick.

Step 2: Set Up the Geometry
- The stick makes an angle of \(30^\circ\) with the wall.
- The height of the wall is \(h\), and the length of the stick is \(l\).
- The height of the stick above the floor can be expressed in terms of \(h\) and \(l\).

Step 3: Torque Balance
- Choose the point where the stick touches the floor as the pivot point (O).
- The torque due to the weight of the stick (W) is given by:
\( \text{Torque due to W} = W \cdot \left(\frac{l}{2} \cdot \cos(30^\circ)\right) = 16 \cdot \left(\frac{l}{2} \cdot \frac{\sqrt{3}}{2}\right) = 4l\sqrt{3} \)
- The torque due to the normal force from the wall (N₁) is:
\( \text{Torque due to N₁} = N₁ \cdot \left(\frac{2h}{\sqrt{3}}\right) \)
- Setting the net torque to zero:
\( N₁ \cdot \left(\frac{2h}{\sqrt{3}}\right) = 4l\sqrt{3} \)

Step 4: Force Balance in Vertical Direction
- The sum of vertical forces must equal zero:
\( N₂ - W = 0 \implies N₂ = W = 16 \text{ N} \)

Step 5: Force Balance in Horizontal Direction
- The sum of horizontal forces must equal zero:
\( f - N₁ \cdot \sin(30^\circ) = 0 \implies f = N₁ \cdot \frac{1}{2} \)

Step 6: Relate Normal Forces
- Since the normal force from the wall is equal to the normal force from the floor (N₁ = N₂):
\( N₁ = 16 \text{ N} \)

Step 7: Calculate Frictional Force
- Substitute \(N₁\) into the equation for friction:
\( f = 16 \cdot \frac{1}{2} = 8 \text{ N} \)

Step 8: Calculate Height to Length Ratio
- From the torque balance equation:
\( 16 \cdot \frac{2h}{\sqrt{3}} = 4l\sqrt{3} \implies 32h = 4l \cdot 3 \implies h = \frac{3l}{8} \)
- Therefore, the ratio \( \frac{h}{l} \):
\( \frac{h}{l} = \frac{3}{8} \)

Final Answers
- The ratio \( \frac{h}{l} = \frac{3}{8} \)
- The frictional force \( f = 8 \text{ N} \)