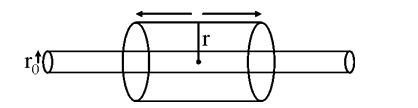
An infinite cylinder of radius $r_{o},$ carrying linear charge density $λ .$ The equation of the equipotential surface for the cylinder is

Video Solution

Text Solution

Verified by Experts

There is an infinite cylinder of radius $r_{0}$ and having linear charge density $λ$ . Assume a Gaussian surface of radius r and length l. According of Gauss’s theorem,
$\int E . d s = \frac{q}{e_{0}}$
$\int E . d s = \frac{λ 1}{e_{0}} (∵ q = λ 1)$ ( $∵$ Angle between E and ds is zero.)
$E \int d S = E .2 π r l = \frac{λ l}{e_{0}}$ [ where $(2 π r l)$ is area of the curved surface of the cylinder]
$E = \frac{λ}{2 π e_{0} r}$ ..........(i)
If the radius is $r_{0}$ , to find the potential difference at distance r from the line, consider the electric field. According to the formula of potential gradient,
$V_{r} - V_{r_{0}} = \int_{r_{0}}^{r} \frac{λ}{2 π e_{0} r} . d r$ (angle between E and dr to zero)
$= - \frac{λ}{2 π e_{0}} \int_{r_{0}}^{r} \frac{d r}{r} = - \frac{λ}{2 π e_{0}} . {[\log_{e} r]}_{r_{0}}^{r} = \frac{- λ}{2 π e_{0}} [\log_{e} r - \log_{e} r_{0}] = \frac{λ}{2 π e_{0}} . [\log_{e} r_{0} - \log_{e} r] = \frac{λ}{2 π e_{0}} . \frac{{\log r}_{0}}{r}$
$\frac{\log_{e} r_{0}}{r} = \frac{2 π r_{0}}{λ} [V_{r} - V_{r_{0}}], \frac{\log_{e} r}{r_{0}} = - \frac{2 π e_{0}}{λ} [V_{r} - V_{r_{0}}]$
$\frac{r}{r_{=}} e^{\frac{π e_{□}}{λ}} [V_{r} - V_{r_{0}}], r = r_{0} e^{\frac{2 π e_{0}}{λ}} [V_{r} - V_{r_{0}}]$
This is the equation of required equipotential surfaces.

Updated on:21/07/2023

|

Class 12 PHYSICS ELECTRIC CHARGES AND FIELDS

Topper's Solved these Questions

Similar Questions

VMC MODULES ENGLISH-ELECTROSTATICS-LEVEL - 0 (SHORT ANSWER TYPE )

Home
Ask Doubt
Profile