A 30kg block rests on a rough horizontal surface A force of 200N is applied on the block The block acquires a speed of 4m/s starting from rest in 2s What is the value of coefficient of friction ? .
10/√3
√3/10
0.47
0.185
The correct Answer is:3
To solve the problem step by step, we will follow these steps:
Step 1: Identify the given data
- Mass of the block, m=30kg
- Applied force, F=200N
- Final velocity, v=4m/s
- Initial velocity, u=0m/s
- Time, t=2s
Step 2: Calculate the acceleration of the block
Using the formula for acceleration:
a=v−ut
Substituting the values:
a=4m/s−0m/s2s=42=2m/s2
Step 3: Apply Newton's second law of motion
According to Newton's second law:
Fnet=m⋅a
The net force acting on the block is the applied force minus the frictional force:
Fnet=F−Ffriction=F−μ⋅N
Where N (normal force) is equal to m⋅g:
N=m⋅g=30kg⋅10m/s2=300N
Thus, the frictional force can be expressed as:
Ffriction=μ⋅N=μ⋅300N
Step 4: Set up the equation for net force
Now we can set up the equation:
F−μ⋅N=m⋅a
Substituting the known values:
200N−μ⋅300N=30kg⋅2m/s2
This simplifies to:
200−300μ=60
Step 5: Solve for the coefficient of friction μ
Rearranging the equation gives:
200−60=300μ
140=300μ
μ=140300=1430=715≈0.467
Step 6: Final result
The coefficient of friction μ is approximately 0.47.
- Mass of the block, m=30kg
- Applied force, F=200N
- Final velocity, v=4m/s
- Initial velocity, u=0m/s
- Time, t=2s
Using the formula for acceleration:
a=v−ut
Substituting the values:
a=4m/s−0m/s2s=42=2m/s2
According to Newton's second law:
Fnet=m⋅a
The net force acting on the block is the applied force minus the frictional force:
Fnet=F−Ffriction=F−μ⋅N
Where N (normal force) is equal to m⋅g:
N=m⋅g=30kg⋅10m/s2=300N
Thus, the frictional force can be expressed as:
Ffriction=μ⋅N=μ⋅300N
Now we can set up the equation:
F−μ⋅N=m⋅a
Substituting the known values:
200N−μ⋅300N=30kg⋅2m/s2
This simplifies to:
200−300μ=60
Rearranging the equation gives:
200−60=300μ
140=300μ
μ=140300=1430=715≈0.467
The coefficient of friction μ is approximately 0.47.
Topper's Solved these Questions
- LAWS OF MOTION BOOK - VMC MODULES ENGLISHCHAPTER - LAWS OF MOTION EXERCISE - ENABLE
50 Videos
- LAWS OF MOTION BOOK - VMC MODULES ENGLISHCHAPTER - LAWS OF MOTION EXERCISE - EFFICIENT
50 Videos
- KINEMATICS OF A PARTICLE BOOK - VMC MODULES ENGLISHCHAPTER - KINEMATICS OF A PARTICLE EXERCISE - JEE Advanced (archive)
14 Videos
- LIQUIDSBOOK - VMC MODULES ENGLISHCHAPTER - LIQUIDSEXERCISE - JEE ADVANCED (LEVEL -2)
55 Videos
Similar Questions
A block of mass 2 kg rests on a horizontal surface. If a horizontal force of 5 N is applied on the block, the frictional force on it is (take,uk=0.4,μs=0.5)
View SolutionA block of mass 10 kg is placed on the rough horizontal surface. A pulling force F is acting on the block which makes an angle θ above the horizontal. If coefficient of friction between block and surface is 43 then minimum value of force required to just move the block is (g=10ms2)
View Solution
Knowledge Check
A cubical box of side L rests on a rough horizontal surface with coefficient of friction p. A horizontal force F is a applied on the block as shown in Fig. 15.4.6. If the coefficient of friction is sufficiently high so that the block does not slide before toppling, the minimum force required to topple the block is
 AinfinitesimalBmg/4Cmg/2Dmg (−1μ)
AinfinitesimalBmg/4Cmg/2Dmg (−1μ)
A block A of mass 2 kg rests on a horizontal surface. Another block B of mass 1 kg moving at a speed of m/s when at a distance of 16 cm from A. collides elastically with A. The coefficeint of friction between the horizontal surface and earth of the blocks is 0.2. Then (g=10m/s2)
View SolutionA block of unknown mass is at rest on a rough, horizontal surface. A horizontal force F is applied to the block. The graph in the figure shows the acceleration of the block with respect to the applied force. The mass of the block is
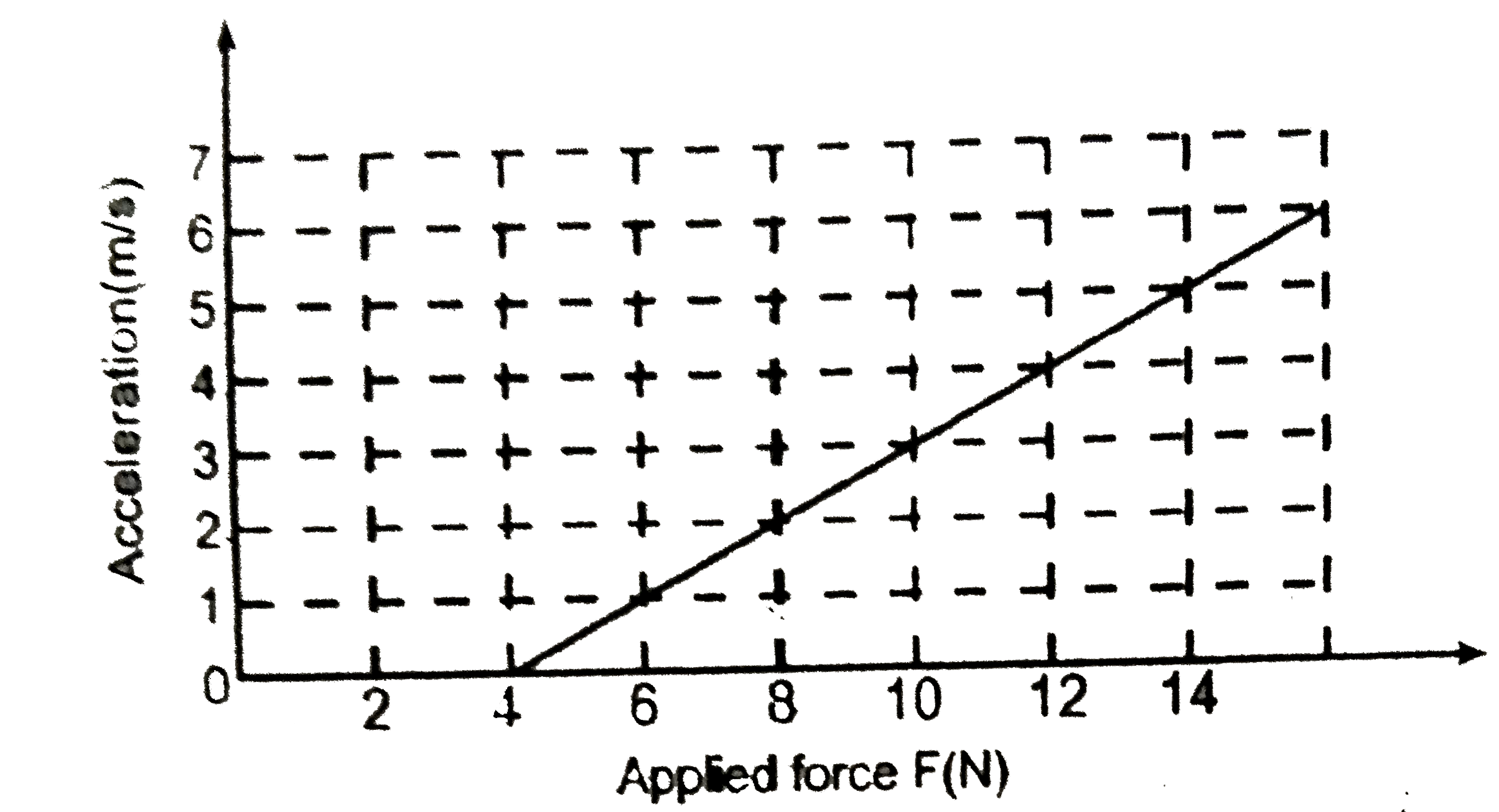
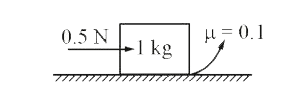
View SolutionA block of mass 1 kg in kept on a rough horizontal surface with μs=0.1 . A force of 0.5 N is applied as shown in the figure. What is the frictional force applied by ground on the block?
View SolutionA block is sliding on a rough horizontal surface. If the contact force on the block is √2 times the frictional force, the coefficient of friction is
View SolutionA block of 10kg is pulled at a constant speed on a rough horizontal plane by a force of 20N. Calculate the coefficient of friction ?
View SolutionA block of mass 10 kg is kept over a rough surface and a force F=4t is applied on it. At what value of t the block will start moving :-
View SolutionTwo blocks A and B are connected by a massless string (shown in figure) A force of 30 N is applied on block B. The distance travelled by centre of mass in 2s starting from rest is :
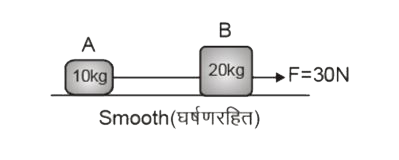
View Solution
