As body of mass 5 kg is suspended by the stirngs making angles 60∘ and 30∘ with the horizontal :
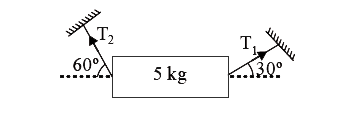
(a) T1=25N (b) T2=25N
( c ) T1=25√3N ( d ) T2=25√3N
(A)a,b,
(B)a,d
(C)c,d
(D)b,c
The correct Answer is:2
Step by step video & image solution for As body of mass 5 kg is suspended by the stirngs making angles 60^(@) and 30^(@) with the horizontal : (a) T_(1) = 25N (b) T_(2) = 25 N ( c ) T_(1) = 25 sqrt( 3) N ( d ) T_(2) = 25 sqrt( 3) N by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- LAWS OF MOTION BOOK - VMC MODULES ENGLISHCHAPTER - LAWS OF MOTION EXERCISE - EFFICIENT
50 Videos
- LAWS OF MOTION BOOK - VMC MODULES ENGLISHCHAPTER - LAWS OF MOTION EXERCISE - IMPECCABLE
53 Videos
- LAWS OF MOTION BOOK - VMC MODULES ENGLISHCHAPTER - LAWS OF MOTION EXERCISE - IMPECCABLE
53 Videos
- KINEMATICS OF A PARTICLE BOOK - VMC MODULES ENGLISHCHAPTER - KINEMATICS OF A PARTICLE EXERCISE - JEE Advanced (archive)
14 Videos
- LIQUIDSBOOK - VMC MODULES ENGLISHCHAPTER - LIQUIDSEXERCISE - JEE ADVANCED (LEVEL -2)
55 Videos
Similar Questions
If (12−t1)+(22−t2)+......+(n2−tn)=13n(n2−1), then tn is
View SolutionIn the figure shown, m1 = 10kg, m2 = 6kg, m3 = 4kg. If T3 = 40 N, T2 = ?
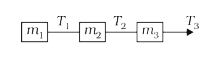
View SolutionIf n∑r=1Tr=(n+1)(n+2)(n+3) then find n∑r=11Tr
View SolutionIf for a sequence (Tn),(i)Sn=2n2+3n+1(ii)Sn=2(3n−1) find Tn and hence T1 and T2
View SolutionIf √5n=125, then 5n√64=
(a) 25
(b) 1125
(c) 625
(d) 15
View SolutionEvaluate :
(i) i135
(ii) i−47
(iii) (−√−1)4n+3,n∈N
(iv) √−25+3√−4+2√−9
View SolutionFind the 10thand nthterms of the G.P. 5, 25, 125, . . .
View SolutionIf n∑r−1Tr=n(n+1)(n+2)3,thenlimx→∞n∑r=12008Tr=
View SolutionIf n∑r−1Tr=n(n+1)(n+2)3,thenlimx→∞n∑r=13012Tr=
View SolutionThe sum Sn where Tn=(−1)nn2+n+1n! is
View Solution