The mass of an elevator is 4000 kg. Starting from rest how much distance will it rise in 2s, if the tension in the cable is 56kN ?
3m
4m
8m
16m
The correct Answer is:3
To solve the problem, we will follow these steps:
Step 1: Identify the given data
- Mass of the elevator (m) = 4000 kg
- Tension in the cable (T) = 56 kN = 56,000 N
- Time (t) = 2 s
- Acceleration due to gravity (g) = 10 m/s² (assuming standard value)
Step 2: Calculate the weight of the elevator
The weight (W) of the elevator can be calculated using the formula:
W=m⋅g
W=4000kg⋅10m/s2=40,000N
Step 3: Calculate the net force acting on the elevator
The net force (F_net) can be calculated by subtracting the weight of the elevator from the tension in the cable:
Fnet=T−W
Fnet=56,000N−40,000N=16,000N
Step 4: Calculate the acceleration of the elevator
Using Newton's second law, we can find the acceleration (a) of the elevator:
Fnet=m⋅a
16,000N=4000kg⋅a
a=16,000N4000kg=4m/s2
Step 5: Calculate the distance traveled by the elevator
Since the elevator starts from rest, the initial velocity (u) is 0. We can use the equation of motion to find the distance (s):
s=u⋅t+12at2
Substituting the known values:
s=0⋅2+12⋅4⋅(2)2
s=0+12⋅4⋅4
s=0+8=8m
Final Answer
The distance the elevator will rise in 2 seconds is 8 meters.
---
- Mass of the elevator (m) = 4000 kg
- Tension in the cable (T) = 56 kN = 56,000 N
- Time (t) = 2 s
- Acceleration due to gravity (g) = 10 m/s² (assuming standard value)
The weight (W) of the elevator can be calculated using the formula:
W=m⋅g
W=4000kg⋅10m/s2=40,000N
The net force (F_net) can be calculated by subtracting the weight of the elevator from the tension in the cable:
Fnet=T−W
Fnet=56,000N−40,000N=16,000N
Using Newton's second law, we can find the acceleration (a) of the elevator:
Fnet=m⋅a
16,000N=4000kg⋅a
a=16,000N4000kg=4m/s2
Since the elevator starts from rest, the initial velocity (u) is 0. We can use the equation of motion to find the distance (s):
s=u⋅t+12at2
Substituting the known values:
s=0⋅2+12⋅4⋅(2)2
s=0+12⋅4⋅4
s=0+8=8m
The distance the elevator will rise in 2 seconds is 8 meters.
Topper's Solved these Questions
- LAWS OF MOTION Book:VMC MODULES ENGLISHChapter:LAWS OF MOTION Exercise:IMPECCABLE
Explore 53 Videos
- LAWS OF MOTION Book:VMC MODULES ENGLISHChapter:LAWS OF MOTION Exercise:ENABLE
Explore 50 Videos
- KINEMATICS OF A PARTICLE Book:VMC MODULES ENGLISHChapter:KINEMATICS OF A PARTICLE Exercise:JEE Advanced (archive)
Explore 14 Videos
- LIQUIDSBook:VMC MODULES ENGLISHChapter:LIQUIDSExercise:JEE ADVANCED (LEVEL -2)
Explore 55 Videos
Similar Questions
The mass of a lift if 600kg and it is moving upwards with a uniform acceleration of 2m/s2. Then the tension in the cable of the lift is :
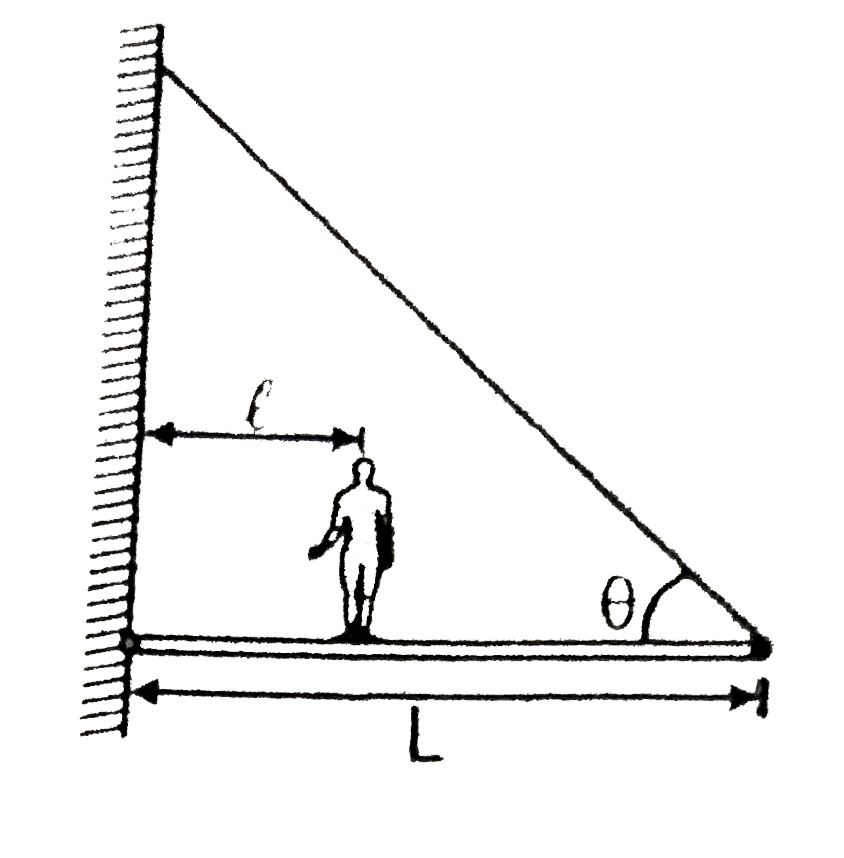
View SolutionA uniform horizontal beam of length L and mass M is attached to a wall by a pin connection. Its far end is supported by a cable that makes an angle θ with the horizontal . If a man of mass 'm' stands at a distance l from the wall , find the tension in the cable in equilibrium.
View SolutionThe total mass of an elevator with a 80 kg man in it is 1000 kg. This elevator moving upward with a speed of 8 m/sec, is brought to rest over a distance of 16m. The tension T in the cables supporting the elevator and the force exerted on the man by the elevator floor will respectively be-
View SolutionAn elevator and its load have a total mass of 800 kg. If the elevator, originally moving downwards at 10ms−1, is brought to rest with constant deceleration in a distance of 25 m, the tension in the supporting cable will be (g=10ms−2)
View SolutionAn elevator and its load have a total mass of 800 kg. If the elevator, originally moving downwards at 10ms−1, is brought to rest with constant deceleration in a distance of 25 m, the tension in the supporting canble will be (g=10ms−2)
View SolutionA wheel rotates with a constant acceleration of 2.0rads2. If the wheel starts from rest, how many revolutions will it make in the first 10 seconds?
View SolutionA particle experiences a constant acceleration for 20 sec after starting from rest. If it travels distance S1 in the first 10 sec and a distance S2 in the next 10 sec, Then
View SolutionA particle experiences a constant acceleration for 20 sec after starting from rest. If it travels distance S1 in the first 10 sec and a distance S2 in the next 10 sec, Then
View SolutionAn elevator has full load capacity 800 kg. Frictional force on the elevator is 4000 N. If elevator is going down with constant speed 2.0 m/s, then the power applied by electric motor at full load is lose to : (1 HP = 746 W, g = 10 m/s2)
View SolutionAn elevator has full load capacity 800 kg. Frictional force on the elevator is 4000 N. If elevator is going down with constant speed 2.0 m/s, then the power applied by electric motor at full load is lose to : (1 HP = 746 W, g = 10 m/s2)
View Solution
