A particle is moving along x-axis under the action of a force, F which varies with its position (x) as F∝x−1/4. The variation of power due to this force with x is
x1/3
x1/8
x1/2
t1/4
The correct Answer is:B
To solve the problem step by step, we will analyze the relationship between force, acceleration, velocity, and power as given in the question.
Step 1: Understanding the relationship between force and position
We are given that the force F varies with position x as:
F∝x−14
This means we can express the force as:
F=kx−14
where k is a proportionality constant.
Step 2: Relating force to acceleration
According to Newton's second law, force can also be expressed in terms of mass m and acceleration a:
F=ma
Since acceleration a can be expressed as a=dvdt=vdvdx, we can write:
F=mvdvdx
Step 3: Equating the two expressions for force
From the previous steps, we have:
mvdvdx=kx−14
Rearranging gives:
vdvdx=kmx−14
Step 4: Integrating to find the relationship between velocity and position
We can separate the variables and integrate:
vdv=kmx−14dx
Integrating both sides:
∫vdv=∫kmx−14dx
The left side integrates to:
v22
The right side integrates to:
km⋅(x3/43/4)=4k3mx3/4
Thus, we have:
v22=4k3mx3/4+C
For simplicity, we can ignore the constant C for finding the relationship:
v2∝x3/4
Taking the square root gives:
v∝x3/8
Step 5: Finding the expression for power
Power P is defined as the product of force and velocity:
P=F⋅v
Substituting the expressions we have:
P∝x−14⋅x38=x−14+38=x−28+38=x18
Conclusion
Thus, the variation of power due to this force with x is:
P∝x18
We are given that the force F varies with position x as:
F∝x−14
This means we can express the force as:
F=kx−14
where k is a proportionality constant.
According to Newton's second law, force can also be expressed in terms of mass m and acceleration a:
F=ma
Since acceleration a can be expressed as a=dvdt=vdvdx, we can write:
F=mvdvdx
From the previous steps, we have:
mvdvdx=kx−14
Rearranging gives:
vdvdx=kmx−14
We can separate the variables and integrate:
vdv=kmx−14dx
Integrating both sides:
∫vdv=∫kmx−14dx
The left side integrates to:
v22
The right side integrates to:
km⋅(x3/43/4)=4k3mx3/4
Thus, we have:
v22=4k3mx3/4+C
For simplicity, we can ignore the constant C for finding the relationship:
v2∝x3/4
Taking the square root gives:
v∝x3/8
Power P is defined as the product of force and velocity:
P=F⋅v
Substituting the expressions we have:
P∝x−14⋅x38=x−14+38=x−28+38=x18
Thus, the variation of power due to this force with x is:
P∝x18
Topper's Solved these Questions
- WORK ENERGY AND POWER BOOK - VMC MODULES ENGLISHCHAPTER - WORK ENERGY AND POWER EXERCISE - EFFICIENT
50 Videos
- WORK ENERGY AND POWER BOOK - VMC MODULES ENGLISHCHAPTER - WORK ENERGY AND POWER EXERCISE - IMPECCABLE
54 Videos
- WORK ENERGY AND POWER BOOK - VMC MODULES ENGLISHCHAPTER - WORK ENERGY AND POWER EXERCISE - IMPECCABLE
54 Videos
- WAVE MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - WAVE MOTIONEXERCISE - JEE ADVANCED ARCHIVE LEVEL 2 (TRUE FALSE TYPE)
4 Videos
Similar Questions
A particle is moving along x-axis under the action of force, F which varies with its position as F∝14√x. Find the variation of power due to this fore with x.
View SolutionA particle is moving along x-axis under the action of a force F, which varies with its position (x)asF∝1√x.Find the variation of power due to this force with x.
View SolutionA particle starts moving from rest along y axis and a variable force F acts on it such that F∞y−15 (y>0). The variation of power P of this force with y is given as
View SolutionFor a particle moving under the action of a variable force, kinetic energy (k) varsus position (x) graph is given, then
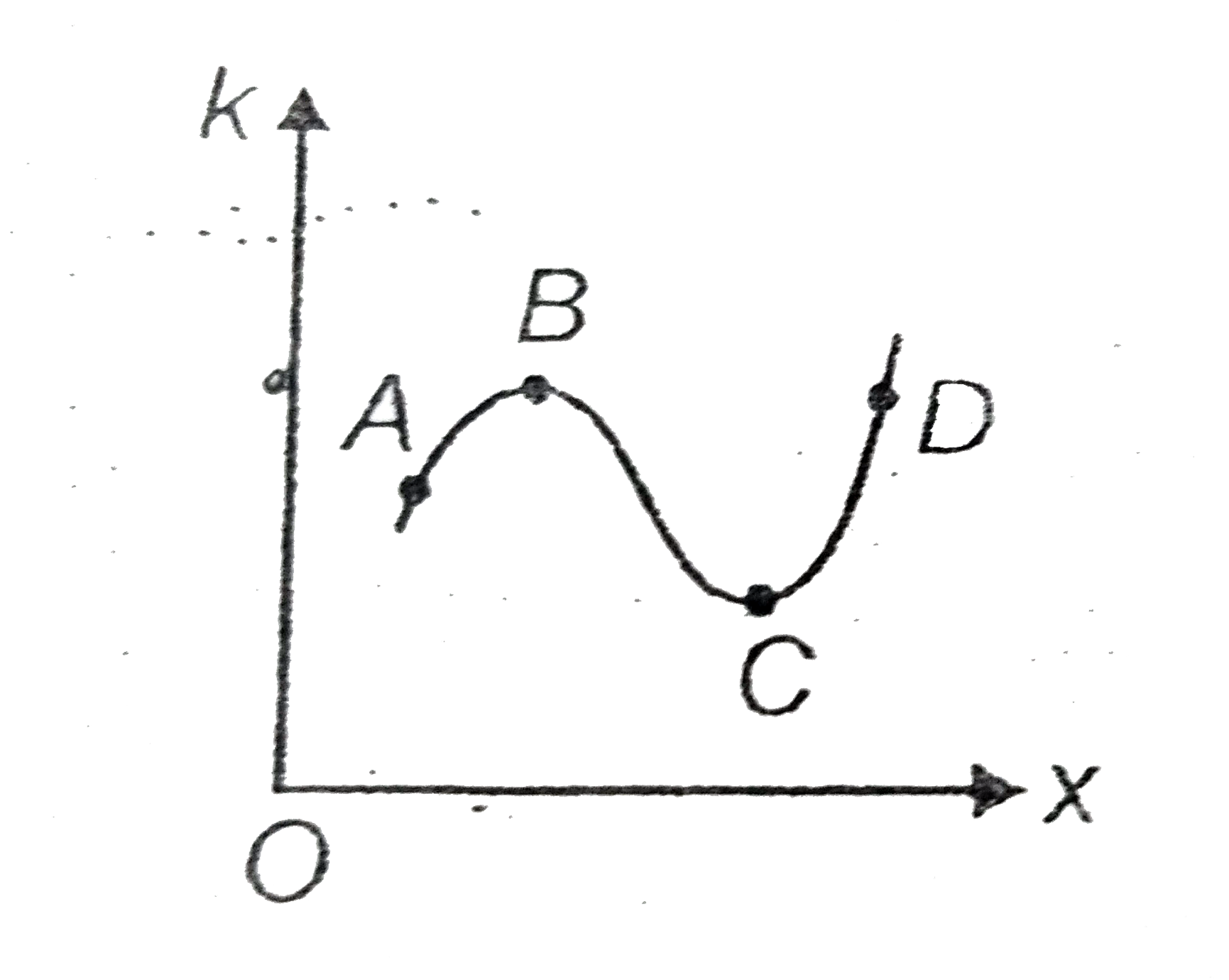
View SolutionUnder the action of a force F = Cx, the position of a body changes from 0 to x. The work done is
View SolutionFor a particle moving under the action of a variable force, kinetic energy (k) versus position (x) graph is given, then

View SolutionA particle is moving along x-axis. Its velocity v with x co-ordinate is varying as v=√x. Then
View SolutionA particle of mass m moves from rest under the action of a constant force F which acts for two seconds. The maximum power attained is
View SolutionA 4kg particle is moving along the x- axis under the action of the force F=−(π216)xN At t=2sec the particle passes through the origin and t=10sec, the speed is 4√2m/s The amplitude of the motion is
View SolutionA particle of unit mass is moving along x-axis. The velocity of particle varies with position x as v(x). =αx−β (where α and β are positive constants and x>0 ). The acceleration of the particle as a function of x is given as
View Solution
