A dancer is standing on a rotating platform taking two sphere on her hands. If she drops down the sphere on ground, then dancer's:
angular velocity will increase
angular momentum & angular velocity both will unchange
angular momentum unchanged & angular velocity will increase
both will decrease
The correct Answer is:3
To solve the problem, we need to analyze the situation using the principles of angular momentum and moment of inertia. Here's a step-by-step breakdown:
Step 1: Understand the System
The dancer is on a rotating platform holding two spheres. When she drops one of the spheres, we need to consider the effects on angular momentum and angular velocity.
Step 2: Apply Conservation of Angular Momentum
In the absence of external torques, the angular momentum of the system must be conserved. This means that the initial angular momentum (L_initial) must equal the final angular momentum (L_final).
Step 3: Define Initial and Final Angular Momentum
1. Initial Angular Momentum (L_initial):
- Let I0 be the moment of inertia of the dancer.
- Let I1 and I2 be the moments of inertia of the two spheres.
- The initial angular momentum can be expressed as:
Linitial=(I0+I1+I2)⋅ωi
where ωi is the initial angular velocity.
2. Final Angular Momentum (L_final):
- After dropping one sphere, the moment of inertia of the system changes. Now, only the dancer and one sphere contribute to the moment of inertia:
Lfinal=(I0+I2)⋅ωf
where ωf is the final angular velocity.
Step 4: Set Initial and Final Angular Momentum Equal
Using the conservation of angular momentum:
(I0+I1+I2)⋅ωi=(I0+I2)⋅ωf
Step 5: Solve for Final Angular Velocity
Rearranging the equation gives:
ωf=(I0+I1+I2)(I0+I2)⋅ωi
Since I1 is positive, it follows that:
ωf>ωi
This indicates that the final angular velocity increases when the dancer drops the sphere.
Step 6: Conclusion on Angular Momentum and Angular Velocity
- Angular Momentum: Unchanged (conserved).
- Angular Velocity: Increases.
Thus, the correct answer is that the angular momentum remains unchanged and the angular velocity increases.
Final Answer
Angular momentum unchanged and angular velocity increases.
---
The dancer is on a rotating platform holding two spheres. When she drops one of the spheres, we need to consider the effects on angular momentum and angular velocity.
In the absence of external torques, the angular momentum of the system must be conserved. This means that the initial angular momentum (L_initial) must equal the final angular momentum (L_final).
1. Initial Angular Momentum (L_initial):
- Let I0 be the moment of inertia of the dancer.
- Let I1 and I2 be the moments of inertia of the two spheres.
- The initial angular momentum can be expressed as:
Linitial=(I0+I1+I2)⋅ωi
where ωi is the initial angular velocity.
- After dropping one sphere, the moment of inertia of the system changes. Now, only the dancer and one sphere contribute to the moment of inertia:
Lfinal=(I0+I2)⋅ωf
where ωf is the final angular velocity.
Using the conservation of angular momentum:
(I0+I1+I2)⋅ωi=(I0+I2)⋅ωf
Rearranging the equation gives:
ωf=(I0+I1+I2)(I0+I2)⋅ωi
Since I1 is positive, it follows that:
ωf>ωi
This indicates that the final angular velocity increases when the dancer drops the sphere.
- Angular Momentum: Unchanged (conserved).
- Angular Velocity: Increases.
Angular momentum unchanged and angular velocity increases.
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - EFFICIENT
50 Videos
- SYSTEM OF A PARTICLES & ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF A PARTICLES & ROTATIONAL MOTIONEXERCISE - IN-CHAPTER EXERCISE F
10 Videos
- UNITS, MEASUREMENTS & ERRORSBOOK - VMC MODULES ENGLISHCHAPTER - UNITS, MEASUREMENTS & ERRORSEXERCISE - IN - CHAPTER EXERCISE - B
10 Videos
Similar Questions
A man is standing on a weighing machine with a block in his hand. The machine records w. When he takes the block upwards with some acceleration the machine records w1. When he takes the block down with some acceleration, the machine records w2. Then choose correct option
View SolutionIn fig. man A is standing on a movable plank while man B is standing on a stationary platform. Both are pulling the string down such that the plank moves slowly up. As a result of this the string slops through the hands of the men, find the ratio of length of the string that slips through the hands of A and B.
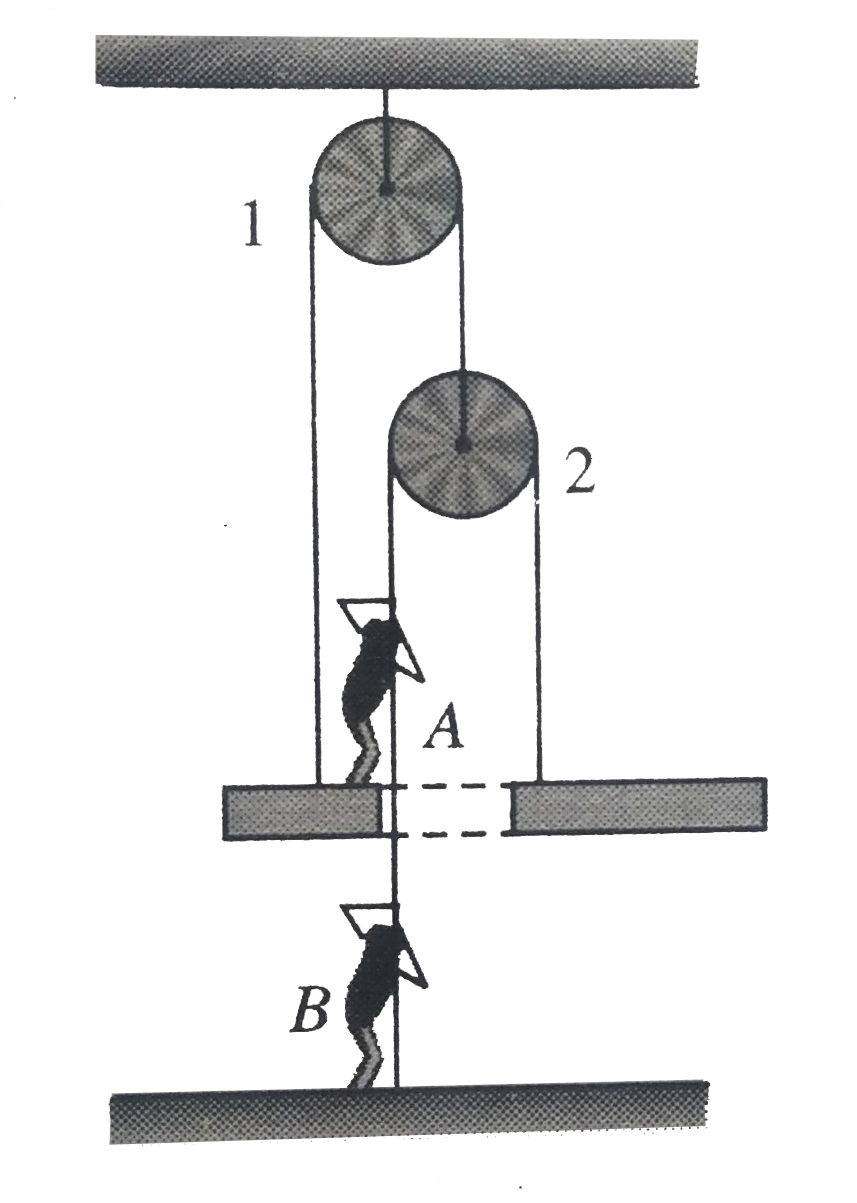
View Solution
Knowledge Check
A person is standing on a rotating table with metal spheres in his hands. If he withdraws his hands to his chest, then the effect on his angular velocity will be. a) increase b) decrease c) remain same d) can't say
AincreaseBdecreaseCremain sameDcan't sayA man stands on a rotating platform with his arms stretched holding a 5kg weight in each hand. The angular speed of the platform is 1.2revs−1. The moment of inertia of the man together with the platform may be taken to be constant and equal to 6kgm2. If the man brings his arms close to his chest with the distance n each weight from the axis changing from 100cm to20cm. The new angular speed of the platform is.
A2revs−1B3revs−1C5revs−1D6revs−1A child is standing with folded hands at the center of a platform rotating about its central axis. The kinetic energy of the system is K. The child now stretches his arms so that the moment of inertia of the system doubles. The kinetic energy of the system now is: a) K/4 b) K/2 c) 2K d) 4K
AK/4BK/2C2 KD4 K
A man of mass 62 kg is standing on a stationary boat of mass 238 kg. The man is carrying a sphere of mass 0.5 kg in his hands. If the man throws the sphere horizontally with a velocity of 12 ms−1, find the velocity with which the boat will move (in magnitude)
View SolutionA sphere and a hollow cylinder roll without slipping down two separate inclined planes and travel the same distance in the same time. If the angle of the plane down which the sphere rolls is 30∘ , the angle of the other pane is
View SolutionA man stands on a rotating platform, with his arms stretched horizontal holding a 5kg weight in each hand. The angular speed of the platform is 30 revolutions per minute. The man then brings his arms close to his body with the distance of each weight from the axis changing from 90cm to 20cm. moment of inertia of the man together with the platform may be taken to be constant and equal t 7.6kgm2. (a) What is the his new angular speed ? (Neglect friction.)
(b) Is kinetic energy conserved in the process ? If not, from where does the change come about ?
View SolutionWater from a tap, maintained at a costant potential V, is allolwed to fall by drops of radius r through a small hole into a hollow conducting sphere of radius R standing on an insulating stand until it fills the entire sphere. Find the potential of the hollow conductor after it is completely filled with water.
View SolutionA uniform solid sphere of mass M and radius R is rotating with respect to its diameter, with a constant angular velocity ω in free space (i.e., there is no one to exert any force on sphere). Consider an hemispherical part of the sphere (such that axis of rotation lies in the place dividing the sphere in two hemisphere). Net force on this hemisphere due to another hemisphere is nMω2R16. Here n is an integer. Find n
View Solution
