If I=50kgm2, then how much torque will be applied to stop it in 10 sec. Its initial angular speed is 20 rad/sec?
(a)100 Nm
(b)150 Nm
(c)200 Nm
(d)250 Nm
The correct Answer is:1
To solve the problem step by step, we will follow these calculations:
Step 1: Identify the given values
- Moment of inertia (I) = 50 kg m²
- Initial angular speed (ω) = 20 rad/s
- Time to stop (t) = 10 s
Step 2: Calculate the angular acceleration (α)
To find the angular acceleration required to stop the object, we can use the formula:
α=Δωt
Since we want to stop the object, the final angular speed (ω_f) will be 0 rad/s. Therefore, the change in angular speed (Δω) is:
Δω=ωf−ω=0−20=−20 rad/s
Now, substituting the values into the formula:
α=−20 rad/s10 s=−2 rad/s2
Step 3: Calculate the torque (τ)
Using the relationship between torque, moment of inertia, and angular acceleration:
τ=I⋅α
Substituting the known values:
τ=50 kg m2⋅(−2 rad/s2)=−100 N m
The negative sign indicates that the torque is applied in the opposite direction of the angular motion to stop it.
Step 4: Conclusion
The magnitude of the torque required to stop the object in 10 seconds is:
τ=100 N m
Final Answer
The torque that must be applied to stop it in 10 seconds is 100 N m.
---
- Moment of inertia (I) = 50 kg m²
- Initial angular speed (ω) = 20 rad/s
- Time to stop (t) = 10 s
To find the angular acceleration required to stop the object, we can use the formula:
α=Δωt
Since we want to stop the object, the final angular speed (ω_f) will be 0 rad/s. Therefore, the change in angular speed (Δω) is:
Δω=ωf−ω=0−20=−20 rad/s
Now, substituting the values into the formula:
α=−20 rad/s10 s=−2 rad/s2
Using the relationship between torque, moment of inertia, and angular acceleration:
τ=I⋅α
Substituting the known values:
τ=50 kg m2⋅(−2 rad/s2)=−100 N m
The negative sign indicates that the torque is applied in the opposite direction of the angular motion to stop it.
The magnitude of the torque required to stop the object in 10 seconds is:
τ=100 N m
The torque that must be applied to stop it in 10 seconds is 100 N m.
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - EFFICIENT
50 Videos
- SYSTEM OF A PARTICLES & ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF A PARTICLES & ROTATIONAL MOTIONEXERCISE - IN-CHAPTER EXERCISE F
10 Videos
- UNITS, MEASUREMENTS & ERRORSBOOK - VMC MODULES ENGLISHCHAPTER - UNITS, MEASUREMENTS & ERRORSEXERCISE - IN - CHAPTER EXERCISE - B
10 Videos
Similar Questions
How much force is required to stop a bus (in 10 seconds) of mass 5000 kg moving with a speed of 72 km/h?
View SolutionHow much force is required to stop a bus ( in 10 seconds) of mass 5000 kg moving with a speed of 72 km/h?
View SolutionSpeed of an object moving in cirular path of radius 10 m with angular speed 2 rad/s is
View SolutionCompute the torque acting on a wheel of moment of inertia 10kgm2, moving with angular acceleration 5rads−2.
View SolutionCompute the torque acting on a wheel of moment of inertia 10kgm2, moving with angular acceleration 5rads−2.
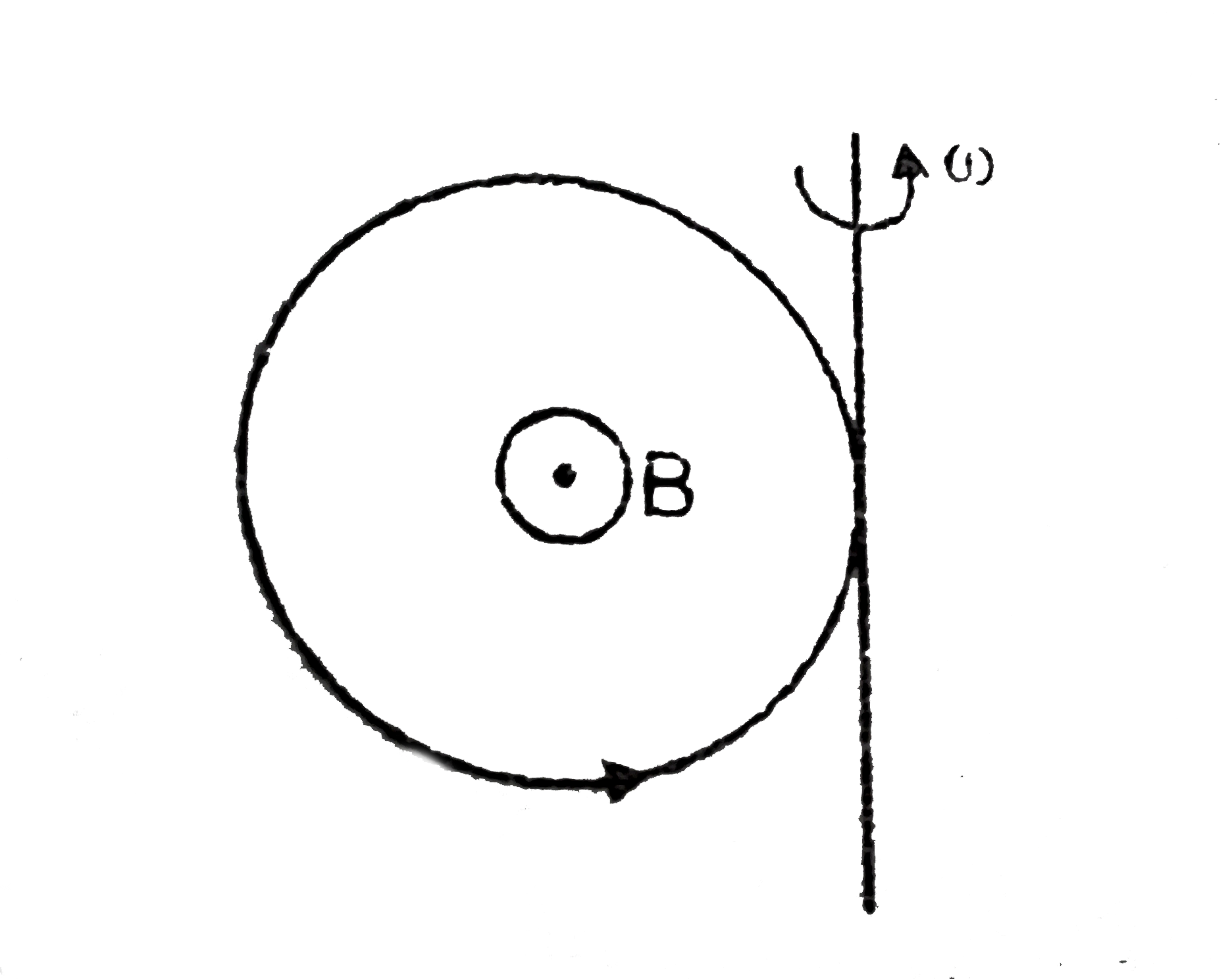
View SolutionA current carrying ring, carrying a constant current 2πAmp, radius 1m mass 23kg having 10 windings is free to rotate about its tangential vertical axis. A uniform magnetic field of 1 tesla is applied perpendicular to its plane. How much minimum angular velocity (in rad/sec) should be given to the ring in the direction shown, so that it can rotate 270∘ in that direction. Write your answer in nearest single digit in rad/sec
View SolutionA diver having a moment of inertia of 6.0kg−m2 about an axis through its centre of mass rotates at an angular speed of 2 rad/s about this axis. If he folds his hands and feet to decrease the moment of inertia to 5.0 kg-m^2 what will be the new angular speed?
View SolutionWhen a constant torque is applied to a flywheel at rest it angular velocity increases by 10 rad/s in 2 s: Calculate the torque applied if the moment of inertia of the flywheel about the axis of rotation is 200kgm2.
View SolutionAn angular impulse of 20 Nms is applied to a hollow cylinder of mass 2 kg and radius 20 cm. The change in its angular speed is :
View SolutionA wheel of moment of inertia 0.500kg−m2 and radius 20.0 cm is rotating about its axis at an angular speed of 20.0 rad/s. It picks up a stationary particle of mass 200 g at its edge. Find the new angular speed of the wheel.
View Solution
