ABC is an equilateral triangle. Length of each side is 'a' and centroid is point O. Find
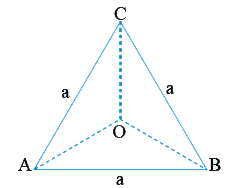
If −−→AB+−−→AC=n−−→AO then n = ?
The correct Answer is:3
−−→AB=−−→AO+−−→OBand−−→AC=−−→AO+−−→OC∴−−→AB+−−→AC=2−−→AO+−−→OB+−−→OC....(1)
but −−→OA+−−→OB+−−→OC=→0∴−−→OB+−−→OC=−−−→OA=−−→AO....(2)
by (1) and (2) −−→AB+−−→AC=2−−→AO+−−→AO −−→AB+−−→AC=3−−→AO∴n=3
Similar Questions
ABC is an equilateral triangle. Length of each side is 'a' and centroid is point O. Find
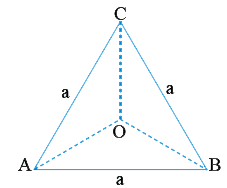
If ∣∣∣−−→AB+−−→BC+−−→AC∣∣∣=na then n = ?View SolutionABC is an equilateral triangle. Length of each side is 'a' and centroid is point O. Find
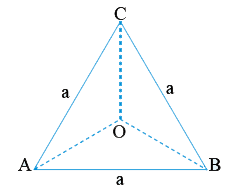
−−→AB+−−→BC+−−→CAView SolutionABC is an equilateral triangle of side 2m. If →E=10NC−1 then VA−VB is
 View Solution
View SolutionLet ABC be a right triangle with length of side AB=3 and hyotenus AC=5. If D is a point on BC such that BDDC=ABAC, then AD is equal to
View SolutionABC is an equilateral triangle with O as its centre −→F1,−→F2 and −→F3 represent three forces acting along the sides AB, BC and AC respectively. If the torque about O is zero then the magnitude of −→F3 is
 View Solution
View SolutionConsider △ABC. Let I bet he incentre and a,b,c be the sides of the triangle opposite to angles A,B,C respectively. Let O be any point in the plane of △ABC within the triangle. AO,BO and CO meet the sides BC, CA and AB in D,E and F respectively. If 3−−→BD=2−−→DC and 4−−→CE=−−→EA then the ratio in which divides −−→AB is(A)3:4(B)3:2(C)4:1(D)6:1
View SolutionOrthocenter of an equilateral triangle ABC is the origin O. If −−→OA=→a,−−→OB=→b,−−→OC=→c, then −−→AB+2−−→BC+3−−→CA=
View SolutionGiven an equilateral triangle ABC with side length equal to 'a'. Let M and N be two points respectivelyАВIn the side AB and AC such that −−→AN=K−−→ACand−−→AM=−−→AB3 If −−→BNand−−→CM are orthogonalthen the value of K is equal to
View SolutionLet ABC be a triangle having its centroid its centroid at G. If S is any point in the plane of the triangle, then −→SA+−−→SB+−−→SC=
View SolutionLet ABC be a triangle having its centroid its centroid at G. If S is any point in the plane of the triangle, then −→SA+−−→SB+−−→SC=
View Solution

