A man when standstill observes the rain falling vertically and when he walks at 4km/h he has to hold his umberella at an angle 53∘ from the vertical. Find velocity of the raindrops.
To solve the problem, we need to analyze the situation using the concepts of relative velocity. Let's break it down step by step.
Step 1: Understand the scenario
When the man is standing still, he observes the rain falling vertically. This means that the velocity of the rain with respect to the ground is purely vertical.
Step 2: Analyze the situation when the man is walking
When the man walks at a speed of 4 km/h, he has to hold his umbrella at an angle of 53 degrees from the vertical. This indicates that the rain is not falling vertically from his perspective anymore; it appears to be falling at an angle.
Step 3: Set up the relative velocity equation
Let:
- Vr = velocity of the rain with respect to the ground (vertical component)
- Vm = velocity of the man = 4 km/h (horizontal component)
- Vrm = velocity of the rain with respect to the man
From the problem, we know:
Vrm=Vr−Vm
Step 4: Determine the angle and use trigonometry
The angle at which the man holds the umbrella is 53 degrees. This means we can use trigonometric relationships to find the components of the rain's velocity.
Using the tangent of the angle:
tan(53∘)=VmVr
Step 5: Calculate tan(53∘)
From trigonometric tables, we find:
tan(53∘)≈1.327
Step 6: Substitute the known values
We know Vm=4 km/h, so we can substitute:
1.327=4Vr
Step 7: Solve for Vr
Rearranging the equation gives:
Vr=41.327
Calculating this:
Vr≈3.01 km/h
Step 8: Conclusion
Thus, the velocity of the rain with respect to the ground is approximately:
Vr≈3 km/h
Final Answer
The velocity of the raindrops is approximately 3 km/h.
---
When the man is standing still, he observes the rain falling vertically. This means that the velocity of the rain with respect to the ground is purely vertical.
When the man walks at a speed of 4 km/h, he has to hold his umbrella at an angle of 53 degrees from the vertical. This indicates that the rain is not falling vertically from his perspective anymore; it appears to be falling at an angle.
Let:
- Vr = velocity of the rain with respect to the ground (vertical component)
- Vm = velocity of the man = 4 km/h (horizontal component)
- Vrm = velocity of the rain with respect to the man
Vrm=Vr−Vm
The angle at which the man holds the umbrella is 53 degrees. This means we can use trigonometric relationships to find the components of the rain's velocity.
tan(53∘)=VmVr
From trigonometric tables, we find:
tan(53∘)≈1.327
We know Vm=4 km/h, so we can substitute:
1.327=4Vr
Rearranging the equation gives:
Vr=41.327
Vr≈3.01 km/h
Thus, the velocity of the rain with respect to the ground is approximately:
Vr≈3 km/h
The velocity of the raindrops is approximately 3 km/h.
Topper's Solved these Questions
- Motion in Straight Line BOOK - VMC MODULES ENGLISHCHAPTER - Motion in Straight Line EXERCISE - IN-CHAPTER EXERCISE-A
11 Videos
- Motion in Straight Line BOOK - VMC MODULES ENGLISHCHAPTER - Motion in Straight Line EXERCISE - IN-CHAPTER EXERCISE-B
10 Videos
- Motion in Straight Line BOOK - VMC MODULES ENGLISHCHAPTER - Motion in Straight Line EXERCISE - SOLVED EXAMPLES
20 Videos
- MOTION IN A STRAIGHT LINE & PLANE BOOK - VMC MODULES ENGLISHCHAPTER - MOTION IN A STRAIGHT LINE & PLANE EXERCISE - IMPECCABLE
52 Videos
- Motion in Two DimensionsBOOK - VMC MODULES ENGLISHCHAPTER - Motion in Two DimensionsEXERCISE - MCQ
2 Videos
Similar Questions
By the term velocity of rain, we mean velocity with which raindrops fall relative to the ground. In absence of wind, raindrops fall vertically and in presence of wind raindrops fall obliqucly. Moreover raindrops acquire a constant terminal velocity due air resistance very quickly as they fall toward the carth. A moving man relative to himself observes an altered velocity of raindrops. Which is known as velocity of rain relative to the man. It is given by the following equation.
→vrm=→vr−→vm
A standstill man relative to himself observes rain falling with velocity, which is equal to velocity of the raindrops relative to the ground. To protect himself a man should his umbrella against velocity of raindrops relative to himself as shown in the following figure.
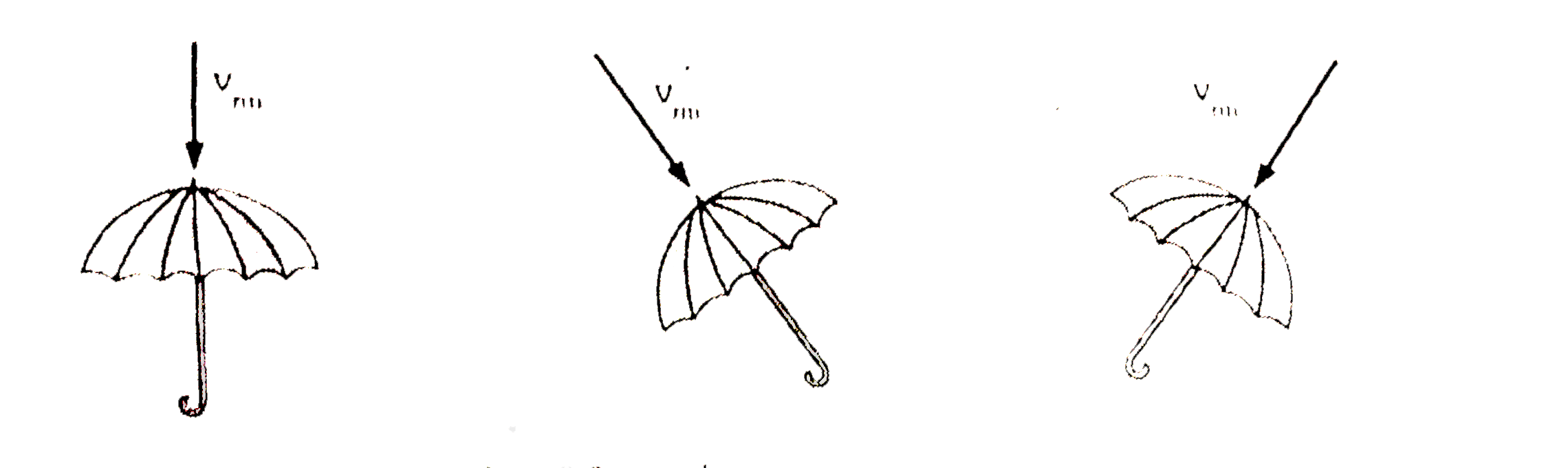
A man walks in rain at 72 cm/m due east and observes the rain falling vertically. When he stops, rain appears to strike his back at 37∘ from the vertical. Find velocity of raindrops relative to the ground.
View SolutionA person walking ,on a horizontal road at 2 km/h finds that the rain is falling vertically . Now the person increasses his speed to 4 km/h and find that rain makes an angle 45∘ with the vertical . Find the velocity of rain with respect to the road.
View SolutionA man starts running along a straight road with uniform velocity observes that the rain is falling vertically downward. If he doubles his speed, he finds that the rain is coming at an angle θ to the vertical. The velocity of rain with respect to the ground is :
View SolutionA man starts running along a straight road with uniform velocity observes that the rain is falling vertically downward. If he doubles his speed, he finds that the rain is coming at an angle θ to the vertical. The velocity of rain with respect to the ground is :
View SolutionTo a man walking at the rate of 3km/h the rain appear to fall vertically downwards. When he increases his speed 6km/h it appears to meet him at an angle of 45∘ with vertically. Find the speed of rain.
View SolutionA man walking with a speed of 3 km/h finds the rain drops falling vertically downwards. When the man increases his speed to 6km/h he find that the rain drops are falling making an angle of 30∘ with the vertical . Find the speed of the rain drops ( in km/h)
View SolutionA stationary man observes that the rain is falling vertically downwards. When he starts running a velocity of 12kmh−1, he observes that the rain is falling at an angle 60∘ with the vertical. The actual velocity of rain is
View SolutionA man running at a speed of 5 km/h finds that the rain is falling vertically. When the stops running, the finds that the rain is falling at an angle of 60∘ with the horizontal. The velocity of rain with respect to running man is
View SolutionBy the term velocity of rain, we mean velocity with which raindrops fall relative to the ground. In absence of wind, raindrops fall vertically and in presence of wind raindrops fall obliqucly. Moreover raindrops acquire a constant terminal velocity due air resistance very quickly as they fall toward the carth. A moving man relative to himself observes an altered velocity of raindrops. Which is known as velocity of rain relative to the man. It is given by the following equation.
→vrm=→vr−→vm
A standstill man relative to himself observes rain falling with velocity, which is equal to velocity of the raindrops relative to the ground. To protect himself a man should his umbrella against velocity of raindrops relative to himself as shown in the following figure.

When you are standstill in rain, you have to hold umbrella vertically to protect yourself.
(a) When you walk with velocity 90 cm/s, you have to hold your umbrella at 53∘ above the horizontal. What is velocity of the raindrops relative to the ground and relative to you ?
(b) If you walk with speed 160 cm/s, how should you hold your umbrella ?
View SolutionTo a man walking at the rate of 4 km/h the rain appears to fall vertically. When he increases his speed 8 km/h it appears to meet him at an angle of 45 with vertical. Find the angle made by the velocity of rain with the vertical and its value.
View Solution
