The second's hand of a watch has length 6 cm. Speed of end point and magnitude of difference of velocities at two perpendicular positions will be
2n & 0 mm/s
2√2n&4.44 mm/s
2√2n&2 n mm/s
2n&2√2 n mm/s
To solve the problem step by step, we need to find the speed of the endpoint of the second's hand of a watch and the magnitude of the difference of velocities at two perpendicular positions.
Step 1: Identify the parameters
The length of the second's hand (which acts as the radius of the circular path) is given as:
- Length (L) = 6 cm
Step 2: Convert length to meters
Since we need the speed in millimeters per second, we can convert the length from centimeters to meters:
- L=6 cm=0.06 m
Step 3: Calculate the circumference of the circular path
The distance traveled by the tip of the second's hand in one complete revolution is the circumference of the circle:
- Circumference (C) = 2πr=2π×0.06 m=0.12π m
Step 4: Determine the time for one complete revolution
The second's hand takes 60 seconds to complete one full revolution:
- Time (T) = 60 s
Step 5: Calculate the speed of the endpoint
Speed (v) is given by the formula:
v=DistanceTime=CT
Substituting the values:
v=0.12π m60 s=0.12π60 m/s
v=2π100 m/s
Step 6: Convert speed to millimeters per second
To convert meters per second to millimeters per second:
v=2π100 m/s=2π mm/s
Step 7: Calculate the velocity at two perpendicular positions
Let’s denote the velocities at two perpendicular positions:
- At position 1 (along the x-axis): →V1=2π^i mm/s
- At position 2 (along the y-axis): →V2=2π^j mm/s
Step 8: Calculate the difference in velocities
The difference in velocities ΔV is given by:
ΔV=→V2−→V1=2π^j−2π^i
This results in:
ΔV=−2π^i+2π^j
Step 9: Calculate the magnitude of the difference in velocities
The magnitude of ΔV is calculated as:
|ΔV|=√(−2π)2+(2π)2=√4π2+4π2=√8π2=2π√2
Final Results
- Speed of the endpoint: 2π mm/s
- Magnitude of the difference of velocities: 2π√2 mm/s
Summary
The final answers are:
- Speed of the endpoint: 2π mm/s
- Magnitude of the difference of velocities: 2π√2 mm/s
The length of the second's hand (which acts as the radius of the circular path) is given as:
- Length (L) = 6 cm
Since we need the speed in millimeters per second, we can convert the length from centimeters to meters:
- L=6 cm=0.06 m
The distance traveled by the tip of the second's hand in one complete revolution is the circumference of the circle:
- Circumference (C) = 2πr=2π×0.06 m=0.12π m
The second's hand takes 60 seconds to complete one full revolution:
- Time (T) = 60 s
Speed (v) is given by the formula:
v=DistanceTime=CT
Substituting the values:
v=0.12π m60 s=0.12π60 m/s
v=2π100 m/s
To convert meters per second to millimeters per second:
v=2π100 m/s=2π mm/s
Let’s denote the velocities at two perpendicular positions:
- At position 1 (along the x-axis): →V1=2π^i mm/s
- At position 2 (along the y-axis): →V2=2π^j mm/s
The difference in velocities ΔV is given by:
ΔV=→V2−→V1=2π^j−2π^i
This results in:
ΔV=−2π^i+2π^j
The magnitude of ΔV is calculated as:
|ΔV|=√(−2π)2+(2π)2=√4π2+4π2=√8π2=2π√2
- Speed of the endpoint: 2π mm/s
- Magnitude of the difference of velocities: 2π√2 mm/s
The final answers are:
- Speed of the endpoint: 2π mm/s
- Magnitude of the difference of velocities: 2π√2 mm/s
Topper's Solved these Questions
- Motion in Straight Line BOOK - VMC MODULES ENGLISHCHAPTER - Motion in Straight Line EXERCISE - IN-CHAPTER EXERCISE-A
11 Videos
- Motion in Straight Line BOOK - VMC MODULES ENGLISHCHAPTER - Motion in Straight Line EXERCISE - IN-CHAPTER EXERCISE-B
10 Videos
- Motion in Straight Line BOOK - VMC MODULES ENGLISHCHAPTER - Motion in Straight Line EXERCISE - SOLVED EXAMPLES
20 Videos
- MOTION IN A STRAIGHT LINE & PLANE BOOK - VMC MODULES ENGLISHCHAPTER - MOTION IN A STRAIGHT LINE & PLANE EXERCISE - IMPECCABLE
52 Videos
- Motion in Two DimensionsBOOK - VMC MODULES ENGLISHCHAPTER - Motion in Two DimensionsEXERCISE - MCQ
2 Videos
Similar Questions
Knowledge Check
A particle executes linear simple harmonic motion with an amplitude of 2 cm . When the particle is at 1 cm from the mean position the magnitude of its velocity is equal to that of its acceleration. Then its time period in seconds is
A12π√3B2π√3C2π√3D√32π
A stone tied to a string of length L is whirled in a vertical circle with the other end of the string at the centre. At a certain instant of time the stone is at lowest position and has a speed u . Find the magnitude of the change in its velocity as it reaches a position, where the string is horizontal.
View SolutionThe length of a seconds hand in watch is 1cm. The change in velocity of its tip in 15s is
View SolutionIf the length of second's hand of a clock is 10 cm, the speed of its dip (in cm 1s^(-1)) is nearly
View SolutionA conducing rod of length l is falling with a velocity v perpendicular to a unifrorm horizontal magnetic field B . The potential difference between its two ends will be
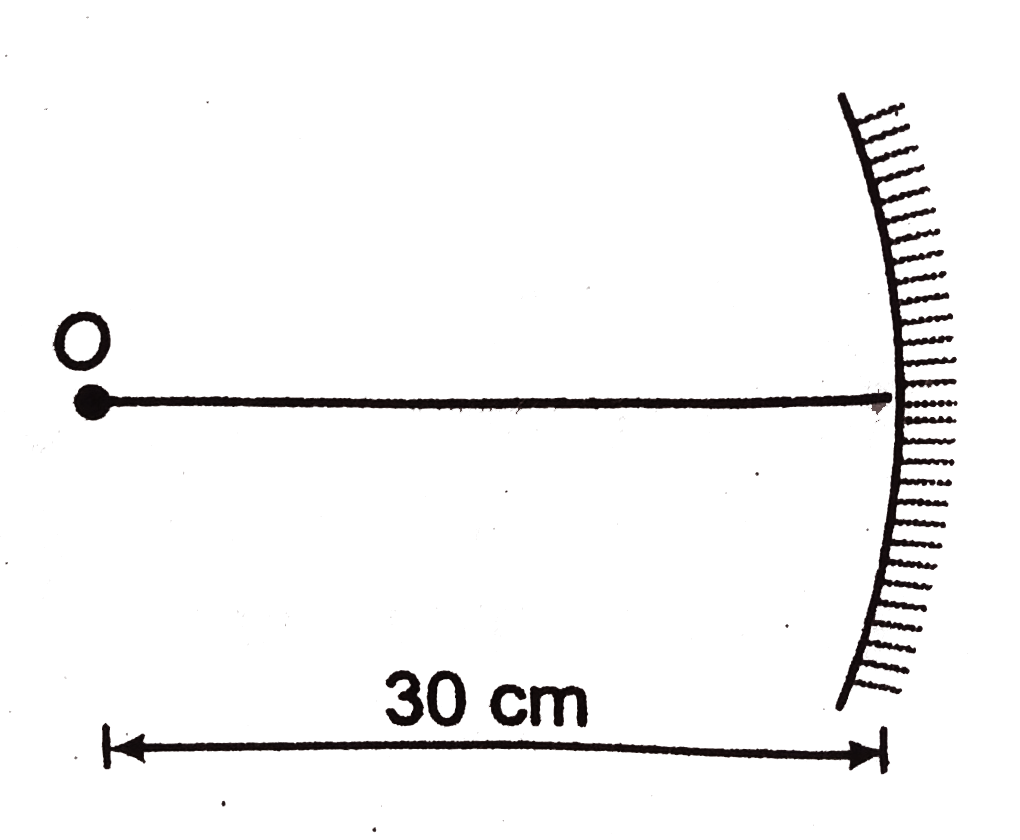
View SolutionA point object is placed on the principal axis of a concave mirror of focal length 20 cm. At this instant object is given a velocity v towards the axis (event-I) or perpendicular to axis (event-2). Then, speed of image
View SolutionA particle executes linear simple harmonic motion with an amplitude of 3cm. When the particle is at 2cm from the mean position, the magnitude of its velocity is equal to that of its acceleration. Then, its time period in seconds is
View SolutionA particle executes linear simple harmonic motion with an amplitude of 2 cm . When the particle is at 1 cm from the mean position the magnitude of its velocity is equal to that of its acceleration. Then its time period in seconds is
View Solution
