A particle is thrown up vertically with a speed v1 in air . If takes time t1 in upward journey and t2(>t1) in the downward journey and returns to the starting point with a speed v2. Then:-
v1=v2
v2>v1
v1>v2
Data is insufficient
The correct Answer is:C
To solve the problem step by step, we will analyze the motion of the particle during its upward and downward journeys, taking into account the effects of gravity and air resistance.
Step 1: Analyze the upward journey
When the particle is thrown upwards with an initial speed v1, it will experience two forces: the gravitational force acting downwards and the air resistance also acting downwards.
Using the third equation of motion:
v2=u2+2as
where:
- v = final velocity at the highest point = 0 (since it momentarily stops)
- u = initial velocity = v1
- a = acceleration = −(g+a) (negative because both gravity and air resistance oppose the motion)
- s = height reached = h
Substituting the values, we get:
0=v21−2(g+a)h
Rearranging gives:
v21=2(g+a)h(Equation 1)
Step 2: Analyze the downward journey
During the downward journey, the particle will fall under the influence of gravity, but air resistance will act in the opposite direction (upwards).
Using the same equation of motion:
v2=u2+2as
where:
- v = final velocity when it hits the ground = v2
- u = initial velocity at the highest point = 0
- a = acceleration = g−a (net acceleration downwards)
- s = height fallen = h
Substituting the values, we get:
v22=0+2(g−a)h
Rearranging gives:
v22=2(g−a)h(Equation 2)
Step 3: Compare the two equations
From Equation 1:
v21=2(g+a)h
From Equation 2:
v22=2(g−a)h
To compare v1 and v2, we can set up the inequality:
v21=2(g+a)handv22=2(g−a)h
Since g+a>g−a (because a is a positive value representing air resistance), we can conclude:
v21>v22
Taking the square root of both sides (and noting that both velocities are positive), we have:
v1>v2
Conclusion
Thus, the relationship between the speeds is:
v1>v2
When the particle is thrown upwards with an initial speed v1, it will experience two forces: the gravitational force acting downwards and the air resistance also acting downwards.
v2=u2+2as
where:
- v = final velocity at the highest point = 0 (since it momentarily stops)
- u = initial velocity = v1
- a = acceleration = −(g+a) (negative because both gravity and air resistance oppose the motion)
- s = height reached = h
0=v21−2(g+a)h
Rearranging gives:
v21=2(g+a)h(Equation 1)
During the downward journey, the particle will fall under the influence of gravity, but air resistance will act in the opposite direction (upwards).
v2=u2+2as
where:
- v = final velocity when it hits the ground = v2
- u = initial velocity at the highest point = 0
- a = acceleration = g−a (net acceleration downwards)
- s = height fallen = h
v22=0+2(g−a)h
Rearranging gives:
v22=2(g−a)h(Equation 2)
From Equation 1:
v21=2(g+a)h
From Equation 2:
v22=2(g−a)h
v21=2(g+a)handv22=2(g−a)h
v21>v22
v1>v2
Thus, the relationship between the speeds is:
v1>v2
Topper's Solved these Questions
- Motion in Straight Line BOOK - VMC MODULES ENGLISHCHAPTER - Motion in Straight Line EXERCISE - IN-CHAPTER EXERCISE-D
10 Videos
- Motion in Straight Line BOOK - VMC MODULES ENGLISHCHAPTER - Motion in Straight Line EXERCISE - IN-CHAPTER EXERCISE-E
10 Videos
- Motion in Straight Line BOOK - VMC MODULES ENGLISHCHAPTER - Motion in Straight Line EXERCISE - IN-CHAPTER EXERCISE-B
10 Videos
- MOTION IN A STRAIGHT LINE & PLANE BOOK - VMC MODULES ENGLISHCHAPTER - MOTION IN A STRAIGHT LINE & PLANE EXERCISE - IMPECCABLE
52 Videos
- Motion in Two DimensionsBOOK - VMC MODULES ENGLISHCHAPTER - Motion in Two DimensionsEXERCISE - MCQ
2 Videos
Similar Questions
Knowledge Check
The height of a tower is h meter, A body is thrown from the top of tower vertically upwards with some speed, it takes t1 seconds to reach the ground. Another body thrown from the top of tower with same speed downwards and takes t2 seconds to reach the ground. If third body, released from same place takes 't' second to reach the ground, then
At=t1+t22Bt=t1t2C2t=1t1+1t2Dt=√(t1t2)
The fig. shows the v−t graph of a particle moving in straight line. Find the time when particle returns to the starting point.
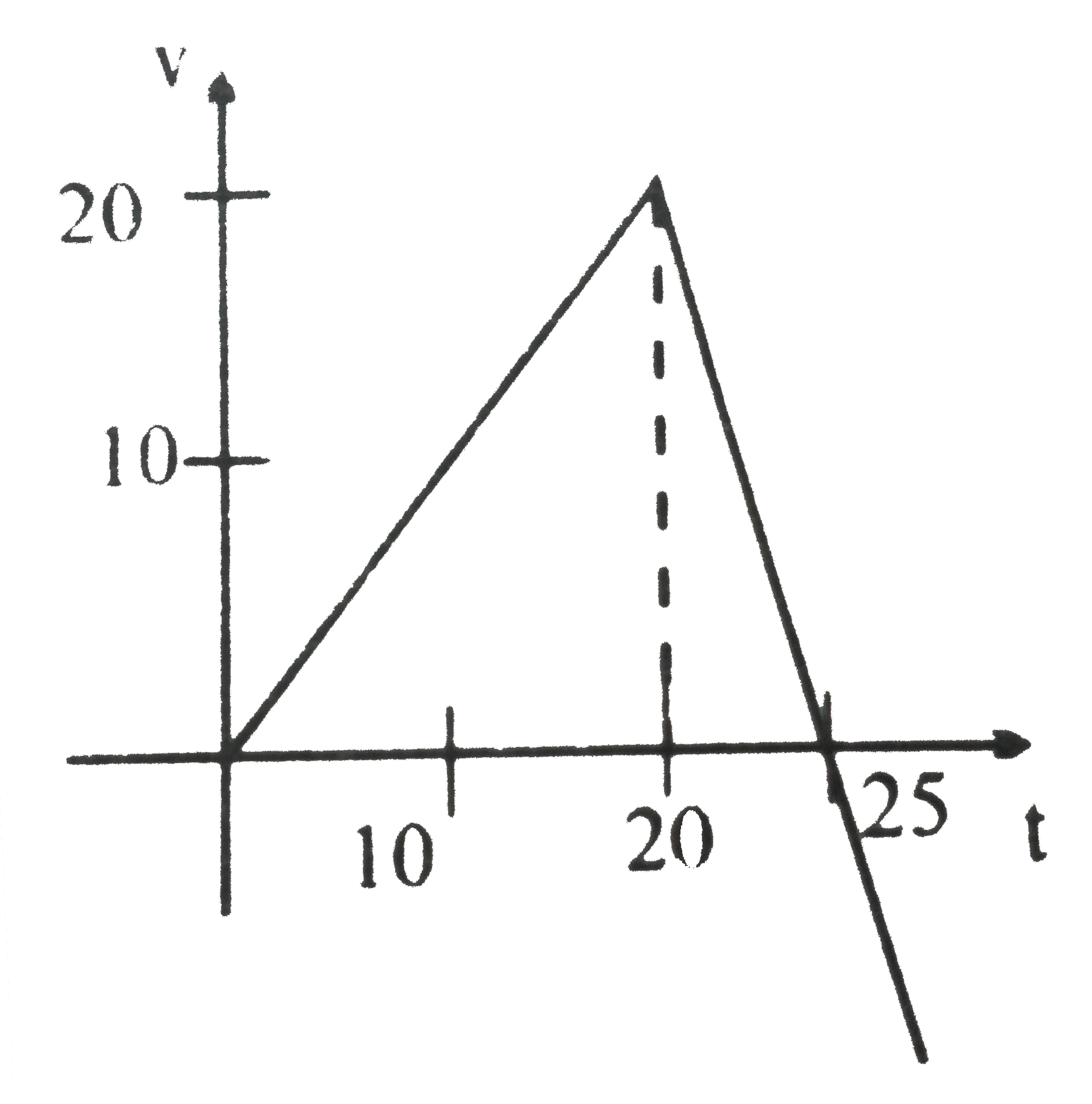
View SolutionIn an imaginary atmosphere, the air exerts a small force F on any particle in the direction of the particle's motion. A particle of mass m projected upward takes time t1 and reaching the maximum height and t2 in the return journey to the original point. Then
View SolutionW1,W2 and W3 are the different sizes of windows 1,2, and 3 respectively placed in a vertical plane. A particle is thrown up in the vertical plane. Let t1,t2 and t3 are the time taken to cross the window W1,W2 and W3 respectively and ΔV1,ΔV2 and ΔV3 are the change in speed after respective window cross.
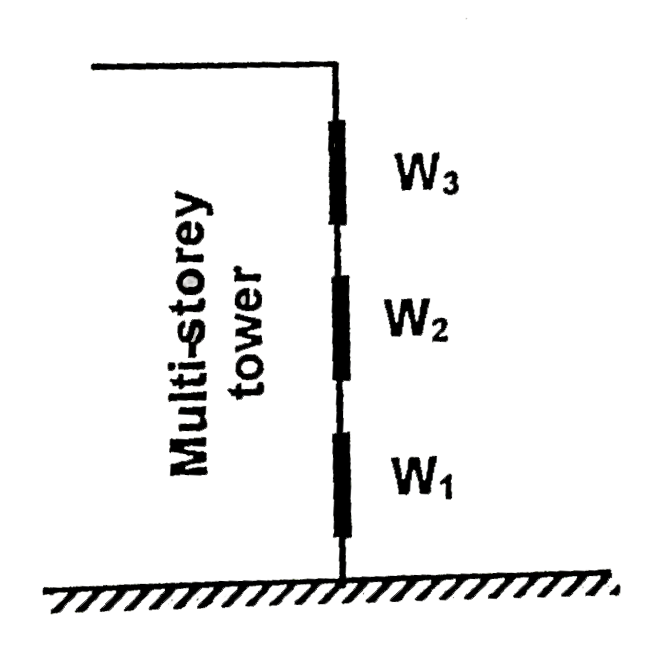
View SolutionA ball is thrown vertically upwards from the ground. If T1 and T2 are the respective time taken in going up and coming down, and the air resistance is not ignored, then
View SolutionA particle moves vertically with an upwards initial speed v0=10.5ms−1. If its acceleration varies with time as shown in a-t graph in figure, find the velocity of the particles at t = 4s.
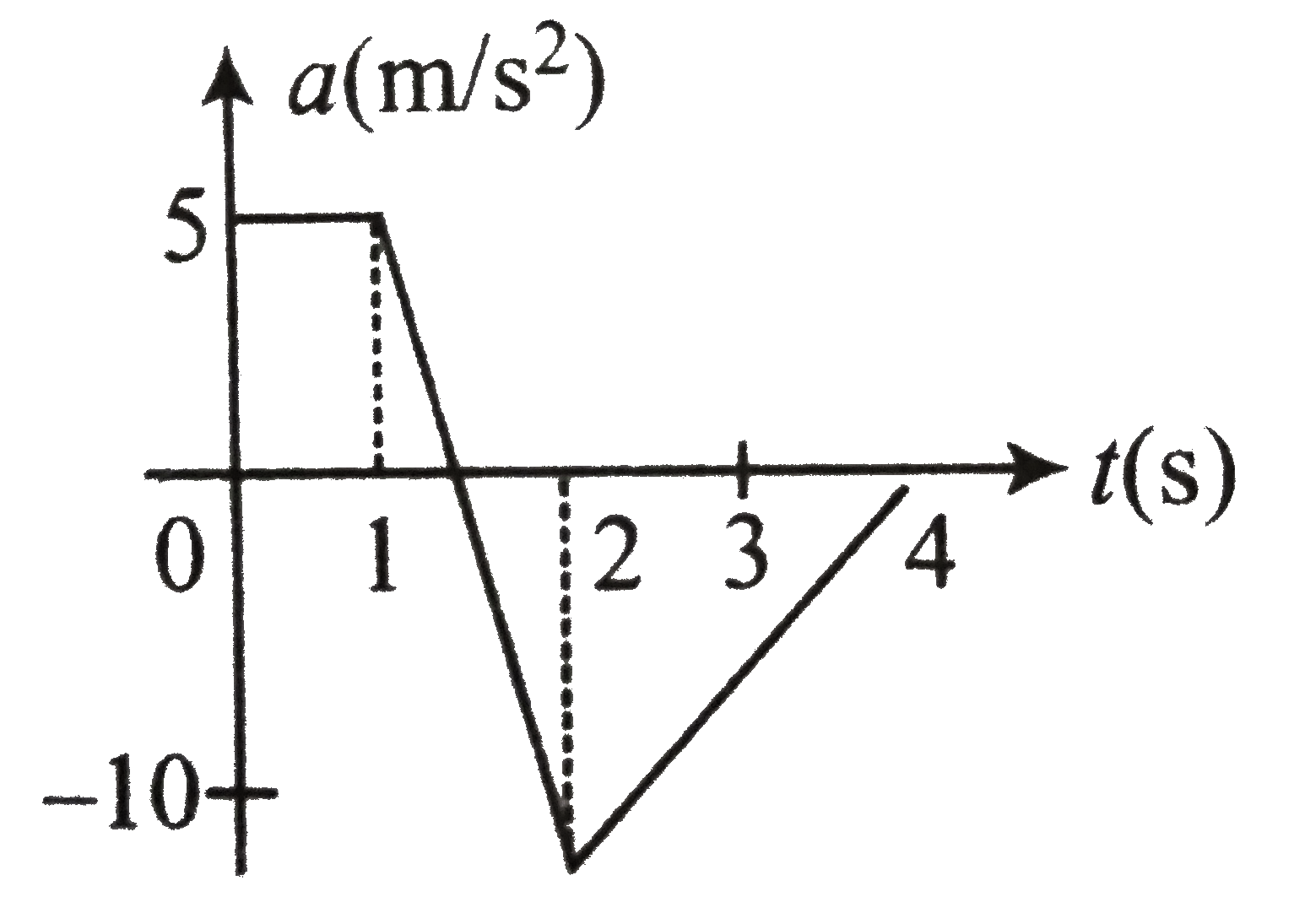
View SolutionA particle is projected horizontally with a speed v0 at t = 0 from a certain point above the ground . What is the magnitude of tangential acceleration of the particle at t=t1 is ( Assume that the particle does not strike the ground between the time interval t = 0 to t=t1 )
View SolutionA particle travels first half of the total time with speed v1 and second half time with speed v2. Find the average speed during the complete journey.
View Solution
