A variable line through the point (65,65) cuts the coordinates axes in the point AandB. If the point P divides AB internally in the ratio 2:1, show that the equation to the locus of P is :
xy=2x+y
5xy=2x+y
5xy=2(2x+y)
5xy=2(x+2y)
The correct Answer is:C
To find the locus of the point P that divides the line segment AB in the ratio 2:1, we can follow these steps:
Step 1: Define the variable line
Let the variable line that passes through the point (65,65) be represented in intercept form as:
xA+yB=1
where A and B are the x-intercept and y-intercept of the line, respectively.
Step 2: Identify the coordinates of points A and B
From the intercept form, the coordinates of point A (where the line intersects the x-axis) are (A,0) and the coordinates of point B (where the line intersects the y-axis) are (0,B).
Step 3: Use the section formula to find the coordinates of point P
Let the coordinates of point P be (H,K). Since P divides AB in the ratio 2:1, we can use the section formula:
H=2⋅0+1⋅A2+1=A3
K=2⋅B+1⋅02+1=2B3
Step 4: Express A and B in terms of H and K
From the equations derived:
A=3H
B=3K2
Step 5: Substitute A and B into the line equation
Since the line passes through the point (65,65), we can substitute A and B into the line equation:
6/5A+6/5B=1
Substituting A and B:
6/53H+6/53K2=1
This simplifies to:
615H+1215K=1
Multiplying through by 15 gives:
6K+12H=15HK
Step 6: Rearranging the equation
Rearranging the equation gives:
15HK−6K−12H=0
Factoring out common terms:
3(5HK−2K−4H)=0
Thus, we have:
5HK−2K−4H=0
Step 7: Final equation for the locus
To express this in terms of x and y (where H=x and K=y):
5xy−2y−4x=0
This is the equation of the locus of point P.
Let the variable line that passes through the point (65,65) be represented in intercept form as:
xA+yB=1
where A and B are the x-intercept and y-intercept of the line, respectively.
From the intercept form, the coordinates of point A (where the line intersects the x-axis) are (A,0) and the coordinates of point B (where the line intersects the y-axis) are (0,B).
Let the coordinates of point P be (H,K). Since P divides AB in the ratio 2:1, we can use the section formula:
H=2⋅0+1⋅A2+1=A3
K=2⋅B+1⋅02+1=2B3
From the equations derived:
A=3H
B=3K2
Since the line passes through the point (65,65), we can substitute A and B into the line equation:
6/5A+6/5B=1
Substituting A and B:
6/53H+6/53K2=1
This simplifies to:
615H+1215K=1
Multiplying through by 15 gives:
6K+12H=15HK
Rearranging the equation gives:
15HK−6K−12H=0
Factoring out common terms:
3(5HK−2K−4H)=0
Thus, we have:
5HK−2K−4H=0
To express this in terms of x and y (where H=x and K=y):
5xy−2y−4x=0
This is the equation of the locus of point P.
Topper's Solved these Questions
- STRAIGHT LINESBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - ILLUSTRATION 18
1 Videos
- STRAIGHT LINESBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - Section I - Solved Mcqs
64 Videos
- SETSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - SETSEXERCISE - Chapter Test
30 Videos
Similar Questions
A variable line through the point (65,65) cuts the coordinate axes at the points A and B respectively. If the point P divides AB internally in the ratio 2:1, then the equation of the locus of P is
View SolutionA(2,3) is a fixed point and Q(3cosθ,2sinθ) a variable point. If P divides AQ internally in the ratio 3:1, find the locus of P.
View SolutionA straight line passes through the points P(-1, 4) and Q(5,-2). It intersects the co-ordinate axes at points A and B. M is the midpoint of the segment AB. Find :
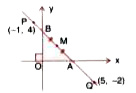
The equation of the line.
View SolutionFind the equation of straight line which passes through the point P(2,6) and cuts the coordinate axis at the point A and B respectively so that AP:BP=2:3.
View SolutionA straight line passes through the points A(-5, 2) and B(3,6). It intersects the co-ordinate axes at points C and D . M is a point on AB which divides CD in the ratio 1: 2. Find :
the co-ordinates of point M.
View SolutionA straight line passes through the points A(-5, 2) and B(3,6). It intersects the co-ordinate axes at points C and D . M is a point on AB which divides CD in the ratio 1: 2. Find :
the co-ordinates of points C and D.
View SolutionA straight line passes through the points A(-5, 2) and B(3,-6). It intersects the co-ordinate axes at points C and D . Find :
the equation of AB.
View SolutionA variable line through the point P(2,1) meets the axes at A an d B . Find the locus of the centroid of triangle OAB (where O is the origin).
View SolutionWrite the coordinates of the point dividing line segment joining points (2, 3) and (3, 4) internally in the ratio 1 :5 .
View SolutionFind the coordinates of a point which divides internally the points (1,3,7),(6,3,2) in the ratio 2:3.
View Solution
