PQRS is a parallclogram such that PQ is parallel to SR and SP is parallel to RQ. The length of side PQ is 20 cm. M is point between P and Q such that the length of PM is 3 cm. N is a points between points S and R. Find the length of SN such that segmen MN divides the paralelogram in two regions with equal areas.
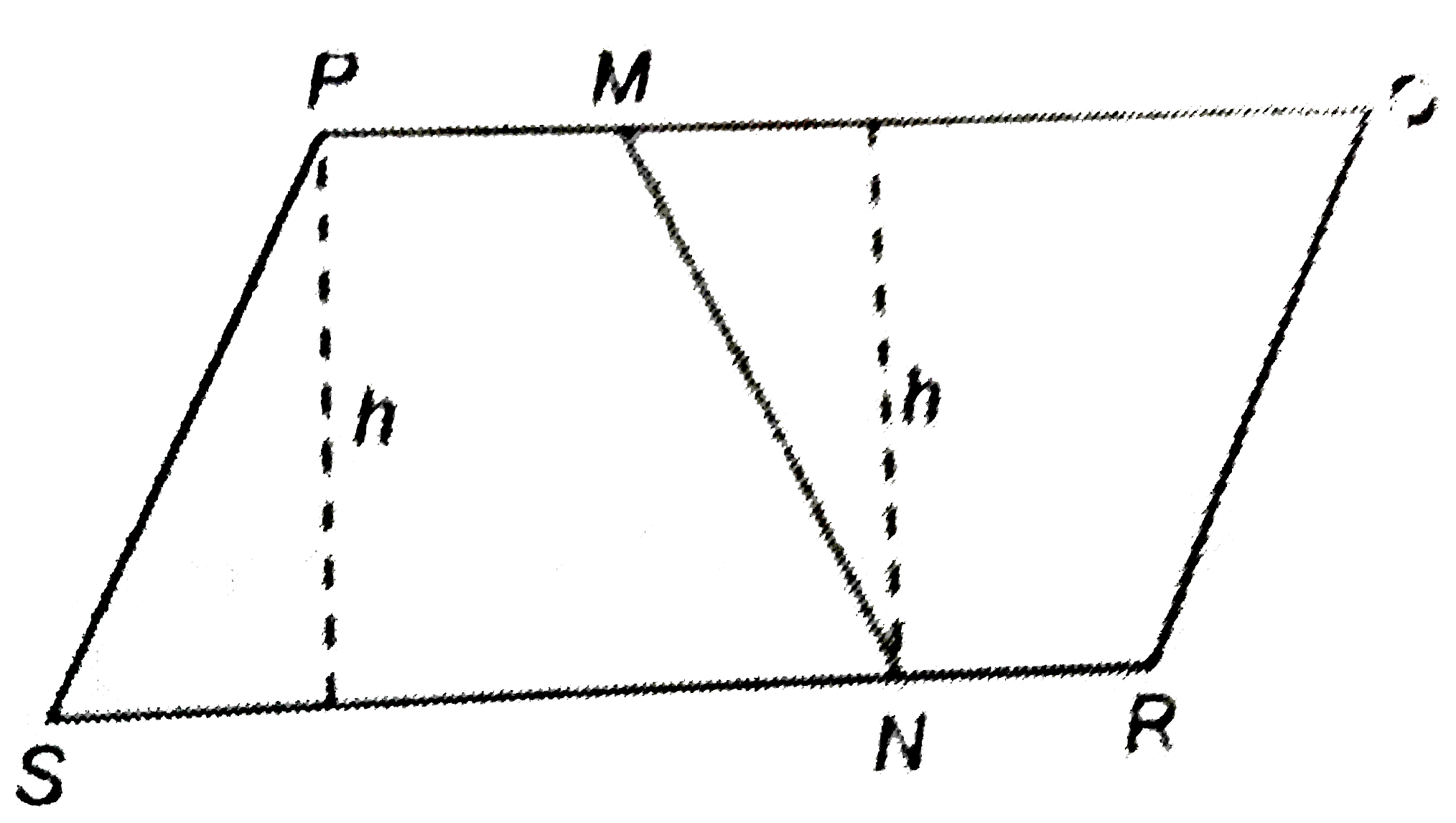
Let h be the perpendicular distance between PQ and SR.
Since PQ = 20 cm and PM = 3 cm
∴MQ=20−3=17cm
Also, SR=PQ=20cm (becauseopposite sides of a parallelogram are equal)
Now, ar(Trap.PMNS)=ar(Trip.MQRN)(given)
⇒ 12×h(PM+SN)=12×h(MQ+NR)
⇒ 3+SN=17+(SR−SN)
⇒ 2.SN=17+20−3=34
= SN=17cm.
Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - QUADRILATERALSEXERCISE - Problems From NCERT/exemplar11videos
QUADRILATERALS
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - QUADRILATERALSEXERCISE - Exercise 8a29videos
QUADRILATERALS
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - PROBABILITYEXERCISE - Revision Exercise (very Short Answer /short Answer Questions)10videos
PROBABILITY
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - STATISTICSEXERCISE - Revision Exercise12videos
STATISTICS
Similar Questions
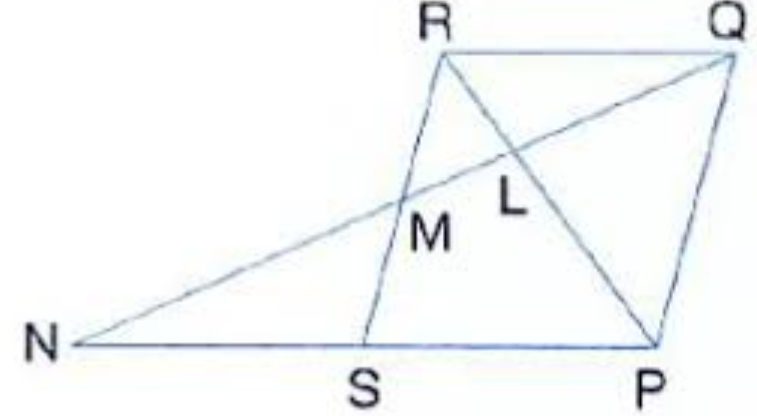
In the figure, PQRS is a parallelogram with PQ = 16 cm and QR = 10 cm. L is a point on PR such that RL:LP=2:3. QL produced meets RS at M and PS produced at N.
Find the lengths of PN and RM.
 View Solution
View SolutionABCD is a square with each side 12 cm. P is a point on BC such that area of ΔABP : area of trapezium APCD = 1:5. Find the length of CP.
View SolutionPQ is a chord of length 8 cm of a circle of radius 5 cm. The tangents at P and Q intersect at a point T . Find the length TP .
View SolutionPQR is a triangle. S is a point on the side QR of ΔPQR such that ∠PSR=∠QPR. Given QP = 8 cm, PR = 6 cm and SR = 3 cm.

Find the lengths of QR and PS.View SolutionTwo parallel chords are drawn in a circle of diameter 30.0 cm. The length of one chord is 24.0 cm and the distance between the two chords is 21.0 cm, find the length of the other chord.
View SolutionA tangent PQ at a point P of a circle of radius 5 cm meets a line through the centre O at a point Q so that OQ=13cm . Find the length of PQ .
View SolutionIn parallelogram PQRS. L is mid-point of side SR and SN is drawn parallel to LQ which meets RQ produced prove that :
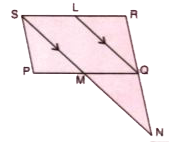
SP=12RNView SolutionGiven point (2,-3) and point (-5,4), find the length of ¯¯¯¯¯¯PQ and the coordinates of the midpoint, M.
View SolutionThe area of a trapezium is 475 cm2 and the height is 19 cm. Find the lengths of its two parallel sides, if one side is 4 cm greater than the other.
View SolutionA tangent PQ at a point P of a circle of radius 5 cm meets a line through the centre O at a point Q so that OQ=12cm. Length PQ is :
(A) 12 cm (B) 13 cm (C) 8.5 cm (D) √119cm.View Solution
