Question

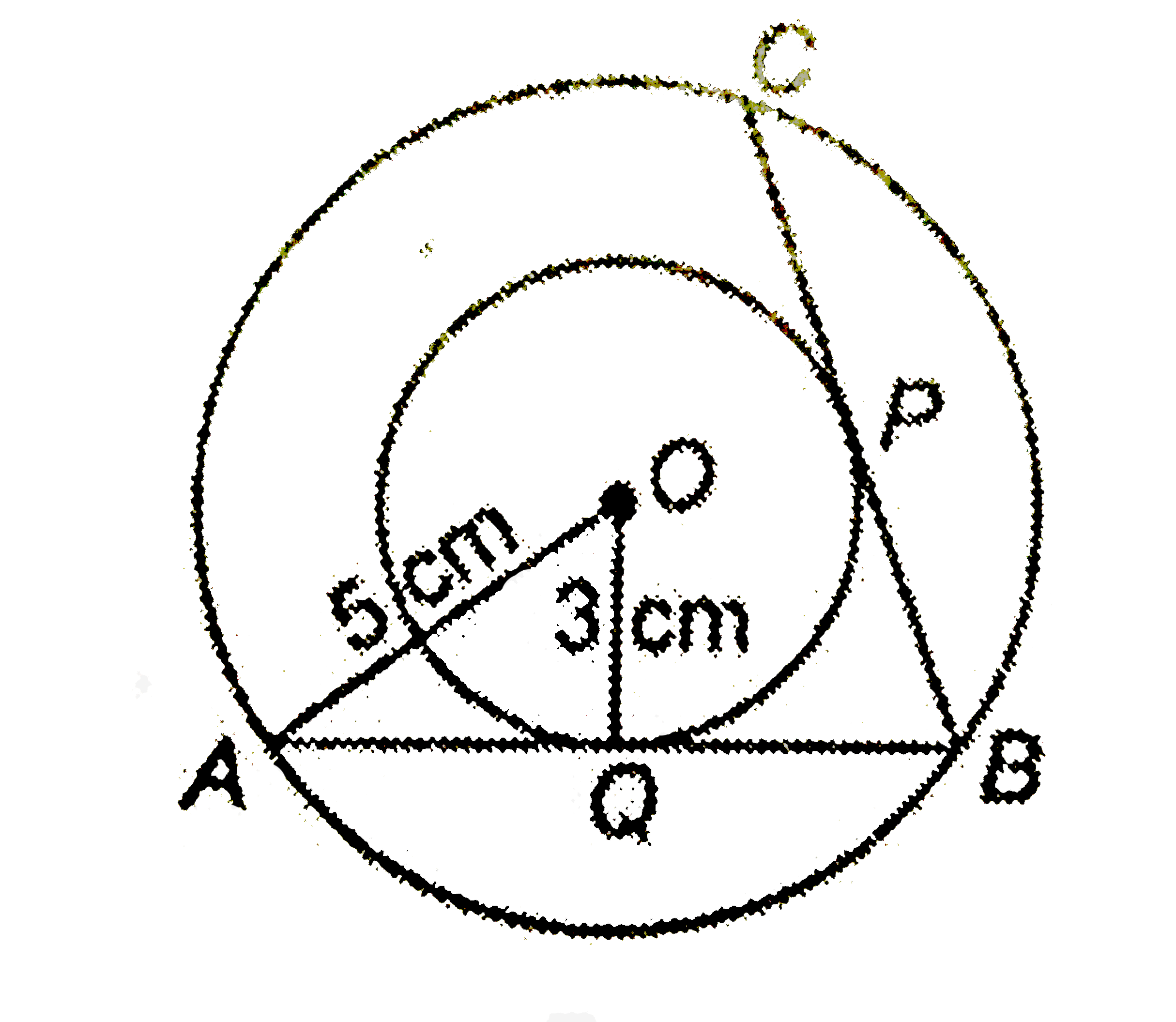
Two concentric circles are of radii 5 cm. and 3 c. Find the length of the chord of the larger circle which touches the cmaller circle.

Answer

8cm

Explanation

To find the length of the chord of the larger circle that touches the smaller circle, we can follow these steps:

Step 1: Understand the Problem
We have two concentric circles with the same center. The radius of the larger circle (R) is 5 cm, and the radius of the smaller circle (r) is 3 cm. We need to find the length of the chord of the larger circle that touches the smaller circle.

Hint: Draw a diagram of the two circles with the center and the chord.

Step 2: Draw the Diagram
Let the center of the circles be point C. Draw the larger circle with radius 5 cm and the smaller circle with radius 3 cm. Let the chord of the larger circle that touches the smaller circle be AB.

Hint: Label the points clearly and ensure that the chord is horizontal for simplicity.

Step 3: Identify the Right Triangle
Since the chord AB touches the smaller circle, the radius of the smaller circle (3 cm) drawn to the point of tangency (let's call it D) will be perpendicular to the chord AB. Therefore, we can form a right triangle ACD, where:
- AC is the radius of the larger circle (5 cm),
- CD is the radius of the smaller circle (3 cm),
- AD is half the length of the chord AB.

Hint: Remember that in a right triangle, the square of the hypotenuse equals the sum of the squares of the other two sides.

Step 4: Apply the Pythagorean Theorem
Using the Pythagorean theorem in triangle ACD:
\( AC^2 = AD^2 + CD^2 \)
Substituting the known values:
\( 5^2 = AD^2 + 3^2 \)
\( 25 = AD^2 + 9 \)

Hint: Isolate AD^2 to find its value.

Step 5: Solve for AD
Rearranging the equation gives:
\( AD^2 = 25 - 9 \)
\( AD^2 = 16 \)
Taking the square root of both sides:
\( AD = 4 \text{ cm} \)

Hint: Remember that AD is half the length of the chord AB.

Step 6: Find the Length of the Chord AB
Since AD is half of AB, we can find the full length of the chord:
\( AB = 2 \times AD = 2 \times 4 = 8 \text{ cm} \)

Hint: Double-check your calculations to ensure accuracy.

Final Answer
The length of the chord of the larger circle that touches the smaller circle is 8 cm.