Two concentric circles are of diameters 30cm and 18cm. Find the length of the chord of the larger circle which touches the smaller circle.
To solve the problem, we will follow these steps:
Step 1: Find the radii of the circles.
- The diameter of the larger circle is 30 cm, so its radius R1 is:
R1=302=15 cm
- The diameter of the smaller circle is 18 cm, so its radius R2 is:
R2=182=9 cm
Step 2: Understand the geometry of the problem.
- We have two concentric circles (they share the same center).
- A chord of the larger circle touches the smaller circle. This means that the distance from the center of the circles to the chord is equal to the radius of the smaller circle.
Step 3: Set up the right triangle.
- Let O be the center of the circles, M be the midpoint of the chord AB, and A and B be the endpoints of the chord.
- The distance OM (from the center to the chord) is equal to the radius of the smaller circle, which is 9 cm.
- The distance OA (from the center to point A) is equal to the radius of the larger circle, which is 15 cm.
Step 4: Apply the Pythagorean theorem.
- In triangle OMA, we have:
OA2=OM2+AM2
- Substituting the known values:
152=92+AM2
225=81+AM2
AM2=225−81=144
AM=√144=12 cm
Step 5: Calculate the length of the chord.
- The length of the chord AB is twice the length of AM:
AB=2×AM=2×12=24 cm
Final Answer:
The length of the chord of the larger circle which touches the smaller circle is 24 cm.
---
- The diameter of the larger circle is 30 cm, so its radius R1 is:
R1=302=15 cm
- The diameter of the smaller circle is 18 cm, so its radius R2 is:
R2=182=9 cm
- We have two concentric circles (they share the same center).
- A chord of the larger circle touches the smaller circle. This means that the distance from the center of the circles to the chord is equal to the radius of the smaller circle.
- Let O be the center of the circles, M be the midpoint of the chord AB, and A and B be the endpoints of the chord.
- The distance OM (from the center to the chord) is equal to the radius of the smaller circle, which is 9 cm.
- The distance OA (from the center to point A) is equal to the radius of the larger circle, which is 15 cm.
- In triangle OMA, we have:
OA2=OM2+AM2
- Substituting the known values:
152=92+AM2
225=81+AM2
AM2=225−81=144
AM=√144=12 cm
- The length of the chord AB is twice the length of AM:
AB=2×AM=2×12=24 cm
The length of the chord of the larger circle which touches the smaller circle is 24 cm.
Topper's Solved these Questions
Similar Questions
Two concentric circles are of radii 5 cm and 3 cm. Find the length of the chord of the larger circle which touches the smaller circle.
View SolutionTwo concentric circles are of radii 5 cm and 3 cm. Find the length of the chord of the larger circle which touches the smaller circle.
View SolutionTwo concentric circles are of radii 5 cm and 3 cm. Find the length of the chord of the larger circle which touches the smaller circle.
View SolutionTwo concentric circles are of radii 5 cm. and 3 c. Find the length of the chord of the larger circle which touches the cmaller circle.
View SolutionTwo concentric circles are of radii 13 cm and 5 cm. Find the length of the chord of the larger circle which touches the inner circle.
View SolutionIf the radii of two concentric circles are 4 cm and 5 cm, then find the length of each chord of one circle which is tangent to the other circle.
View SolutionTwo concentric circle of radii 3cm and 5cm are given. Find the chord BC which touches the inner circle at P.
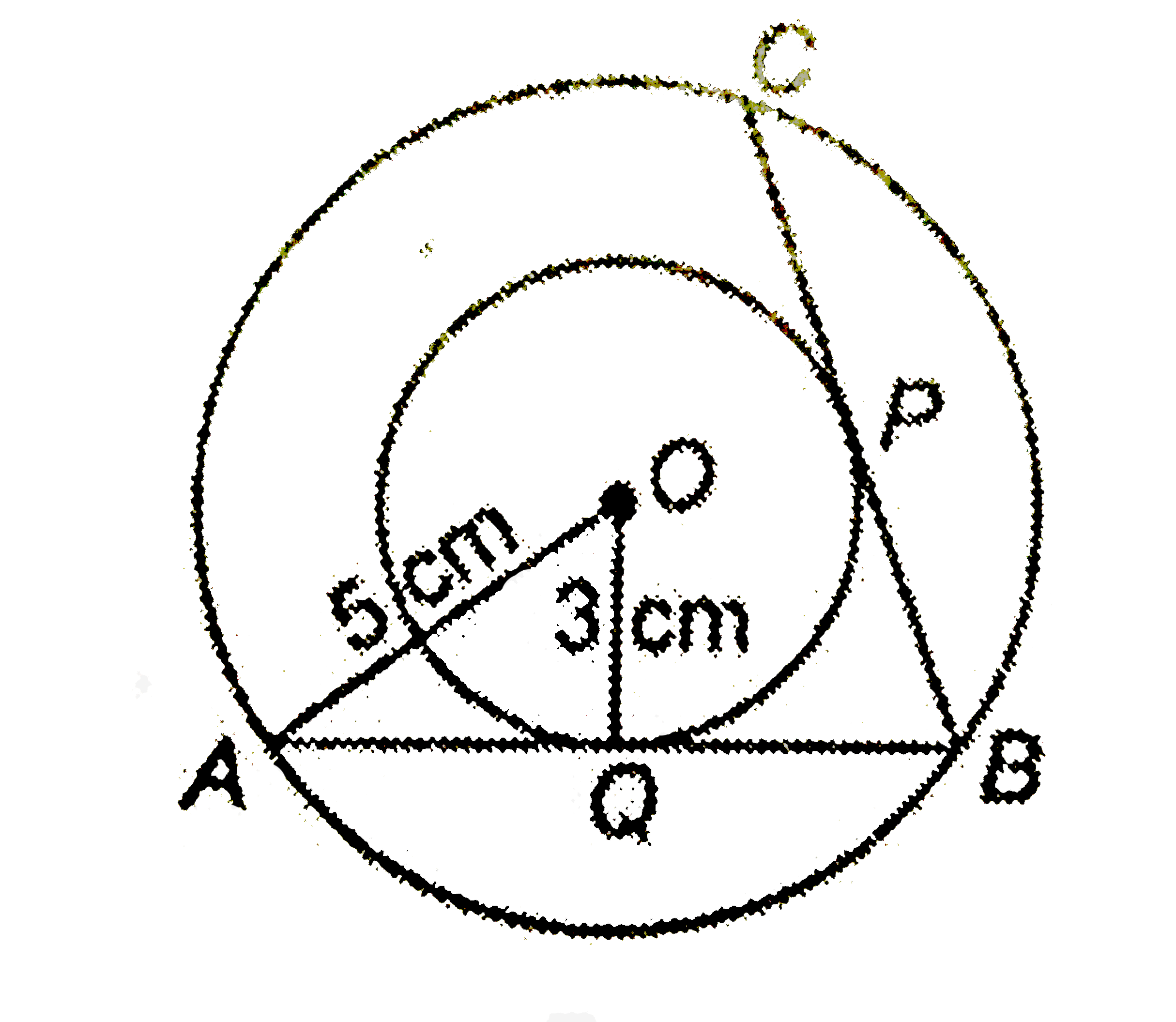
View SolutionIf radii of two concentric circles are 4 cm and 5 cm, then length of each chord of one circle which is tangent to the other circle, is
View SolutionIf radii of the two concentric circles are 15cm and 17cm, then the length of each chord of one circle which is tangent to other is: 8cm (b) 16cm (c) 30cm (d) 17cm
View SolutionIf radii of the two concentric circles are 15cm and 17cm, then the length of each chord of one circle which is tangent to other is: 8cm (b) 16cm (c) 30cm (d) 17cm
View Solution