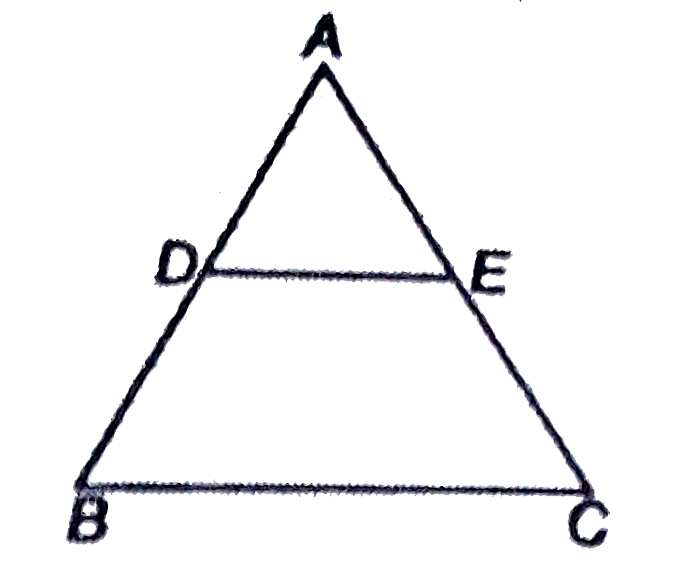
In the given figure, in ΔABC,DE∣∣BC so that AD= (4x - 3) cm, AE= ( 8x-7) cm, BD= (3x-1) cm and CE= (5x-3) cm . Find the value of x.
To solve the problem, we will use Thales' theorem, which states that if a line is drawn parallel to one side of a triangle, it divides the other two sides proportionally.
Given:
- AD = (4x - 3) cm
- AE = (8x - 7) cm
- BD = (3x - 1) cm
- CE = (5x - 3) cm
Since DE || BC, we can apply Thales' theorem:
ADBD=AECE
Substituting the given values into the equation:
4x−33x−1=8x−75x−3
Next, we will cross-multiply to eliminate the fractions:
(4x−3)(5x−3)=(8x−7)(3x−1)
Now, we will expand both sides of the equation:
Left side:
(4x−3)(5x−3)=20x2−12x−15
Right side:
(8x−7)(3x−1)=24x2−8x−21
Now we set the two expanded expressions equal to each other:
20x2−12x−15=24x2−8x−21
Next, we will move all terms to one side of the equation to set it to zero:
20x2−12x−15−24x2+8x+21=0
Combining like terms:
−4x2−4x+6=0
To simplify, we can multiply the entire equation by -1:
4x2+4x−6=0
Now, we can divide the entire equation by 2 to simplify further:
2x2+2x−3=0
Next, we will use the quadratic formula to find the values of x, where a=2,b=2,c=−3:
x=−b±√b2−4ac2a
Calculating the discriminant:
b2−4ac=22−4(2)(−3)=4+24=28
Now substituting back into the quadratic formula:
x=−2±√284
Simplifying √28:
√28=2√7
So,
x=−2±2√74=−1±√72
Thus, the possible values of x are:
x=−1+√72orx=−1−√72
Since x must be a positive length, we take:
x=−1+√72
- AD = (4x - 3) cm
- AE = (8x - 7) cm
- BD = (3x - 1) cm
- CE = (5x - 3) cm
(4x−3)(5x−3)=20x2−12x−15
(8x−7)(3x−1)=24x2−8x−21
Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLES EXERCISE - Problems From NCERT/ Exemplar14videos
TRIANGLES
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLES EXERCISE - Exercise 6a24videos
TRIANGLES
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - STATISTICS EXERCISE - Revision Exercise Long Answer Questions4videos
STATISTICS
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - VOLUME AND SURFACE AREA OF SOLIDSEXERCISE - Revisions Exercise Long Answer Questions5videos
VOLUME AND SURFACE AREA OF SOLIDS
Similar Questions
In the given figure, ΔABC is similar to ΔDEF,AB=(x−0.5) cm, AC=1.5xcm,DE=9cm,andDF=3xcm. Find the lengths of AB and DF.
 View Solution
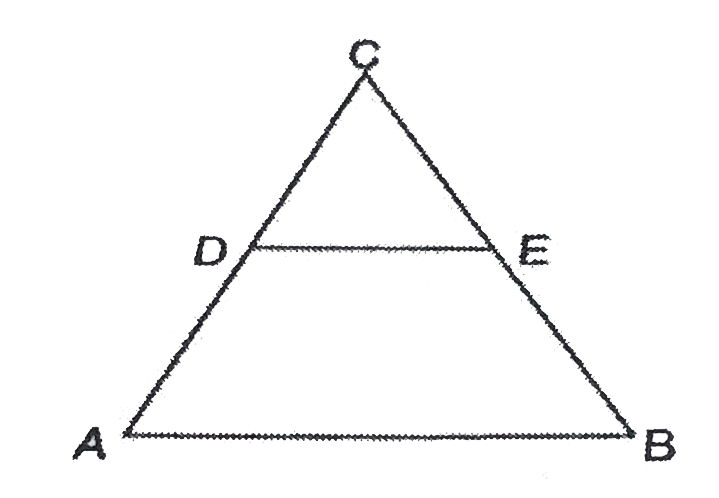
View SolutionIn the given figure if DE||BC, find the value of x.
View SolutionIn the figure if DE||AB, find the value of x . Given AD= (x-4).
DC= 4cm, EB= (3x-19) cm and EC= (x-3) cm.
 View Solution
View SolutionThe perimeter of a parallelogram ABCD = 40 cm , AB = 3x cm, BC = 2x cm and CD = 2( y+1) cm . Find the values of x and y.
View SolutionIn the given figure, DE||BC and ADDB=23if AE=3.7 cm , find EC.
 View Solution
View SolutionIn the following figure, ∠ABC=90∘ AB = (x+8) cm , BC = (x+ 1) cm and AC = (x+15) cm.
Find the lengths of the sides of the triangles.
 View Solution
View SolutionIn parallelogram ABCD , AB = (3x- 4) cm, BC = ( y- 1) cm , CD = ( y+ 5) cm and AD = (2x+ 5) cm. find the ratio AB : BC.
Find the values of x and y.View SolutionIn ΔABC, angle A=90∘, side AB = x cm, AC = (x+5) cm and area = 150 cm2. Find the sides of triangle.
View SolutionIt is given that ΔABC~ΔEDF such that AB=5 cm, AC=7 cm, DF= 15 cm and DE = 12 cm. Find the lengths of the remaining sides of the triangles.
View SolutionIn ΔABC , ∠A is right - angled . If AB= 1 cm , AC =3 cm and BC = √10 cm , then find the values of cos B and sin C.
View Solution

