A bullet of mass 0.02 kg travelling horizontally with velocity 250ms−1 strikes a block of wood of mass 0.23 kg which rests on a rough horizontal surface. After the impact, the block and bullet move together and come to rest after travelling a distance of 40m. The coefficient of sliding friction of the rough surface is (g=9.8ms_2)
(a)0.75
(b)0.61
(c)0.51
(d)0.30
To solve the problem step by step, we will follow these steps:
Step 1: Understand the Problem
We have a bullet of mass m1=0.02kg traveling with a velocity u1=250m/s that strikes a block of wood of mass m2=0.23kg at rest. After the collision, the bullet and block move together and come to rest after traveling a distance of d=40m.
Step 2: Apply Conservation of Momentum
Before the collision, the momentum of the system is given by:
Initial Momentum=m1u1+m2u2
Since the block is at rest, u2=0:
Initial Momentum=(0.02kg⋅250m/s)+(0.23kg⋅0)=5kg m/s
After the collision, the bullet and block move together with a common velocity v. The total mass after the collision is m1+m2=0.02+0.23=0.25kg.
Using conservation of momentum:
m1u1=(m1+m2)v
Substituting the values:
5=0.25v
Solving for v:
v=50.25=20m/s
Step 3: Apply Work-Energy Principle
The work done by friction is equal to the change in kinetic energy. The initial kinetic energy of the block and bullet system after the collision is:
KE=12(m1+m2)v2=12(0.25)(20)2=12(0.25)(400)=50J
The work done by friction when the block comes to rest over a distance d=40m is given by:
W=μ(m1+m2)gd
Setting the work done equal to the initial kinetic energy:
μ(0.25)(9.8)(40)=50
Step 4: Solve for Coefficient of Friction μ
Rearranging the equation:
μ=50(0.25)(9.8)(40)
Calculating the denominator:
(0.25)(9.8)(40)=98
Thus:
μ=5098≈0.51
Final Answer
The coefficient of sliding friction μ is approximately 0.51.
---
We have a bullet of mass m1=0.02kg traveling with a velocity u1=250m/s that strikes a block of wood of mass m2=0.23kg at rest. After the collision, the bullet and block move together and come to rest after traveling a distance of d=40m.
Before the collision, the momentum of the system is given by:
Initial Momentum=m1u1+m2u2
Since the block is at rest, u2=0:
Initial Momentum=(0.02kg⋅250m/s)+(0.23kg⋅0)=5kg m/s
m1u1=(m1+m2)v
Substituting the values:
5=0.25v
Solving for v:
v=50.25=20m/s
The work done by friction is equal to the change in kinetic energy. The initial kinetic energy of the block and bullet system after the collision is:
KE=12(m1+m2)v2=12(0.25)(20)2=12(0.25)(400)=50J
W=μ(m1+m2)gd
Setting the work done equal to the initial kinetic energy:
μ(0.25)(9.8)(40)=50
Rearranging the equation:
μ=50(0.25)(9.8)(40)
(0.25)(9.8)(40)=98
Thus:
μ=5098≈0.51
The coefficient of sliding friction μ is approximately 0.51.
Similar Questions
A body is moving along a rough horizontal surface with an initial velocity 6m/s m If the body comes to rest after travelling 9 m , then the coefficient of sliding friction will be
View SolutionA 30kg block rests on a rough horizontal surface A force of 200N is applied on the block The block acquires a speed of 4m/s starting from rest in 2s What is the value of coefficient of friction ? .
View Solution
Knowledge Check
A block slides with a velocity of 10 m/sec. on a rough horizontal surface. It comes to rest after covering of 50 m. If g is 10 m/sec2, then the coefficient of dynamic friction between the block and surface is
A1B10C2D0.1
A ball rolled on ice with a velocity of 14 ms−1 comes to rest after travelling 40 m . Find the coefficient of friction . (Given g=9.8m/s2
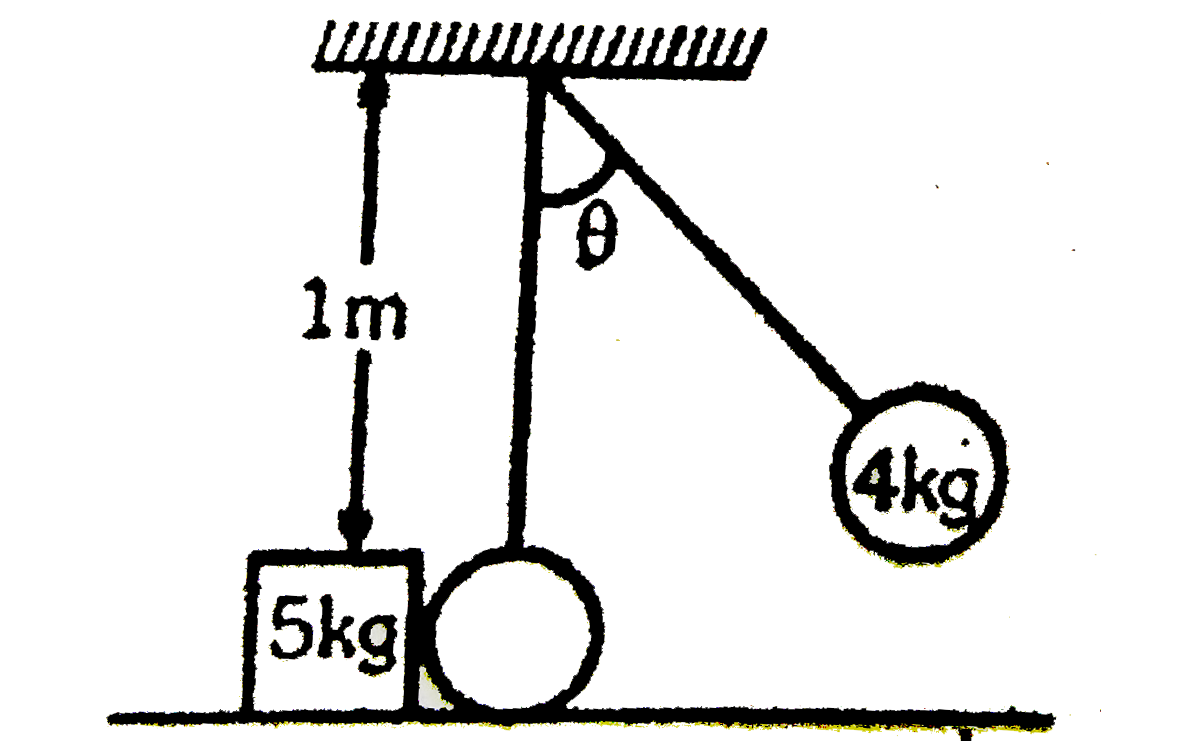
View SolutionThe 4kg sphere form rest when θ=60∘ strikes a block mass of 5kg placed on a rough horizontal plane and comes to rest after collision. The 5kg block comes to rest after moving a distance of 0.8m. Find μ of ground & block & coefficient of restituation e.
View SolutionA block moving on a horizontal surface with velocity 20ms−1 comes to rest because of surface friction over a distance of 40 m. Taking g=10ms−2 , the coefficient of dynamic friction is :
View SolutionA bullet of mass 10g travelling horizontally with a velocity of 150ms−1 strikes a stationary wooden block and come to rest in 0.03s. Calculate the distance of penetration of the bullet into the block. Also, Calculate the magnitude of the force exerted by the wooden block on the bullet,
View SolutionA bullet of mass 20 g travelling horizontally with a speed of 500 m/s passes through a wooden block of mass 10.0 kg initially at rest on a level surface. The bulet emerges with speed of 100 m/s and the block slides 20 cm on the surface before coming to rest. Find the friction cioefficient between the block and the surface figure.

View SolutionA bullet of mass 0.01kg and travelling at a speed of 500ms−1 strikes a block of mass 2kg which is suspended by a string of length 5m. The centre of gravity of the block is found to raise a vertical distance of 0.2m. What is the speed of the bullet after it emerges from the block?
View SolutionA bullet of mass 0.012 kg and horizontal speed 70ms−1 strikes a block of wood of mass 0.4 kg and instantly comes to rest with respect to the block. The block is suspended from the ceiling by thin wire. Calculate the height to which the block rises. Also, estimate the amount of heat produced in the block.
View Solution
