A uniform rope of length l lies on a table . If the coefficient of friction is μ , then the maximum length l1 of the part of this rope which can overhang from the edge of the table without sliding down is :
1μ
lμ+1
μl1+μ
μl1−μ
1μ
lμ+1
μl1+μ
μl1−μ
The correct Answer is:C
To solve the problem of determining the maximum length l1 of the part of the rope that can overhang from the edge of the table without sliding down, we can follow these steps:
Step 1: Define the Variables
Let:
- l = total length of the rope
- l1 = length of the rope hanging off the table
- μ = coefficient of friction
- λ = linear mass density of the rope (mass per unit length)
Step 2: Determine the Weight of the Hanging Part
The weight of the hanging part of the rope can be expressed as:
Whanging=λl1g
where g is the acceleration due to gravity.
Step 3: Determine the Weight of the Part on the Table
The length of the rope lying on the table is l−l1. Therefore, the weight of the part of the rope lying on the table is:
Wtable=λ(l−l1)g
Step 4: Calculate the Friction Force
The frictional force Ffriction that prevents the rope from sliding is given by:
Ffriction=μ⋅N
where N is the normal force. The normal force is equal to the weight of the part of the rope lying on the table:
N=Wtable=λ(l−l1)g
Thus, the frictional force can be expressed as:
Ffriction=μ⋅λ(l−l1)g
Step 5: Set Up the Equation for Equilibrium
For the rope to not slide down, the frictional force must equal the weight of the hanging part:
λl1g=μ⋅λ(l−l1)g
Step 6: Simplify the Equation
We can cancel g and λ from both sides (assuming λ≠0):
l1=μ(l−l1)
Step 7: Solve for l1
Rearranging the equation gives:
l1+μl1=μl
l1(1+μ)=μl
l1=μl1+μ
Conclusion
The maximum length l1 of the part of the rope that can overhang without sliding down is:
l1=μl1+μ
Final Answer
Thus, the correct option is:
μl1+μ
Let:
- l = total length of the rope
- l1 = length of the rope hanging off the table
- μ = coefficient of friction
- λ = linear mass density of the rope (mass per unit length)
The weight of the hanging part of the rope can be expressed as:
Whanging=λl1g
where g is the acceleration due to gravity.
The length of the rope lying on the table is l−l1. Therefore, the weight of the part of the rope lying on the table is:
Wtable=λ(l−l1)g
The frictional force Ffriction that prevents the rope from sliding is given by:
Ffriction=μ⋅N
where N is the normal force. The normal force is equal to the weight of the part of the rope lying on the table:
N=Wtable=λ(l−l1)g
Thus, the frictional force can be expressed as:
Ffriction=μ⋅λ(l−l1)g
For the rope to not slide down, the frictional force must equal the weight of the hanging part:
λl1g=μ⋅λ(l−l1)g
We can cancel g and λ from both sides (assuming λ≠0):
l1=μ(l−l1)
Rearranging the equation gives:
l1+μl1=μl
l1(1+μ)=μl
l1=μl1+μ
The maximum length l1 of the part of the rope that can overhang without sliding down is:
l1=μl1+μ
Thus, the correct option is:
μl1+μ
Similar Questions
Assuming the length of a chain to be L and coefficient of static friction μ. Compute the maximum length of the chain which can be held outside a table without sliding.
View SolutionA homogoneous chain of length L lies on a table. The coefficient of friction between the chain and the table is μ. The maximum length which can hang over the table in equilibrium is
View Solution
Knowledge Check
A block of mass m is at rest on an inclined plane. The coefficient of static friction is μ. The maximum angle of incline before the block begins to slide down is :
Atan−1(μ/m)Btan−1(μ)Ctan−1(μ/2)Dcos−1(μ)A chain of uniform mass m and length L is held on a frictionless table in such a way that its 1nth part is hanging below the edge of table. The work done to pull the hanging part of chain is :−
A√nBnCn−3Dn−2
A uniform rope so lies on a table that part of it lays over. The rope begins to slide when the length of hanging part is 25% of entire length. The co-efficient of friction between rope and table is
View SolutionA car has to move on a level turn of radius 45 m. If the coefficient of static friction between the tyre and the road is μs=2.0, find the maximum speed the car can take without skidding.
View SolutionA uniform chain of mass m and length I is kept on the table with a part of it overhanging (see figure). If the coefficient of friction between the table and the chain is 1/3 then find the maximum length of the chain that can overhang such that the chain remain in equilibrium.
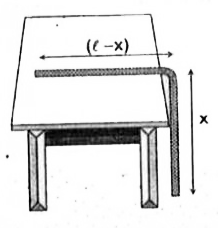
View SolutionA uniform chain of length L lies on a smooth horizontal table with its length perpendicular to the edge of the table and a small portion of the chain is hanging over the edge. The chain starts sliding due to the weight of the hanging part
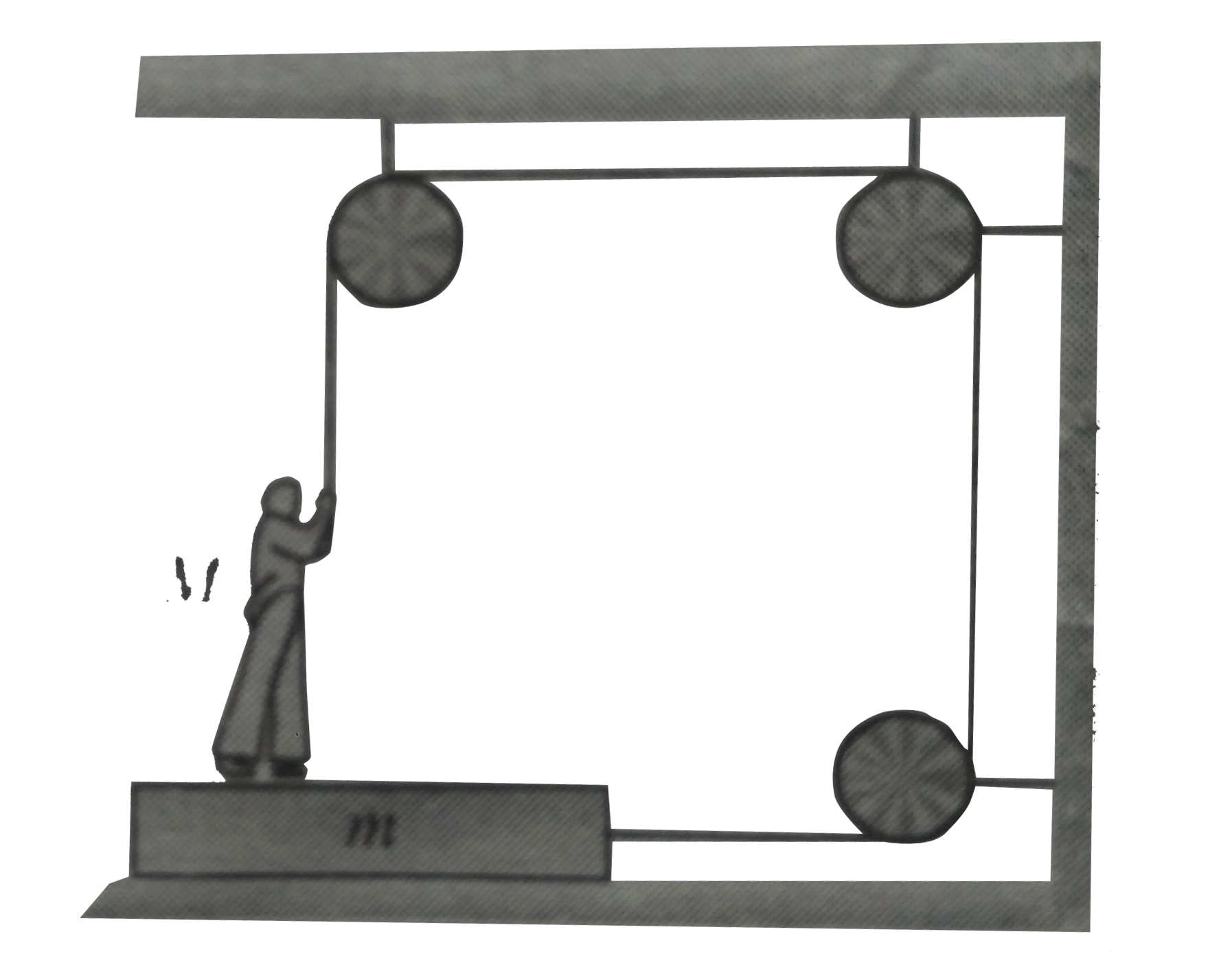
View SolutionThe friction coefficient between the board and the floor shown in figure is μ Find the maximum force that the man can exert on the rope so that the board does not slip on the floor
View SolutionA rope lies on a table such that a part of it hangs down the table, when the length of hanging part is 1/3 of entire length the rope just begins to slide. The coefficient of friction between the rope and the table is:-
View Solution
