A battery of 10V is connected to a resistance of 20Ω through a variable resistance R. If the variable resistance R is increased at the rate of 5Ω min−1, then the amount of charge that passes through the battery in 4 min is
120 C
120loge(2)C
120loge(2)C
60loge(2)C
The correct Answer is:B
To solve the problem step by step, we will follow these steps:
Step 1: Understand the Circuit Configuration
We have a battery of 10V connected to a fixed resistor of 20Ω and a variable resistor R. The variable resistance R is increasing at a rate of 5Ω/min.
Step 2: Define the Variable Resistance
Let the variable resistance R at time t (in minutes) be given by:
R(t)=5tΩ
where t is the time in minutes.
Step 3: Calculate the Total Resistance
The total resistance Rtotal in the circuit is the sum of the fixed resistance and the variable resistance:
Rtotal=20Ω+R(t)=20Ω+5tΩ=20+5tΩ
Step 4: Calculate the Current in the Circuit
Using Ohm's Law, the current I in the circuit can be calculated as:
I=VRtotal=10V20+5t
Step 5: Relate Current to Charge
The charge dq that passes through the battery in a small time dt can be expressed as:
dq=Idt=1020+5tdt
Step 6: Integrate to Find Total Charge
To find the total charge Q that passes through the battery in 4 minutes, we need to integrate dq from t=0 to t=4:
Q=∫401020+5tdt
Step 7: Solve the Integral
First, simplify the integral:
Q=10∫40120+5tdt
Let u=20+5t, then du=5dt or dt=du5. Changing the limits:
- When t=0, u=20
- When t=4, u=20+20=40
Now, substitute into the integral:
Q=10∫40201u⋅du5=2∫40201udu
Q=2(lnu)4020=2(ln40−ln20)=2ln(4020)=2ln2
Step 8: Convert to SI Units
Since we calculated the charge in terms of minutes, we need to convert it to seconds. The total time is 4min=240s:
Q=2ln2×60s
Final Calculation
Calculating Q:
Q=120ln2C
Conclusion
The amount of charge that passes through the battery in 4 minutes is 120ln2C.
---
We have a battery of 10V connected to a fixed resistor of 20Ω and a variable resistor R. The variable resistance R is increasing at a rate of 5Ω/min.
Let the variable resistance R at time t (in minutes) be given by:
R(t)=5tΩ
where t is the time in minutes.
The total resistance Rtotal in the circuit is the sum of the fixed resistance and the variable resistance:
Rtotal=20Ω+R(t)=20Ω+5tΩ=20+5tΩ
Using Ohm's Law, the current I in the circuit can be calculated as:
I=VRtotal=10V20+5t
The charge dq that passes through the battery in a small time dt can be expressed as:
dq=Idt=1020+5tdt
To find the total charge Q that passes through the battery in 4 minutes, we need to integrate dq from t=0 to t=4:
Q=∫401020+5tdt
First, simplify the integral:
Q=10∫40120+5tdt
Let u=20+5t, then du=5dt or dt=du5. Changing the limits:
- When t=0, u=20
- When t=4, u=20+20=40
Q=10∫40201u⋅du5=2∫40201udu
Q=2(lnu)4020=2(ln40−ln20)=2ln(4020)=2ln2
Since we calculated the charge in terms of minutes, we need to convert it to seconds. The total time is 4min=240s:
Q=2ln2×60s
Calculating Q:
Q=120ln2C
The amount of charge that passes through the battery in 4 minutes is 120ln2C.
Similar Questions
A battery of emf 10 V is connected with a load resistance of 50 Omega the total power delivered by the battery is 2 W. If load resistance is replaced by R then power delivered is 4 W, the value of R is
View SolutionA 24 V battery of internal resistance 4.0Ω is connected to a variable resistance. At what value of the current drawn from the battery is the rate of heat produced in the resistor maximum?
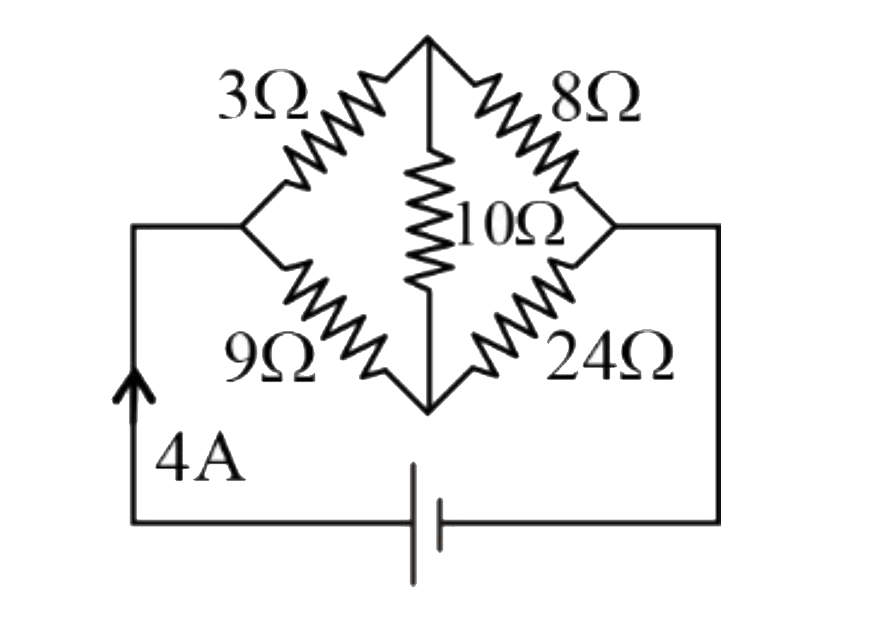
View SolutionIn the circuit shown, if the 10Ω resistance is replaced by 20Ω Then the amount of current draw from the battery will be
View SolutionA 50V battery is connected across a 10 ohm resistor. The current is 4.5A is flowing through the resistor then the internal resistance of the battery is
View SolutionA potentiometer wire of length 10m and resistance 30Ω is connected in series with a battery of emf 2.5V, internal resistance 5Ω and an external resistance R. If the fall of potential along the potentiometer wire is 50μVmm−1, then the value of R is found to be 23nΩ. What is n?
View SolutionA battery of e.m.f. 10V and internal resistance 0.5ohm is connected across a variable resistance R. The value of R for which the power delivered in it is maximum is given by
View SolutionA battery of ε and internal resistance r is used in a circuit with a variable external resistance R. Find the value of R for which the power consumed in R is maximum.
View SolutionWhen a battery connected across a resistor of 16Ω, the voltage across the resistor is 12 V. When the same battery is connected across a resistor of 10Ω, voltage across it is 11 V. The internal resistance of the battery (in ohm) is
View SolutionA battery of emf 2V and internal resistance 0.5(Ω)is connected across a resistance 1.5(Ω). Find the current flow through battery ?
View SolutionA battery of EMF E produces currents 4 A and 3 A when connected to external resistance 1Ω and 2Ω respectively. The internal resistance of the battery is
View Solution
