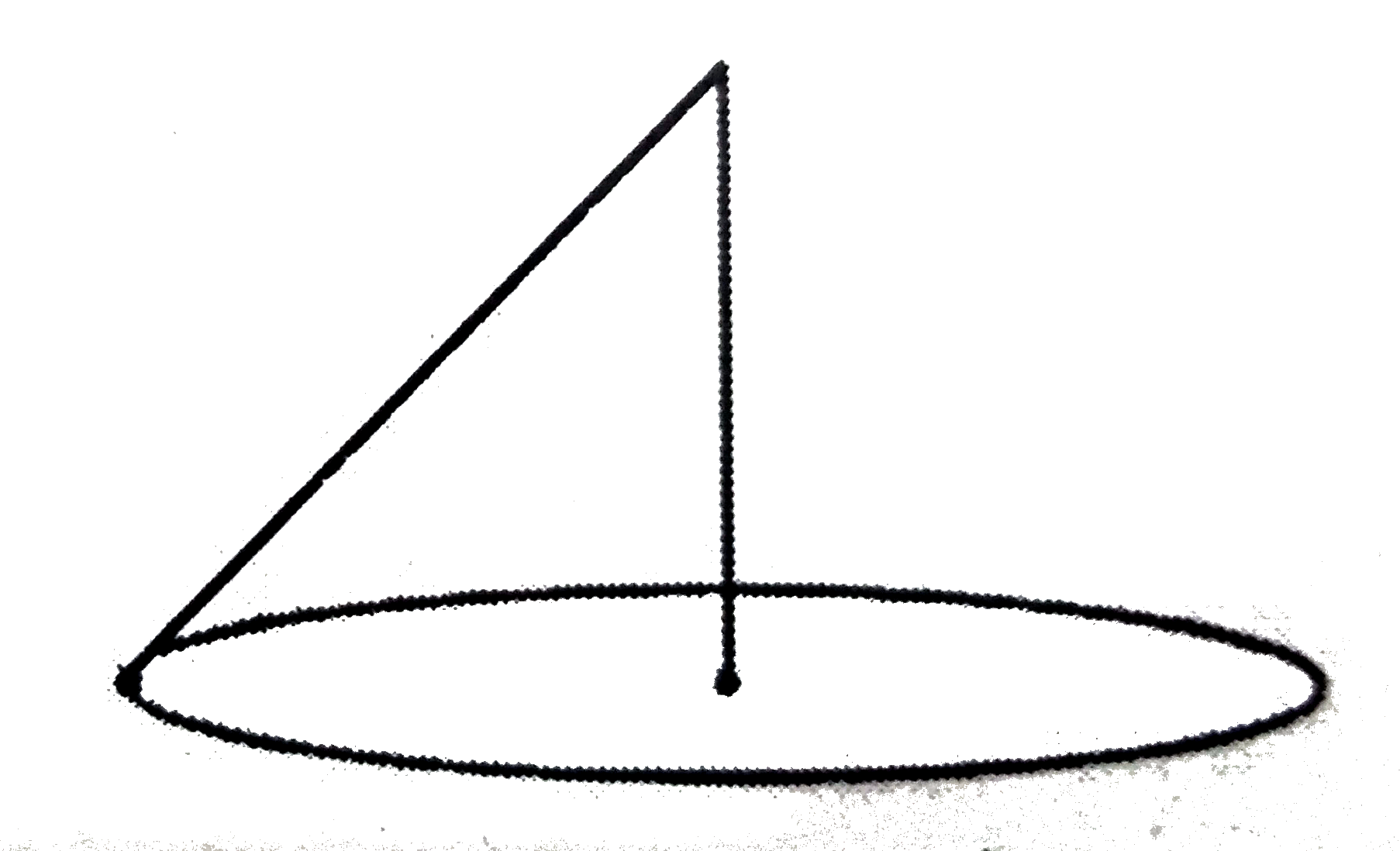
A particle of mass m is suspended from a ceiling through a string of length L. The particle moves in a horizontal circle of radius r. Find a. the speed of the particle and b. the tension in the string. Such a system is called a conical pendulum.
v=2r√g(L2−r2)1/2andT=3mgL(L2−r2)1/2
v=r√g(L2−r2)1/4andT=mgL(L3−r3)1/3
v=3r√g(L2−r2)1/2andT=mgL(L2−r2)1/2
v=r√g(L2−r2)1/4andT=mgL(L2−r2)1/2
The correct Answer is:D
To solve the problem of a particle of mass m suspended from a ceiling through a string of length L moving in a horizontal circle of radius r, we will follow these steps:
Step 1: Understand the Geometry of the Problem
The particle moves in a horizontal circle, forming a cone shape with the string. The angle between the string and the vertical is θ. The vertical component of the tension T balances the weight of the particle, and the horizontal component provides the necessary centripetal force for circular motion.
Step 2: Identify the Forces Acting on the Particle
1. The weight of the particle acting downwards: mg
2. The tension in the string T, which can be resolved into two components:
- Vertical component: Tcosθ
- Horizontal component: Tsinθ
Step 3: Set Up the Equations
From the vertical equilibrium (forces in the vertical direction):
Tcosθ=mg(1)
From the horizontal motion (providing centripetal force):
Tsinθ=mv2r(2)
Step 4: Relate the Radius and the Length of the String
Using the geometry of the conical pendulum, we can relate r, L, and θ:
Lcosθ=h(height from the ceiling to the particle)
Lsinθ=r
From these, we can express cosθ and sinθ:
sinθ=rLandcosθ=√1−(rL)2=√L2−r2L
Step 5: Substitute into the Equations
Substituting sinθ and cosθ into equations (1) and (2):
From equation (1):
T⋅√L2−r2L=mg⇒T=mgL√L2−r2(3)
From equation (2):
T⋅rL=mv2r⇒T=mv2Lr2(4)
Step 6: Equate the Two Expressions for Tension
Setting equations (3) and (4) equal to each other:
mgL√L2−r2=mv2Lr2
Cancelling m and L (assuming L≠0):
g√L2−r2=v2r2
Rearranging gives:
v2=gr2√L2−r2⇒v=r√g√L2−r2
Step 7: Solve for Tension
Substituting v2 back into equation (4):
T=m⋅gr2L2−r2⋅Lr2=mgL√L2−r2
Final Answers
a. The speed of the particle v is:
v=r√g√L2−r2
b. The tension in the string T is:
T=mgL√L2−r2
The particle moves in a horizontal circle, forming a cone shape with the string. The angle between the string and the vertical is θ. The vertical component of the tension T balances the weight of the particle, and the horizontal component provides the necessary centripetal force for circular motion.
1. The weight of the particle acting downwards: mg
2. The tension in the string T, which can be resolved into two components:
- Vertical component: Tcosθ
- Horizontal component: Tsinθ
From the vertical equilibrium (forces in the vertical direction):
Tcosθ=mg(1)
From the horizontal motion (providing centripetal force):
Tsinθ=mv2r(2)
Using the geometry of the conical pendulum, we can relate r, L, and θ:
Lcosθ=h(height from the ceiling to the particle)
Lsinθ=r
From these, we can express cosθ and sinθ:
sinθ=rLandcosθ=√1−(rL)2=√L2−r2L
Substituting sinθ and cosθ into equations (1) and (2):
From equation (1):
T⋅√L2−r2L=mg⇒T=mgL√L2−r2(3)
T⋅rL=mv2r⇒T=mv2Lr2(4)
Setting equations (3) and (4) equal to each other:
mgL√L2−r2=mv2Lr2
Cancelling m and L (assuming L≠0):
g√L2−r2=v2r2
Rearranging gives:
v2=gr2√L2−r2⇒v=r√g√L2−r2
Substituting v2 back into equation (4):
T=m⋅gr2L2−r2⋅Lr2=mgL√L2−r2
a. The speed of the particle v is:
v=r√g√L2−r2
T=mgL√L2−r2
Similar Questions
A particle of mass 'm' is suspended from the ceiling through a string of length L. The particle moves in a horizontal circle of radius r. The speed of the particle is
View SolutionA particle of mass 'm' is suspended from the ceiling through a string of length L. The particle moves in a horizontal circle of radius r. The speed of the particle is
View SolutionA particle of mass 'm' is suspended from a ceiling through a string of length 'L'. If the particle moves in a horizontal circle of radius 'r' as shown in the figure, then the speed of the particle is

View SolutionA particle of mass 'm' is suspended from a ceiling through a string of length'L'. If the particle moves in a horizontal circle of radius 'r', then the speed of the particle is
View SolutionA particle of mass m is doing horizontal circular motion with the help of a string (conical pendulum) as shown in the figure. If speed of the particle is constant then,
View SolutionA particle of mass m is tied to a string of length L and whirled into a horizontal plan. If tension in the string is T then the speed of the particle will be :
View SolutionConsider a conical pendulum having bob is mass m is suspended from a ceiling through a string of length L. The bob moves in a horizontal circle of radius r. Find (a) the angular speed of the bob and (b) the tension in the string.
View Solution